Cantellated 5-simplexes

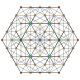
In five-dimensional geometry, a cantellated 5-simplex is a convex uniform 5-polytope, being a cantellation of the regular 5-simplex.

5-simplex	Cantellated 5-simplex	Bicantellated 5-simplex
Birectified 5-simplex	Cantitruncated 5-simplex	Bicantitruncated 5-simplex
Orthogonal projections in A5 Coxeter plane

There are unique 4 degrees of cantellation for the 5-simplex, including truncations.

Cantellated 5-simplex

Cantellated 5-simplex
Type	Uniform 5-polytope
Schläfli symbol	rr{3,3,3,3} = ${\displaystyle r\left\{{\begin{array}{l}3,3,3\\3\end{array}}\right\}}$
Coxeter-Dynkin diagram	or
4-faces	27	6 r{3,3,3} 6 rr{3,3,3} 15 {}x{3,3}
Cells	135	30 {3,3} 30 r{3,3} 15 rr{3,3} 60 {}x{3}
Faces	290	200 {3} 90 {4}
Edges	240
Vertices	60
Vertex figure	Tetrahedral prism
Coxeter group	A5 [3,3,3,3], order 720
Properties	convex

The cantellated 5-simplex has 60 vertices, 240 edges, 290 faces (200 triangles and 90 squares), 135 cells (30 tetrahedra, 30 octahedra, 15 cuboctahedra and 60 triangular prisms), and 27 4-faces (6 cantellated 5-cell, 6 rectified 5-cells, and 15 tetrahedral prisms).

Alternate names

• Cantellated hexateron
• Small rhombated hexateron (Acronym: sarx) (Jonathan Bowers)^[1]

Coordinates

The vertices of the cantellated 5-simplex can be most simply constructed on a hyperplane in 6-space as permutations of (0,0,0,1,1,2) or of (0,1,1,2,2,2). These represent positive orthant facets of the cantellated hexacross and bicantellated hexeract respectively.

Images

orthographic projections

Ak Coxeter plane	A5	A4
Graph
Dihedral symmetry	[6]	[5]
Ak Coxeter plane	A3	A2
Graph
Dihedral symmetry	[4]	[3]

Bicantellated 5-simplex

Bicantellated 5-simplex
Type	Uniform 5-polytope
Schläfli symbol	2rr{3,3,3,3} = ${\displaystyle r\left\{{\begin{array}{l}3,3\\3,3\end{array}}\right\}}$
Coxeter-Dynkin diagram	or
4-faces	32	12 t02{3,3,3} 20 {3}x{3}
Cells	180	30 t1{3,3} 120 {}x{3} 30 t02{3,3}
Faces	420	240 {3} 180 {4}
Edges	360
Vertices	90
Vertex figure
Coxeter group	A5×2, [[3,3,3,3]], order 1440
Properties	convex, isogonal

Alternate names

• Bicantellated hexateron
• Small birhombated dodecateron (Acronym: sibrid) (Jonathan Bowers)^[2]

Coordinates

The coordinates can be made in 6-space, as 90 permutations of:

(0,0,1,1,2,2)

This construction exists as one of 64 orthant facets of the bicantellated 6-orthoplex.

Images

orthographic projections

Ak Coxeter plane	A5	A4
Graph
Dihedral symmetry	[6]	[[5]]=[10]
Ak Coxeter plane	A3	A2
Graph
Dihedral symmetry	[4]	[[3]]=[6]

Cantitruncated 5-simplex

cantitruncated 5-simplex
Type	Uniform 5-polytope
Schläfli symbol	tr{3,3,3,3} = ${\displaystyle t\left\{{\begin{array}{l}3,3,3\\3\end{array}}\right\}}$
Coxeter-Dynkin diagram	or
4-faces	27	6 t012{3,3,3} 6 t{3,3,3} 15 {}x{3,3}
Cells	135	15 t012{3,3} 30 t{3,3} 60 {}x{3} 30 {3,3}
Faces	290	120 {3} 80 {6} 90 {}x{}
Edges	300
Vertices	120
Vertex figure	Irr. 5-cell
Coxeter group	A5 [3,3,3,3], order 720
Properties	convex

Alternate names

• Cantitruncated hexateron
• Great rhombated hexateron (Acronym: garx) (Jonathan Bowers)^[3]

Coordinates

The vertices of the cantitruncated 5-simplex can be most simply constructed on a hyperplane in 6-space as permutations of (0,0,0,1,2,3) or of (0,1,2,3,3,3). These construction can be seen as facets of the cantitruncated 6-orthoplex or bicantitruncated 6-cube respectively.

Images

orthographic projections

Ak Coxeter plane	A5	A4
Graph
Dihedral symmetry	[6]	[5]
Ak Coxeter plane	A3	A2
Graph
Dihedral symmetry	[4]	[3]

Bicantitruncated 5-simplex

Bicantitruncated 5-simplex
Type	Uniform 5-polytope
Schläfli symbol	2tr{3,3,3,3} = ${\displaystyle t\left\{{\begin{array}{l}3,3\\3,3\end{array}}\right\}}$
Coxeter-Dynkin diagram	or
4-faces	32	12 tr{3,3,3} 20 {3}x{3}
Cells	180	30 t{3,3} 120 {}x{3} 30 t{3,4}
Faces	420	240 {3} 180 {4}
Edges	450
Vertices	180
Vertex figure
Coxeter group	A5×2, [[3,3,3,3]], order 1440
Properties	convex, isogonal

Alternate names

• Bicantitruncated hexateron
• Great birhombated dodecateron (Acronym: gibrid) (Jonathan Bowers)^[4]

Coordinates

The coordinates can be made in 6-space, as 180 permutations of:

(0,0,1,2,3,3)

This construction exists as one of 64 orthant facets of the bicantitruncated 6-orthoplex.

Images

orthographic projections

Ak Coxeter plane	A5	A4
Graph
Dihedral symmetry	[6]	[[5]]=[10]
Ak Coxeter plane	A3	A2
Graph
Dihedral symmetry	[4]	[[3]]=[6]

Related uniform 5-polytopes

The cantellated 5-simplex is one of 19 uniform 5-polytopes based on the [3,3,3,3] Coxeter group, all shown here in A₅ Coxeter plane orthographic projections. (Vertices are colored by projection overlap order, red, orange, yellow, green, cyan, blue, purple having progressively more vertices)

A5 polytopes
t0	t1	t2	t0,1	t0,2	t1,2	t0,3
t1,3	t0,4	t0,1,2	t0,1,3	t0,2,3	t1,2,3	t0,1,4
t0,2,4	t0,1,2,3	t0,1,2,4	t0,1,3,4	t0,1,2,3,4

Notes

1. Klitizing, (x3o3x3o3o - sarx)
2. Klitizing, (o3x3o3x3o - sibrid)
3. Klitizing, (x3x3x3o3o - garx)
4. Klitizing, (o3x3x3x3o - gibrid)

References

• H.S.M. Coxeter:
  • H.S.M. Coxeter, Regular Polytopes, 3rd Edition, Dover New York, 1973
  • Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6
    • (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10]
    • (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II, [Math. Zeit. 188 (1985) 559-591]
    • (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45]
• Norman Johnson Uniform Polytopes, Manuscript (1991)
  • N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D.
• Klitzing, Richard. "5D uniform polytopes (polytera)". x3o3x3o3o - sarx, o3x3o3x3o - sibrid, x3x3x3o3o - garx, o3x3x3x3o - gibrid

External links

• Glossary for hyperspace, George Olshevsky.
• Polytopes of Various Dimensions, Jonathan Bowers
• Multi-dimensional Glossary

v t e Fundamental convex regular and uniform polytopes in dimensions 2–10
Family	An	Bn	I2(p) / Dn	E6 / E7 / E8 / F4 / G2	Hn
Regular polygon	Triangle	Square	p-gon	Hexagon	Pentagon
Uniform polyhedron	Tetrahedron	Octahedron • Cube	Demicube		Dodecahedron • Icosahedron
Uniform polychoron	Pentachoron	16-cell • Tesseract	Demitesseract	24-cell	120-cell • 600-cell
Uniform 5-polytope	5-simplex	5-orthoplex • 5-cube	5-demicube
Uniform 6-polytope	6-simplex	6-orthoplex • 6-cube	6-demicube	122 • 221
Uniform 7-polytope	7-simplex	7-orthoplex • 7-cube	7-demicube	132 • 231 • 321
Uniform 8-polytope	8-simplex	8-orthoplex • 8-cube	8-demicube	142 • 241 • 421
Uniform 9-polytope	9-simplex	9-orthoplex • 9-cube	9-demicube
Uniform 10-polytope	10-simplex	10-orthoplex • 10-cube	10-demicube
Uniform n-polytope	n-simplex	n-orthoplex • n-cube	n-demicube	1k2 • 2k1 • k21	n-pentagonal polytope
Topics: Polytope families • Regular polytope • List of regular polytopes and compounds