8-cube

In geometry, an 8-cube is an eight-dimensional hypercube. It has 256 vertices, 1024 edges, 1792 square faces, 1792 cubic cells, 1120 tesseract 4-faces, 448 5-cube 5-faces, 112 6-cube 6-faces, and 16 7-cube 7-faces.

8-cube Octeract
Orthogonal projection inside Petrie polygon
Type	Regular 8-polytope
Family	hypercube
Schläfli symbol	{4,36}
Coxeter-Dynkin diagrams
7-faces	16 {4,35}
6-faces	112 {4,34}
5-faces	448 {4,33}
4-faces	1120 {4,32}
Cells	1792 {4,3}
Faces	1792 {4}
Edges	1024
Vertices	256
Vertex figure	7-simplex
Petrie polygon	hexadecagon
Coxeter group	C8, [36,4]
Dual	8-orthoplex
Properties	convex, Hanner polytope

It is represented by Schläfli symbol {4,3⁶}, being composed of 3 7-cubes around each 6-face. It is called an octeract, a portmanteau of tesseract (the 4-cube) and oct for eight (dimensions) in Greek. It can also be called a regular hexdeca-8-tope or hexadecazetton, being an 8-dimensional polytope constructed from 16 regular facets.

It is a part of an infinite family of polytopes, called hypercubes. The dual of an 8-cube can be called an 8-orthoplex and is a part of the infinite family of cross-polytopes.

Cartesian coordinates

Cartesian coordinates for the vertices of an 8-cube centered at the origin and edge length 2 are

(±1,±1,±1,±1,±1,±1,±1,±1)

while the interior of the same consists of all points (x₀, x₁, x₂, x₃, x₄, x₅, x₆, x₇) with -1 < x_i < 1.

As a configuration

This configuration matrix represents the 8-cube. The rows and columns correspond to vertices, edges, faces, cells, 4-faces, 5-faces, 6-faces, and 7-faces. The diagonal numbers say how many of each element occur in the whole 8-cube. The nondiagonal numbers say how many of the column's element occur in or at the row's element.^[1][2]

${\displaystyle {\begin{bmatrix}{\begin{matrix}256&8&28&56&70&56&28&8\\2&1024&7&21&35&35&21&7\\4&4&1792&6&15&20&15&6\\8&12&6&1792&5&10&10&5\\16&32&24&8&1120&4&6&4\\32&80&80&40&10&448&3&3\\64&192&240&160&60&12&112&2\\128&448&672&560&280&84&14&16\end{matrix}}\end{bmatrix}}}$

The diagonal f-vector numbers are derived through the Wythoff construction, dividing the full group order of a subgroup order by removing one mirror at a time.^[3]

B8		k-face	fk	f0	f1	f2	f3	f4	f5	f6	f7	k-figure	notes
A7		( )	f0	256	8	28	56	70	56	28	8	{3,3,3,3,3,3}	B8/A7 = 2^8*8!/8! = 256
A6A1		{ }	f1	2	1024	7	21	35	35	21	7	{3,3,3,3,3}	B8/A6A1 = 2^8*8!/7!/2 = 1024
A5B2		{4}	f2	4	4	1792	6	15	20	15	6	{3,3,3,3}	B8/A5B2 = 2^8*8!/6!/4/2 = 1792
A4B3		{4,3}	f3	8	12	6	1792	5	10	10	5	{3,3,3}	B8/A4B3 = 2^8*8!/5!/8/3! = 1792
A3B4		{4,3,3}	f4	16	32	24	8	1120	4	6	4	{3,3}	B8/A3B4 = 2^8*8!/4!/2^4/4! = 1120
A2B5		{4,3,3,3}	f5	32	80	80	40	10	448	3	3	{3}	B8/A2B5 = 2^8*8!/3!/2^5/5! = 448
A1B6		{4,3,3,3,3}	f6	64	192	240	160	60	12	112	2	{ }	B8/A1B6 = 2^8*8!/2/2^6/6!= 112
B7		{4,3,3,3,3,3}	f7	128	448	672	560	280	84	14	16	( )	B8/B7 = 2^8*8!/2^7/7! = 16

Projections

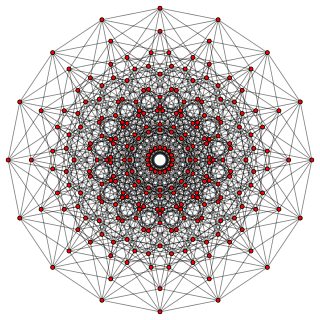
This 8-cube graph is an orthogonal projection. This orientation shows columns of vertices positioned a vertex-edge-vertex distance from one vertex on the left to one vertex on the right, and edges attaching adjacent columns of vertices. The number of vertices in each column represents rows in Pascal's triangle, being 1:8:28:56:70:56:28:8:1.

orthographic projections

B8			B7
[16]			[14]
B6			B5
[12]			[10]
B4		B3		B2
[8]		[6]		[4]
A7		A5		A3
[8]		[6]		[4]

Derived polytopes

Applying an alternation operation, deleting alternating vertices of the octeract, creates another uniform polytope, called a 8-demicube, (part of an infinite family called demihypercubes), which has 16 demihepteractic and 128 8-simplex facets.

Related polytopes

The 8-cube is 8th in an infinite series of hypercube:

Petrie polygon orthographic projections

Line segment	Square	Cube	4-cube	5-cube	6-cube	7-cube	8-cube	9-cube	10-cube

References

1. Coxeter, Regular Polytopes, sec 1.8 Configurations
2. Coxeter, Complex Regular Polytopes, p.117
3. Klitzing, Richard. "o3o3o3o3o3o3o4x - octo".

• H.S.M. Coxeter:
  • Coxeter, Regular Polytopes, (3rd edition, 1973), Dover edition, ISBN 0-486-61480-8, p. 296, Table I (iii): Regular Polytopes, three regular polytopes in n-dimensions (n≥5)
  • Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6
    • (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10]
    • (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II, [Math. Zeit. 188 (1985) 559-591]
    • (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45]
• Norman Johnson Uniform Polytopes, Manuscript (1991)
  • N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D. (1966)
• Klitzing, Richard. "8D uniform polytopes (polyzetta) o3o3o3o3o3o3o4x - octo".

External links

• Weisstein, Eric W. "Hypercube". MathWorld.
• Olshevsky, George. "Measure polytope". Glossary for Hyperspace. Archived from the original on 4 February 2007.
• Multi-dimensional Glossary: hypercube Garrett Jones

v t e Fundamental convex regular and uniform polytopes in dimensions 2–10
Family	An	Bn	I2(p) / Dn	E6 / E7 / E8 / F4 / G2	Hn
Regular polygon	Triangle	Square	p-gon	Hexagon	Pentagon
Uniform polyhedron	Tetrahedron	Octahedron • Cube	Demicube		Dodecahedron • Icosahedron
Uniform polychoron	Pentachoron	16-cell • Tesseract	Demitesseract	24-cell	120-cell • 600-cell
Uniform 5-polytope	5-simplex	5-orthoplex • 5-cube	5-demicube
Uniform 6-polytope	6-simplex	6-orthoplex • 6-cube	6-demicube	122 • 221
Uniform 7-polytope	7-simplex	7-orthoplex • 7-cube	7-demicube	132 • 231 • 321
Uniform 8-polytope	8-simplex	8-orthoplex • 8-cube	8-demicube	142 • 241 • 421
Uniform 9-polytope	9-simplex	9-orthoplex • 9-cube	9-demicube
Uniform 10-polytope	10-simplex	10-orthoplex • 10-cube	10-demicube
Uniform n-polytope	n-simplex	n-orthoplex • n-cube	n-demicube	1k2 • 2k1 • k21	n-pentagonal polytope
Topics: Polytope families • Regular polytope • List of regular polytopes and compounds