沙普利-福克曼引理

沙普利-福克曼引理是凸几何（英语：Convex geometry）的一条引理，其于数理经济学有应用。引理描述向量空间子集的闵可夫斯基和有何性质。若干个集合的闵可夫斯基和，即从各集合分别取一个元素相加，组成的集合：例如，将整数${\displaystyle 0}$和${\displaystyle 1}$组成的集合，与自身相加，得到由${\displaystyle 0,\ 1,\ 2}$组成的集合，以符号可写成：

${\displaystyle \{0,1\}+\{0,1\}=\{0,1,2\}.}$

此处以四个集合的闵可夫斯基和为沙普利-福克曼引理的例证。在左边，四个非凸集中的点(+)之和，就是右边，其闵氏和凸包中的点(+)。左边四点之中，有两点位于相应的非凸集中，另有两点位于非凸集的凸包中。各凸包以浅粉红色画出。原来的集合各只有两点（以红点表示）。^[1]

“许多个集合的闵氏和，是否必定近似凸集？”沙普利-福克曼引理和相关的结果表明，问题的答案为肯定。^[2]集合称为凸，意思是连接其中任意两点的线段，必为该集合的子集：举例，实心圆盘 ${\displaystyle \bullet }$ 为凸集，但圆 ${\displaystyle \circ }$ 则不然，因为连接相异两点的线段 ${\displaystyle \oslash }$ 并不是圆的子集。沙普利-福克曼引理大致断言，若求和项的数目超出向量空间的维数，则其闵氏和将近凸。^[1]

沙普利-福克曼引理引入时，是作为证明沙普利-福克曼定理的一步，该定理断言闵氏和与其凸包的距离不超过某个上界。所谓集合${\displaystyle Q}$的凸包，是包含${\displaystyle Q}$的最小凸集。而当且仅当和为凸时，上述距离为零。定理中，距离的上界取决于维数${\displaystyle D}$及求和项的形状，但不取决于求和的项数${\displaystyle N}$，而只需${\displaystyle N>D}$。只要其中${\displaystyle D}$个求和项的形状，就足以确定${\displaystyle N}$个集合的闵可夫斯基平均集

${\displaystyle {\frac {1}{N}}\left(Q_{1}+Q_{2}+\cdots +Q_{N}\right)}$

与其凸包的距离的上界。当${\displaystyle N}$趋向无穷时，该上界递减至零（需要各求和项的大小一致有界）。^[3]斯塔（Starr）的推论将沙普利-福克曼定理的上界压得更低，故又称为沙普利-福克曼-斯塔定理。

劳埃德·沙普利和强·福克曼（英语：Jon Folkman）的引理，最先由经济学家罗斯·斯塔（英语：Ross Starr）发表，其时斯塔正在肯尼斯·阿罗门下，研究经济均衡的存在性。^[1]斯塔在论文中，研究凸化（convexified）经济体，即将非凸集换成其凸包。斯塔证明，凸化之后，有些均衡由原经济体的“准均衡”（quasi-equilibria）逼近；还证明，每个准均衡都具有真均衡的许多最优性质，而凸经济体中则必定存在真均衡。斯塔1969年的论文发表后，沙普利-福克曼-斯塔的结果得到广泛应用，用作证明（凸）经济理论的若干核心结果，尽管不适用于有非凸部分的大经济体，但在此等经济体中，仍是良好的近似。罗歇·盖内里（英语：Roger Guesnerie）评论：“这些结论的一般形式的推导，是战后经济理论的重大成就。”^[4]许多诺贝尔奖得主都曾研究经济学中的非凸集（英语：Non-convexity (economics)），除了前述的劳埃德·沙普利（2012年获奖）外，还有：阿罗（1972）、罗伯特·约翰·奥曼（2005）、杰拉德·德布鲁（1983）、特亚林·科普曼斯（1975）、保罗·克鲁曼（2008）、保罗·萨缪尔森（1970）。至于互补的主题“经济学中的凸集（英语：Convexity in economics）”，除以上得主著重外，还有里奥尼德·赫维克兹、列昂尼德·维塔利耶维奇·康托罗维奇（1975）、罗伯特·索洛（1987）都著重。

沙普利-福克曼引理在优化论和概率论皆有应用。^[3]优化论中，可以引理解释，在求多个函数之和的最小值时，为何一些解法可以成功。^[5][6]概率论中，引理适用于证明随机集（英语：Stochastic geometry）的“大数定律”。此定理先前仅在凸集的情况得证。^[7]

简单例子

考虑凸的实数区间${\displaystyle [0,1]}$，其包含整数子集${\displaystyle \{0,1\}}$，且是其凸包（添加了两点所连线段上的所有点）。仅得两个元素的集合${\displaystyle \{0,1\}}$，复制成三份，并按元素求和，得到

${\displaystyle \{0,1\}+\{0,1\}+\{0,1\}=\{0,1,2,3\}.}$

（此处集合的和，是从各集合分别取一个元素相加，得到的可能结果的集合，称为闵氏和。）和集的凸包则为区间${\displaystyle [0,3]}$。

区间${\displaystyle [0,3]}$中，每个数${\displaystyle x}$都可以写成区间${\displaystyle [0,1]}$中某三个实数（允许重复）之和，例如将${\displaystyle x}$等分成三份即可。但使用沙普利-福克曼引理，则有更强的结论：${\displaystyle [0,3]}$的每个数，都可以写成${\displaystyle \{0,1\}}$中某两个整数（允许重复），与${\displaystyle [0,1]}$中某一个实数之和。^[8]

凸包${\displaystyle [0,1]}$中的点，到${\displaystyle \{0,1\}}$的距离，至多为${\displaystyle 1/2}$：

${\displaystyle {\frac {1}{2}}=\left|{\frac {1}{2}}-0\right|=\left|{\frac {1}{2}}-1\right|,}$

然而，考虑三个区间${\displaystyle [0,1]}$的闵氏平均

${\displaystyle {\frac {1}{3}}\left(\{0,1\}+\{0,1\}+\{0,1\}\right)=\left\{0,{\frac {1}{3}},{\frac {2}{3}},\ 1\right\},}$

与其凸包${\displaystyle [0,1]}$的距离，仅为${\displaystyle 1/6}$，即未平均前${\displaystyle \{0,1\}}$与${\displaystyle [0,1]}$距离（${\displaystyle 1/2}$）的三分之一。越多个集合相加，其闵氏平均就将凸包填得越满：由闵氏平均至凸包的最远距离，随被加项数增加，而趋向于零。^[8]此为沙普利-福克曼定理的结论。

前置概念

沙普利-福克曼引理需要用到凸几何（英语：convex geometry）的若干定义和定理，本节将作简介。

实向量空间

二维的实向量空间上，有笛卡儿坐标系，将每点视为一对实数，称为“坐标”，按惯例记为${\displaystyle x,\ y}$。笛卡儿平面上的两点，可以逐个坐标相加：

${\displaystyle (x_{1},y_{1})+(x_{2},y_{2})=(x_{1}+x_{2},y_{1}+y_{2}).}$

此外，点也可以逐个坐标与实数${\displaystyle \lambda }$相乘：

${\displaystyle \lambda (x,y)=(\lambda x,\lambda y).}$

更一般而言，任何${\displaystyle D}$维（有限维）实向量空间，均可视为${\displaystyle D}$个实数组成的D元组的集合${\displaystyle \{(v_{1},v_{2},\ldots ,v_{D})\}}$，并配备两种运算：向量加法和标量乘法。有限维实空间的此两种运算，皆定义成逐个坐标运算，与笛卡儿平面上的运算类似。^[9]

凸集

凸集${\displaystyle Q}$中，连接任意两点的线段仍是${\displaystyle Q}$的子集。

非凸集${\displaystyle Q}$中，连接某两点的线段上，有一点不再是${\displaystyle Q}$的元素。

用线段可以检验某集合是否凸集。

实向量空间中，非空子集${\displaystyle Q}$称为凸集，意思是，对于${\displaystyle Q}$的任意两个点，连接两点成一线段，其上的所有点仍在${\displaystyle Q}$中。例如，实心圆盘 ${\displaystyle \bullet }$ 为凸，但圆 ${\displaystyle \circ }$ 则不然，因为连接相异两点的线段 ${\displaystyle \oslash }$ 并不是圆的子集。三个整数的集合${\displaystyle \{0,1,2\}}$非凸，但其为区间${\displaystyle [0,2]}$的子集，而该区间为凸。又例如，实心立方体为凸，然而任何空心或凹陷的图形，如弯月形（英语：Crescent），则非凸。空集也是凸集，视乎作者偏好，这可能是专门的定义^[10]，也可能是因为要满足的条件是空真命题（英语：Vacuous truth）。

更严谨而言，集合${\displaystyle Q}$称为凸，意思是，对${\displaystyle Q}$中任意两点${\displaystyle v_{0},v_{1}}$，和单位区间${\displaystyle [0,1]}$中的任意实数${\displaystyle \lambda }$，点

${\displaystyle (1-\lambda )v_{0}+\lambda v_{1}}$

仍是${\displaystyle Q}$的元素。

由数学归纳法，集合${\displaystyle Q}$为凸，当且仅当其任意多个元素的凸组合仍在${\displaystyle Q}$中。所谓向量空间子集${\displaystyle \{v_{0},v_{1},\ldots ,v_{D}\}}$的凸组合，是其元素的任意加权平均${\displaystyle \lambda _{0}v_{0}+\lambda _{1}v_{1}+\cdots +\lambda _{D}v_{D}}$，而各${\displaystyle \lambda _{i}}$可以是总和为${\displaystyle 1}$的任意非负实数，即只需${\displaystyle \lambda _{0}+\lambda _{1}+\cdots +\lambda _{D}=1}$。^[11]

凸集的定义推出，两个凸集的交集仍是凸集。更甚者，任意一族凸集的交也是凸集。作为特例，若取两个不交的凸集，则其交集为空集，故应当称空集为凸。^[10]

凸包

红色集的凸包中，每个蓝点都是若干个红点的凸组合。

对于实向量空间的每个子集${\displaystyle Q}$，其凸包${\displaystyle \mathrm {Conv} (Q)}$是包含${\displaystyle Q}$的最小凸集。所以，${\displaystyle \mathrm {Conv} (Q)}$是所有覆盖${\displaystyle Q}$的凸集的交。等价地，可以将${\displaystyle \mathrm {Conv} (Q)}$定义成${\displaystyle Q}$的点的所有凸组合的集合。^[12]作为例子，整数集合${\displaystyle \{0,1\}}$的凸包，是实数闭区间${\displaystyle [0,1]}$，其两端为原有的整数${\displaystyle 0,1}$。^[8]单位圆的凸包则是闭单位圆盘，其包含单位圆。

闵可夫斯基和

集合的闵可夫斯基和。两个小正方形${\displaystyle Q_{1}=[0,1]^{2}}$和${\displaystyle Q_{2}=[1,2]^{2}}$的和，是大正方形${\displaystyle Q_{1}+Q_{2}=[1,3]^{2}}$。

在任何向量空间（或有定义加法的代数结构）${\displaystyle X}$中，两个非空子集${\displaystyle A,B\subseteq X}$的闵可夫斯基和，定义为其元素之和的集合，即 ${\displaystyle A+B:=\{x+y\mid x\in A,~y\in B\}}$（参见^[13])。例如：

${\displaystyle \{0,1\}+\{0,1\}=\{0+0,0+1,1+0,1+1\}=\{0,1,2\}.}$

此运算定义在非空子集族上，且满足结合律和交换律。既然有结合律和交换律，就可以递归定义多个被加项的运算结果${\displaystyle \sum _{n=1}^{N}Q_{n}=Q_{1}+Q_{2}+\cdots +Q_{N}}$。由数学归纳法，可见^[14]

${\displaystyle \sum _{n=1}^{N}Q_{n}=\left\{\left.\sum _{n=1}^{N}q_{n}\right|q_{n}\in Q_{n},~1\leq n\leq N\right\}.}$

闵可夫斯基和的凸包

闵可夫斯基和与凸包运算可以交换。具体而言，对实向量空间${\displaystyle X}$的任意子集${\displaystyle A,B\subseteq X}$，其闵氏和的凸包等于其凸包的闵氏和。换言之，

${\displaystyle \mathrm {Conv} (A+B)=\mathrm {Conv} (A)+\mathrm {Conv} (B).}$

故由数学归纳法得

${\displaystyle \mathrm {Conv} (\sum _{n=1}^{N}Q_{n})=\sum _{n=1}^{N}\mathrm {Conv} (Q_{n})}$

对任意${\displaystyle N\in \mathbb {N} }$和非空子集${\displaystyle Q_{n}\subseteq X}$（${\displaystyle 1\leq n\leq N}$）成立。^[15][16]

各命题的叙述

闵氏和及凸包。右图的十六个红点，组成左图四个非凸集的闵氏和。左图每个非凸集由两个红点组成，其凸包（浅粉红色）包含标记加号（+）的点，而右图中的加号等于左图各个标加号的点之和。

由前一段的恒等式，对和的凸包中的每点${\displaystyle x\in \mathrm {Conv} \left(\sum _{n=1}^{N}Q_{n}\right)}$，都存在各凸包的${\displaystyle q_{n}(x)\in \mathrm {Conv} (Q_{n})}$（${\displaystyle n}$取遍${\displaystyle 1}$至${\displaystyle N}$），使得${\displaystyle \sum _{n=1}^{N}q_{n}(x)=x}$。各点${\displaystyle q_{n}(x)}$的位置取决于${\displaystyle x}$。

引理

劳埃德·沙普利，2012年诺贝尔经济学奖得主。他与强·福克曼（英语：Jon Folkman）一同证明沙普利-福克曼引理。^[1]

有了以上背景，沙普利-福克曼引理断言，${\displaystyle x}$的表示法

${\displaystyle x=\sum _{n=1}^{N}q_{n}(x)}$

中，仅需要不多于${\displaystyle D}$个被加项${\displaystyle q_{n}(x)}$取自凸包，而其他被加项，则只需取自原来的集合${\displaystyle Q_{n}}$。以符号复述，即有以上方法表示${\displaystyle x}$，且满足${\displaystyle |\{l\leq n\leq N\mid q_{n}(x)\in \mathrm {Conv} (Q_{n})\setminus Q_{n}\}|\leq D}$。不妨将下标重新排序，然后就有

${\displaystyle x=\sum _{n=1}^{D}q_{n}(x)+\sum _{n=D+1}^{N}q_{n}(x)}$

其中对${\displaystyle 1\leq n\leq D}$，有${\displaystyle q_{n}\in \mathrm {Conv} (Q_{n})}$，而对${\displaystyle D+1\leq n\leq N}$，则有${\displaystyle q_{n}\in Q_{n}}$。注意，倘若重排，则排序的方式也取决于点${\displaystyle x}$。^[17]再精简，沙普利-福克曼引理可以写成

${\displaystyle \mathrm {Conv} (\sum _{n=1}^{N}Q_{n})\subseteq \bigcup _{I\subseteq \{1,2,\ldots N\}:~|I|=D}\sum _{n\in I}\mathrm {Conv} (Q_{n})+\sum _{n\notin I}Q_{n}.}$

举例，集合${\displaystyle [0,2]=[0,1]+[0,1]=\mathrm {Conv} (\{0,1\})+\mathrm {Conv} (\{0,1\})}$中的每个点，根据引理，必定可以写成${\displaystyle \{0,1\}}$的某个元素，与${\displaystyle [0,1]}$的某个元素之和。^[8]

实向量空间的维数

反之，沙普利-福克曼引理刻划了有限维实向量空间的维数。具体而言，若某向量空间，对于某个自然数${\displaystyle D}$满足引理的结论，但对小于${\displaystyle D}$的数不满足，则其维数恰好为${\displaystyle D}$。^[18]引理仅对有限维向量空间成立。^[19]

沙普利-福克曼定理及斯塔的推论

某点集（深红）的凸包以浅红虚线围起。点集的内半径（绿色）必定小于其外接圆半径（蓝色），除非所有点共圆，此时两个半径相等。

沙普利与福克曼运用其引理，证明沙普利-福克曼定理，给出集合的闵氏和与其凸包（称为凸化（convexified）和）的距离上界。定理的叙述如下：

凸化和${\displaystyle \mathrm {Conv} \left(\sum Q_{n}\right)}$的任一点，到（未凸化）和集${\displaystyle \sum Q_{n}}$的欧氏距离平方，不超过各集合${\displaystyle Q_{n}}$的外接半径中，最大${\displaystyle D}$个的平方和。(所谓外接半径，定义为包围该集合的最小球面的半径。）^[20]此上界与求和项数${\displaystyle N}$无关（但要求${\displaystyle N>D}$，且集合不能越来越大）。^[21]

上述定理给出闵氏和及其凸包之间距离的上界。当且仅当闵氏和为凸集时，此距离为零。该上界取决于维数${\displaystyle D}$及各被加项的形状，但只要${\displaystyle N>D}$，就不取决于项数${\displaystyle N}$。^[3]

通常，外接半径会大于内半径，而无论如何，外接半径总不能小于内半径。集合${\displaystyle Q_{n}}$的内半径定义为：^[22]

最小的正实数${\displaystyle r}$，满足：若${\displaystyle q}$在${\displaystyle Q_{n}}$凸包中，则${\displaystyle q}$在${\displaystyle Q_{n}}$若干点的凸包中，而该些点皆在同一个半径为${\displaystyle r}$的球内。

斯塔将沙普利-福克曼定理中的外接半径换成内半径，从而压低沙普利-福克曼定理的上界：

沙普利-福克曼定理的斯塔推论：

凸化和${\displaystyle \mathrm {Conv} \left(\sum Q_{n}\right)}$的任一点，到（未凸化）和集${\displaystyle \sum Q_{n}}$的欧氏距离平方，不超过各集合${\displaystyle Q_{n}}$的内半径中，最大${\displaystyle D}$个的平方和。^[22][23]

斯塔的推论确定，${\displaystyle N}$个集合的闵氏和与其凸包之间，欧氏距离的上界。此距离可以衡量闵氏和非凸的程度，故为简单起见，下文称为非凸度。于是，斯塔对非凸度的上界，仅取决于${\displaystyle D}$个最大内半径之值，但不取决于求和的项数${\displaystyle N}$（假设${\displaystyle N>D}$）。

例如，非凸集${\displaystyle \{0,1,2\}}$的非凸度为${\displaystyle 1/2}$，因为与其凸包（区间${\displaystyle [0,2]}$）的距离为

${\displaystyle {\frac {1}{2}}=\left|1-{\frac {1}{2}}\right|=\left|0-{\frac {1}{2}}\right|=\left|2-{\frac {3}{2}}\right|=\left|1-{\frac {3}{2}}\right|.}$

所以，既然${\displaystyle \sum Q_{n}}$的非凸度有不取决于项数${\displaystyle N}$的上界，就知平均集

${\displaystyle {\frac {1}{N}}\left(\sum Q_{n}\right)}$

非凸度上界，会随${\displaystyle N}$增加而递减。例如，平均集

${\displaystyle {\frac {1}{2}}\left(\{0,1\}+\{0,1\}\right)=\{0,\ 1/2,\ 1\}}$

和其凸包${\displaystyle [0,1]}$的距离仅为${\displaystyle 1/4}$，等于被加项${\displaystyle \{0,1\}}$与其凸包${\displaystyle [0,1]}$的距离（${\displaystyle 1/2}$）之半。仅要最大${\displaystyle D}$个求和项的形状，已足以计算和集与凸包的距离的上界，故除以${\displaystyle N}$后，平均集与凸包的距离，在${\displaystyle N}$趋向无穷时，会递减至零（对均匀有界的求和项成立，即各个求和项的大小需有同一个上界）。^[3]若使用斯塔的上界，则前一句结论的条件可以放宽，只需各求和项的内半径有同一个上界。^[3]

证明和计算

沙普利-福克曼引理的原证明，仅确定存在该种表示法，而无说明如何构造。阿罗与哈恩^[24]、盖士利（英语：J. W. S. Cassels）^[25]、斯奈德（Schneider）^[26]和其他人都曾给出类似的证明。阿特斯泰因（Artstein）扩展了埃科兰德（英语：Ivar Ekeland）的简洁抽象证明。^[27][28]也有未经出版的另证。^[2][29]1981年，斯塔发表一种叠代法，可以计算给定点的表示法，然而，此计算方法给出的上界，较非构造性证明的上界差。^[30]博赛卡斯的书有讲述有限维空间沙普利-福克曼引理的初等证明，^[31]并将引理应用于估计可分优化（separable optimization）问题与零和赛局的对偶间隙。

应用

有沙普利-福克曼引理，学者就得将有关凸集闵氏和的结果，套用到（无需凸的）一般集合之和。此种和见于经济学、最优化理论、概率论。此三领域的应用中，非凸性起到重要作用。

经济学

消费者偏好位于无差异曲线${\displaystyle I_{3}}$上的任意篮子，胜过位于${\displaystyle I_{2}}$上的篮子。篮子${\displaystyle (Q_{x},Q_{y})}$，是预算线（蓝色）支撑${\displaystyle I_{2}}$的位置，所以是最优而又可行。相反，虽然消费者更偏好${\displaystyle I_{3}}$上的任意篮子，但无足够预算，所以并不可行。

参见：经济学中的凸集（英语：Convexity in economics）

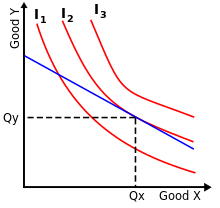
经济学中，消费者对每一“篮子”商品（basket，即商品的组合）有其偏好程度。每篮子可用一个非负向量表示，其坐标为篮中各商品的量。所有篮子组成的集合中，每个消费者有自己的一族无差异曲线，满足：在同一条曲线上的各篮子，对该消费者是等价的，即消费者并不觉该曲线上有篮子胜于另一个篮子。每篮子恰好处于一条无差异曲线上。消费者相对于一条无差异曲线的偏好集，定义为该无差异曲线与其更偏好的区域的并集。称消费者的偏好为凸，意思是其所有偏好集皆为凸。^[32]

如图所示，消费者认为的最优篮子，是在预算线支撑某个偏好集时取到，因为此时，所选的篮子是整条预算线上，达到最高的无差异曲线的一点。所谓预算线，是由商品的价格向量与消费者的收入计算得出的限制，消费者无足够收入购买高于此线的篮子。所以，最优篮子的集合是各价格的函数，称为消费者的需求。若偏好集为凸，则不论价格为何，消费者认为的最优篮子总是组成凸集，例如可能是单元集，或是一条线段。^[33]

非凸偏好

若消费者的偏好有凹陷，则消费者的决定可以不连续，从一个篮子跳到另一个隔开的篮子。

参见：非凸集 (经济学)（英语：Non-convexity (economics)）

然而，若有偏好集非凸，则某些价格确定的预算线，可能在两个分开的最优篮子支撑该偏好集。例如，可以设想，动物园购买狮子或鹰的价钱一样，且其预算恰好够买一只狮子或一只鹰。此外，假设园主亦认为两只动物价值相同，则动物园有可能买狮子，也可能买鹰，但当然不可能买半只狮子加半只鹰（狮鹫）。所以，园主的偏好非凸：买两只动物中的任一只，胜于两者的严格凸组合。^[34]

若消费者有非凸偏好集，则对于某些价格，需求不连通。不连通的需求，会导致消费者的行为出现不连续的间断。引述哈罗德·霍特林的话（宜配合所附动图理解）：

若考虑波浪形的无差异曲线，即在某些区域向原点凸，而在其他区域向原点凹，则我等被迫推论，仅有向原点凸的部分需要理会，因为几乎不可能观测到其他部分。要侦测该些部分，唯一方法是，观察价格之比率变化时，需求的不连续变化。此变化的效果是，当直线旋转时，切点会突然跃过某凹陷。虽然此种不连续的表现，揭示有凹陷，但却不可能量度缺口的深度。倘若无差异曲线，或其高维推广，有凹陷部分，则定必因永远无法测量，而不为人知。^[35]

瓦尔特·迪韦尔特（英语：Walter Erwin Diewert）^[36]书中，称赫尔曼·沃尔德（英语：Herman Wold）^[37]有强调研究非凸偏好的困难，也引述保罗·萨缪尔森称凹陷处“被永恒的黑暗遮蔽”^[38]。

尽管有上述困难，《政治经济期刊（英语：Journal of Political Economy）》（JPE）在1959年至1961年间，刊登一系列的论文，解明非凸偏好。投稿人包括：法雷尔（Farrell）^[39]、巴托尔（英语：Francis M. Bator）^[40]、科普曼斯^[41]、罗森博格（Rothenberg）^[42]。其中，罗森博格讨论非凸集和的近似凸性。^[43]该些JPE论文，促使劳埃德·沙普利和马丁·舒比克也合著论文，研究凸化的消费者偏好，并引入“近似均衡”（approximate equilibrium）的概念。^[44]JPE论文和沙普利-舒比克论文又启发了罗伯特·奥曼提出另一个概念，称为“准均衡”（quasi-equilibrium）。^[45][46]

斯塔1969年的论文与当代经济学

肯尼斯·阿罗（1972年诺贝尔奖得主）帮助罗斯·斯塔（英语：Ross Starr）研究非凸经济体（英语：Convex preferences）。^[47]

肯尼斯·阿罗收集前人研究经济学中的非凸集（英语：Non-convexity (economics)）的文献，列成表，并加入注解，交给罗斯·斯塔（英语：Ross Starr）。斯塔当时仍是本科生，但已在修读阿罗开设的高等数理经济学（研究生）课程。^[47]斯塔在学期论文中，考虑将非凸偏好换成其凸包所得的假想经济体，研究其一般均衡。凸化经济体中，在每个价位，总需求皆是各消费者需求的凸包之和。斯塔的想法吸引数学家劳埃德·沙普利和强·福克曼（英语：Jon Folkman）参与，两人“在私人通信中”证明现以两人命名的引理和定理，到1969年，斯塔才于论文报告此事。^[1]

斯塔1969年的论文中，应用沙普利-福克曼-斯塔定理，证明“凸化”经济体有一些一般均衡，只要参与者足够多，能以原经济体的“准均衡”近似。具体而言，斯塔证明，至少存在一个价格向量为${\displaystyle p_{\mathrm {opt} }}$的准均衡，满足下列条件：

• 对每个准均衡${\displaystyle p_{\mathrm {opt} }}$，所有消费者都可以选到其最优的篮子（在预算限制内，且最偏好）。
• 在该价格${\displaystyle p_{\mathrm {opt} }}$，凸化经济体中，每种商品的市场皆均衡，即供给等于需求。
• 每个准均衡的价格，皆“几乎出清”原经济体的市场：凸化经济体均衡组成的集合，与原经济体的准均衡集合，两者的距离有上界。此结论是根据沙普利-福克曼定理的斯塔推论得到。^[48]

斯塔确立以下结论：

“总体中，［取各消费及生产集的凸包］所得的假想经济体的分配，与真实经济体的某个分配，两者的差异，有不取决于参与者数目的上界。因此，当参与者数目趋向无穷时，平均参与者感受到，与拟作行动的偏差，近乎可以忽略不计。”^[49]

斯塔1969年论文发表后，沙普利-福克曼-斯塔的结论，在经济理论获广泛应用。罗歇·盖内里（英语：Roger Guesnerie）如此总结该结论对经济学的意义：“假设凸性，所得的若干重要结果，在不具凸性的情况下，仍（近似）适用。例如，若经济体具有大消费侧，则偏好的非凸性不影响适用标准结果”^[50]，还称“这些结论的一般形式的推导，是战后经济理论的重大成就。”^[4]许多诺贝尔奖得主都曾研究经济学中的非凸集（英语：Non-convexity (economics)），除了前述的劳埃德·沙普利（2012年获奖）外，还有：阿罗（1972）、罗伯特·约翰·奥曼（2005）、杰拉德·德布鲁（1983）、特亚林·科普曼斯（1975）、保罗·克鲁曼（2008）、保罗·萨缪尔森（1970）。至于互补的主题“经济学中的凸集（英语：Convexity in economics）”，除以上得主著重外，还有里奥尼德·赫维克兹、列昂尼德·维塔利耶维奇·康托罗维奇（1975）、罗伯特·索洛（1987）都著重。^[51]

沙普利-福克曼-斯塔的结论，在经济学各分支的文献都经常出现，包括微观经济学^[52]、一般均衡理论^[53][54]、公共经济学^[55]（包括市场失灵）^[56]、博弈论^[57]、数理经济学^[58]、经济学中的应用数学^[59][60]。沙普利-福克曼-斯塔的结论，也使经济学更多使用测度论和积分理论。^[61]

最优化理论

函数称为凸，意思是图像上方的区域为凸集。

沙普利-福克曼引理可以解释，为何大规模的最小化问题，即使非凸，仍可用迭代法近似求解（仅对于凸问题，有证明叠代法收敛到最优解）。沙普利-福克曼引理，促使学者将凸优化方法，用于优化多个（无需凸的）函数之和。^[62]

最优化理论的前置概念

非线性规划依赖下列有关函数的若干定义：

• 函数${\displaystyle f}$（定义在集合${\displaystyle D}$上）的图像，是所有参数${\displaystyle x}$与相应取值${\displaystyle f(x)}$组成的二元组的集合：

${\displaystyle \mathrm {Graph} (f)=\{(x,f(x)):x\in D\}.}$

• 实值函数（英语：Real-valued function）${\displaystyle f}$的盖图（英语：Epigraph (mathematics)）是图像上方的点的集合：

正弦函数并非凸函数。

${\displaystyle \mathrm {Epi} (f)=\{(x,u):f(x)\leq u\}.}$

• 实值函数称为凸函数，意思是其盖图为凸集。^[63]

举例，二次函数${\displaystyle f:x\mapsto x^{2}}$为凸，而绝对值函数${\displaystyle g:x\mapsto |x|}$亦然，但正弦函数${\displaystyle \sin }$（如图）则不然，因为在区间${\displaystyle (0,\pi )}$的盖图非凸（而有向上的凹陷）。

加性优化问题

许多优化问题中，目标函数${\displaystyle f}$可分离变数（可分），即${\displaystyle f}$是多个函数之和，而各个函数的参数不同，如：

${\displaystyle f({\boldsymbol {x}})=f(x_{1},x_{2},\ldots ,x_{n})=\sum _{n}f_{n}(x_{n}).}$

又例如，线性规划问题就可分离变数。考虑一个可分问题，及一个最优解

${\displaystyle {\boldsymbol {x}}_{\mathrm {min} }=(x_{1},x_{2},\ldots ,x_{n})_{\mathrm {min} },}$

在该点取到最小值${\displaystyle f({\boldsymbol {x}}_{\mathrm {min} })}$。对此可分问题，同时考虑其“凸化问题”的最优解，即将每个求和项${\displaystyle f_{n}}$的图像换成其凸包。此种最优解，会是凸化问题的某点列${\displaystyle ({\boldsymbol {x}}_{j},f({\boldsymbol {x}}_{j}))_{j=1}^{\infty }}$的极限，其中

${\displaystyle ({\boldsymbol {x}}_{j},f({\boldsymbol {x}}_{j}))\in \sum _{n=1}^{N}\mathrm {Conv} (\mathrm {Graph} (f_{n})).}$^[5][64]

当然，因为此点是${\displaystyle N}$个图像凸包的点之和，由沙普利-福克曼引理，就可以写成原图像的点与少数图像凸包的点之和。

以上分析，1974年由埃科兰德（英语：Ivar Ekeland）发表，以解释为何可分问题有许多项时，即使各项非凸，总问题仍看似凸。对非线性最小值问题而言，对偶问题的解，不一定就是原问题的解（但若已知原问题为凸，且满足特定条件，则两者的的最优解相等），但是，1973年，青年数学家克劳德·勒马雷沙尔（英语：Claude Lemaréchal）诧异，对已知非凸的问题使用凸问题的方法，竟然也成功。勒马雷沙尔的问题，正是上段加性可分离变数的形式，而每个和项皆非凸函数，但对偶问题的解，仍近似原问题的最优解。^[65][5][66]埃科兰德的分析说明，“大而可分”的最小值问题，即使各和项有凹陷，仍可运用凸优化的方法。埃科兰德及其后的作者主张，因为变数可以加性分离，所得的总问题近似凸。该等著作以沙普利-福克曼引理为关键一步。^[5][66][67]沙普利-福克曼引理促使学者将凸优化方法，应用到目标函数为多个函数之和的情况。^{[5][6][59][62]}

概率与测度论

凸集常与概率论一同研究。卡拉西奥多里定理（英语：Carathéodory's theorem (convex hull)）说明，${\displaystyle d}$维空间的（非空）子集${\displaystyle Q}$凸包中的点，是取值于${\displaystyle Q}$的简单随机向量（英语：Multivariate random variable）的期望值，而所谓简单，意思是仅在不多于${\displaystyle d+1}$个点处有非零概率。所以，对非空集${\displaystyle Q}$，取值自${\displaystyle Q}$的简单随机向量组成的集合，等于${\displaystyle Q}$的凸包。由此便可在概率论中，套用沙普利-福克曼-斯塔的结果。^[68]反之，藉期望值与凸包之间的联系，概率论亦能用作研究凸集，尤其可用作分析沙普利-福克曼-斯塔的结果。^[69]沙普利-福克曼-斯塔的结果，广泛用于随机集的概率论（英语：Stochastic geometry）^[70]，例如证明随机集的大数定律^[7][71]、中央极限定理^[71][72]、大离差原理（英语：Large deviations theory）^[73]。要证明前列概率极限定理，可以使用沙普利-福克曼-斯塔的结果，来避免假设随机集为凸。

概率测度是有限的测度，而沙普利-福克曼引理还可以应用到非概率的测度论，如体积或向量测度的理论。沙普利-福克曼引理可以加强布伦-闵可夫斯基不等式（英语：Brunn–Minkowski theorem）。该不等式断言，闵氏和的体积，相较于各和项的体积不能太大，即闵氏和的体积有上界，以各和项的体积的表示。^[74]欧氏空间子集的体积，此处定义为其勒贝格测度。

高等测度论中，沙普利-福克曼引理适用于证明李亚普诺夫定理，即向量测度的值域为凸。^[75]值域（或像集）是函数所有可能取值的集合，而向量测度则将测度推广到允许取向量值。例如，若某测度空间有两种概率测度${\displaystyle p_{1}}$和${\displaystyle p_{2}}$，则可定义一个向量测度${\displaystyle (p_{1},p_{2})}$，是对每个事件${\displaystyle \omega }$，将其两个概率结合为一个二元组，即

${\displaystyle (p_{1},p_{2})(\omega )=(p_{1}(\omega ),p_{2}(\omega )).}$

李亚普诺夫定理在经济学^[45][76]、(砰砰)控制论、统计理论（英语：Statistical theory）皆有应用。^[77]李亚普诺夫定理是沙普利-福克曼引理的连续版^[3]，而沙普利-福克曼引理是李亚普诺夫定理的离散类比。^[78]

注

1. Starr (1969)
2. Howe (1979，第1页)
3. Starr (2008) harvtxt error: multiple targets (2×): CITEREFStarr2008 (help)
4. Guesnerie (1989，第138页)
5. (Ekeland 1999，第357–359页): 1976年首部英文版中，埃科兰德在附录证明沙普利-福克曼引理，并在p. 373提到勒马雷沙尔的实验观察。
6. Bertsekas (1996，第364–381页)于p. 374引用Ekeland (1999)，并在p. 381引用Aubin & Ekeland (1976)：

   Bertsekas, Dimitri P. 5.6 Large scale separable integer programming problems and the exponential method of multipliers [第5.6节：大规模可分整数规划问题及乘子指数法]. Constrained optimization and Lagrange multiplier methods [受限优化和拉格朗日乘子法] 1982年Academic Press版的重印. Belmont, Mass.: Athena Scientific. 1996: xiii+395. ISBN 1-886529-04-3. MR 0690767 （英语）.

   Bertsekas (1996，第364–381页)将拉格朗日对偶法用到发电排程（英语：Scheduling (production processes)）上（即机组排程问题（英语：power system simulation）），此种问题有变量限制为整数，所以非凸：

   Bertsekas, Dimitri P.; Lauer, Gregory S.; Sandell, Nils R., Jr.; Posbergh, Thomas A. Optimal short-term scheduling of large-scale power systems [大规模电力系统的最优短期排程] (PDF). IEEE Transactions on Automatic Control. January 1983, 28 (1): 1–11 [2 February 2011]. doi:10.1109/tac.1983.1103136. （原始内容存档 (PDF)于2021-09-09） （英语）. Proceedings of 1981 IEEE Conference on Decision and Control, San Diego, CA, December 1981, pp. 432–443.

7. Artstein & Vitale (1975，第881–882页): Artstein, Zvi; Vitale, Richard A. A strong law of large numbers for random compact sets [随机紧集的强大数定律]. The Annals of Probability. 1975, 3 (5): 879–882. JSTOR 2959130. MR 0385966. Zbl 0313.60012. doi:10.1214/aop/1176996275 （英语）.
8. Carter (2001，第93–94页)，取n = 3。
9. Arrow & Hahn (1980，第375页)
10. Rockafellar (1997，第10页)
11. Arrow & Hahn (1980，第376页)、Rockafellar (1997，第10–11页)、Green & Heller (1981，第37页)
12. Arrow & Hahn (1980，第385页)及Rockafellar (1997，第11–12页)
13. Schneider (1993，第xi页)及Rockafellar (1997，第16页)
14. Rockafellar (1997，第17页)及Starr (1997，第78页)
15. Schneider (1993，第2–3页)
16. Arrow & Hahn (1980，第387页)
17. Starr (1969，第35–36页)
18. Schneider (1993，第131页)
19. Schneider (1993，第140页)归功于Borwein & O'Brien (1978): Borwein, J. M.; O'Brien, R. C. Cancellation characterizes convexity [抵消之事，可以刻画凸性]. Nanta Mathematica (Nanyang University). 1978, 11: 100–102. ISSN 0077-2739. MR 0510842 （英语）.
20. Schneider (1993，第129页)
21. Starr (1969，第36页)
22. Starr (1969，第37页)
23. Schneider (1993，第129–130页)
24. Arrow & Hahn (1980，第392–395页)
25. Cassels (1975，第435–436页)
26. Schneider (1993，第128页)
27. Ekeland (1999，第357–359页)
28. Artstein (1980，第180页)
29. Anderson (2005) harvtxt error: multiple targets (2×): CITEREFAnderson2005 (help)
30. Starr, Ross M. Approximation of points of convex hull of a sum of sets by points of the sum: An elementary approach [以集合之和的点迫近和集凸包的点：初等进路]. Journal of Economic Theory. 1981, 25 (2): 314–317. MR 0640201. doi:10.1016/0022-0531(81)90010-7 （英语）.
31. Bertsekas, Dimitri P. Convex Optimization Theory [凸优化论]. Belmont, Mass.: Athena Scientific. 2009. ISBN 978-1-886529-31-1 （英语）.
32. Mas-Colell (1985，第58–61页) and Arrow & Hahn (1980，第76–79页)
33. Arrow & Hahn (1980，第79–81页)
34. Starr (1969，第26页)：“毕竟，可能觉得车和艇差不多，但多数情况下，半车半艇的组合，既不能驾驶，也不能航行。”（译文）
35. Hotelling (1935，第74页): Hotelling, Harold. Demand functions with limited budgets [有限预算的需求函数]. Econometrica. January 1935, 3 (1): 66–78. JSTOR 1907346. doi:10.2307/1907346 （英语）.
36. Diewert (1982，第552–553页)
37. Wold (1943b，第231 and 239–240页): Wold, Herman. A synthesis of pure demand analysis II [纯需求分析综论二]. Skandinavisk Aktuarietidskrift [斯堪的纳维亚精算期刊]. 1943b, 26: 220–263. MR 0011939. doi:10.1080/03461238.1943.10404737.

    Wold & Juréen (1953，第146页): Wold, Herman; Juréen, Lars (in association with Wold). 8 Some further applications of preference fields (pp. 129–148) [八、偏好域的其他应用]. Demand analysis: A study in econometrics [需求分析：计量经济学研究]. Wiley publications in statistics. New York: John Wiley and Sons, Inc. 1953: xvi+358. MR 0064385 （英语）.

38. Samuelson (1950，第359–360页):

    会注意到，竞争市场中，不能观测到无差异曲线凸处（而不是凹）的任何点。此种点被永恒的黑暗遮蔽，除非我等令该消费者垄断买方，且从非常凸的“预算曲线”上，选取所买的商品。（其沿此曲线，影响所买商品的价格。）在买方垄断的情况，仍可从均衡点观测到的限制的斜算，推断该人无差异曲线的斜率。［译按：此处凸与凹的约定，与本条目相反。］

    Samuelson, Paul A. The problem of integrability in utility theory [效用论的可积性问题]. Economica. New Series. November 1950, 17 (68): 355–385. JSTOR 2549499. MR 0043436. doi:10.2307/2549499 （英语）.

    “永恒的黑暗”描述弥尔顿所著《失乐园》中的地狱，其卷二第592至594行将地狱的凹陷与塞波尼斯大沼泽（英语：Serbonian Bog）相比：

    A gulf profound as that Serbonian Bog
    Betwixt Damiata and Mount Casius old,
    Where Armies whole have sunk.

    弥尔顿对凹陷的描写，是Arrow & Hahn (1980，第169页)第7章"Markets with non-convex preferences and production"［非凸偏好与生产的市场］的题辞（英语：epigraph (literature)）。该章讲解Starr (1969)的成果。
39. Farrell, M. J. The Convexity assumption in the theory of competitive markets [竞争市场论的凸性假设]. The Journal of Political Economy. August 1959, 67 (4): 371–391. JSTOR 1825163. doi:10.1086/258197 （英语）.

    Farrell, M. J. On Convexity, efficiency, and markets: A Reply [论凸性、效率、市场：回复]. Journal of Political Economy. October 1961a, 69 (5): 484–489. JSTOR 1828538. doi:10.1086/258541 （英语）.

    Farrell, M. J. The Convexity assumption in the theory of competitive markets: Rejoinder [竞争市场论的凸性假设：再回应]. Journal of Political Economy. October 1961b, 69 (5): 493. JSTOR 1828541. doi:10.1086/258544 （英语）.

40. Bator, Francis M. On convexity, efficiency, and markets [论凸性、效率、市场]. The Journal of Political Economy. October 1961a, 69 (5): 480–483. JSTOR 1828537. doi:10.1086/258540 （英语）.

    Bator, Francis M. On convexity, efficiency, and markets: Rejoinder [论凸性、效率、市场：再回应]. Journal of Political Economy. October 1961b, 69 (5): 489. JSTOR 1828539. doi:10.1086/258542 （英语）.

41. Koopmans, Tjalling C. Convexity assumptions, allocative efficiency, and competitive equilibrium [凸假设、分配效率、竞争均衡]. The Journal of Political Economy. October 1961, 69 (5): 478–479. JSTOR 1828536. doi:10.1086/258539 （英语）.

    Koopmans (1961，第478页)、Farrell (1959，第390–391页)、Farrell (1961a，第484页)、Bator (1961a，第482–483页)、Rothenberg (1960，第438页)、Starr (1969，第26页)评论了Koopmans (1957，第1–126, 尤其 9–16 [1.3 Summation of opportunity sets]、 23–35 [1.6 Convex sets and the price implications of optimality]、 35–37 [1.7 The role of convexity assumptions in the analysis]三节页)：

    Koopmans, Tjalling C. Allocation of resources and the price system [资源分配与价格制度]. Koopmans, Tjalling C (编). Three essays on the state of economic science [三篇论经济科学现况]. New York: McGraw–Hill Book Company. 1957: 1–126. ISBN 0-07-035337-9 （英语）.

42. Rothenberg (1960，第447页): Rothenberg, Jerome. Non-convexity, aggregation, and Pareto optimality [非凸性、加总、帕累托最优]. The Journal of Political Economy. October 1960, 68 (5): 435–468. JSTOR 1830308. doi:10.1086/258363 （英语）.

    （Rothenberg, Jerome. Comments on non-convexity [评非凸性]. Journal of Political Economy. October 1961, 69 (5): 490–492. JSTOR 1828540. doi:10.1086/258543 （英语）.）

43. Arrow & Hahn (1980，第182页)
44. Shapley & Shubik (1966，第806页): Shapley, L. S.; Shubik, M. Quasi-cores in a monetary economy with nonconvex preferences [非凸偏好货币经济中的准核]. Econometrica. October 1966, 34 (4): 805–827 [2021-08-30]. JSTOR 1910101. Zbl 0154.45303. doi:10.2307/1910101. （原始内容存档于2017-09-24） （英语）.
45. Aumann (1966，第1–2页): Aumann, Robert J. Existence of competitive equilibrium in markets with a continuum of traders [市场有连续统多个交易人，则存在竞争均衡]. Econometrica. January 1966, 34 (1): 1–17. JSTOR 1909854. MR 0191623. doi:10.2307/1909854 （英语）. Aumann (1966)用到 Aumann （1964, 1965）的结果：

    Aumann, Robert J. Markets with a continuum of traders [连续统多个交易人的市场]. Econometrica. January–April 1964, 32 (1–2): 39–50. JSTOR 1913732. MR 0172689. doi:10.2307/1913732 （英语）.

    Aumann, Robert J. Integrals of set-valued functions [集合值函数的积分]. Journal of Mathematical Analysis and Applications. August 1965, 12 (1): 1–12. MR 0185073. doi:10.1016/0022-247X(65)90049-1  （英语）.

46. 据Diewert (1982，第552页)所言，Wold (1943b，第243页)及Wold & Juréen (1953，第146页)已于较早前讨论过取非凸偏好的凸包。

47. Starr & Stinchcombe (1999，第217–218页): Starr, R. M.; Stinchcombe, M. B. Exchange in a network of trading posts. Chichilnisky, Graciela (编). Markets, information and uncertainty: Essays in economic theory in honor of Kenneth J. Arrow [市场、资讯、不确定性：致敬肯尼斯·阿罗的经济理论论文]. Cambridge, UK: Cambridge University Press. 1999: 217–234. ISBN 978-0-521-08288-4. doi:10.2277/0521553555 （英语）.
48. Arrow & Hahn (1980，第169–182页)、Starr (1969，第27–33页)
49. Green & Heller (1981，第44页)
50. Guesnerie (1989，第99页)
51. Mas-Colell (1987)
52. Varian (1992，第393–394页): Varian, Hal R. 21.2 Convexity and size [21.2节：凸性与大小]. Microeconomic Analysis [微观经济分析] 3rd. W. W. Norton & Company. 1992. ISBN 978-0-393-95735-8. MR 1036734 （英语）.

    Mas-Colell, Whinston & Green (1995，第627–630页): Mas-Colell, Andreu; Whinston, Michael D.; Green, Jerry R. 17.1 Large economies and nonconvexities [第17.1节：大经济体与非凸性]. Microeconomic theory [微观经济理论]. Oxford University Press. 1995. ISBN 978-0-19-507340-9 （英语）.

53. Arrow & Hahn (1980，第169–182页)

    Mas-Colell (1985，第52–55, 145–146, 152–153, and 274–275页): Mas-Colell, Andreu. 1.L Averages of sets [第1.L节：集合的平均]. The Theory of general economic equilibrium: A differentiable approach [一般经济均衡理论：可微分的进路]. Econometric Society monographs 9. Cambridge University Press. 1985. ISBN 0-521-26514-2. MR 1113262 （英语）.

    Hildenbrand (1974，第37, 115–116, 122, and 168页): Hildenbrand, Werner. Core and equilibria of a large economy [大经济体的核和均衡]. Princeton studies in mathematical economics 5. Princeton, N.J.: Princeton University Press. 1974: viii+251. ISBN 978-0-691-04189-6. MR 0389160 （英语）.

54. Starr (1997，第169页)，及

    Ellickson (1994，第xviii, 306–310, 312, 328–329, 347, and 352页): Ellickson, Bryan. Competitive equilibrium: Theory and applications [竞争均衡：理论及应用]. Cambridge University Press. 1994. ISBN 978-0-521-31988-1. doi:10.2277/0521319889 （英语）.

55. Laffont, Jean-Jacques. 3. Nonconvexities [第3章：非凸性]. Fundamentals of public economics [公共经济学基础]. MIT Press. 1988: 63–65 [2021-08-30]. ISBN 0-262-12127-1. （原始内容存档于2021-08-30） （英语）.
56. Salanié (2000，第112–113 and 107–115页): Salanié, Bernard. 7 Nonconvexities [第7章：非凸性]. Microeconomics of market failures [市场失灵的微观经济学] 1998年法文版Microéconomie: Les défaillances du marché (Economica, Paris)的英文翻译. Cambridge, Mass.: MIT Press. 2000: 107–125. ISBN 0-262-19443-0 （英语）.
57. Ichiishi (1983，第24–25页): Ichiishi, Tatsuro. Game theory for economic analysis [经济分析的赛局理论]. Economic theory, econometrics, and mathematical economics. New York: Academic Press, Inc. [Harcourt Brace Jovanovich, Publishers]. 1983: x+164. ISBN 0-12-370180-5. MR 0700688 （英语）.
58. Cassels (1981，第127 and 33–34页): Cassels, J. W. S. Appendix A Convex sets [附录A：凸集]. Economics for mathematicians [数学家的经济学]. London Mathematical Society lecture note series 62. Cambridge, UK: Cambridge University Press. 1981: xi+145. ISBN 0-521-28614-X. MR 0657578 （英语）.
59. Aubin (2007，第458–476页): Aubin, Jean-Pierre. 14.2 Duality in the case of non-convex integral criterion and constraints (especially 14.2.3 The Shapley–Folkman theorem, pages 463–465) [第14.2节：非凸积分准则和限制下的对偶性（尤其第14.2.3小节：沙普利-福克曼定理，pp. 463–465]. Mathematical methods of game and economic theory [赛局与经济理论的数学方法] 连新序言，重印1982年North-Holland修订英文版. Mineola, N.Y.: Dover Publications, Inc. 2007: xxxii+616. ISBN 978-0-486-46265-3. MR 2449499 （英语）.
60. Carter (2001，第93–94, 143, 318–319, 375–377, and 416页)
61. Trockel (1984，第30页): Trockel, Walter. Market demand: An analysis of large economies with nonconvex preferences [市场需求：分析非凸偏好的大经济体]. Lecture Notes in Economics and Mathematical Systems 223. Berlin: Springer-Verlag. 1984: viii+205. ISBN 3-540-12881-6. MR 0737006 （英语）.
62. Bertsekas (1999，第496页): Bertsekas, Dimitri P. 5.1.6 Separable problems and their geometry [第5.1.6小节：可分问题及其几何]. Nonlinear Programming [非线性规划] Second. Cambridge, Mass.: Athena Scientific. 1999: 494–498. ISBN 1-886529-00-0 （英语）.
63. Rockafellar (1997，第23页)
64. 某集合内的序列的极限，必在该集合的闭包中，即最小而包含原集合的闭集。两个闭集的闵氏和不必闭，故若以${\displaystyle \mathrm {Cl} }$表示闭包，则虽然有包含关系

    ${\displaystyle \mathrm {Cl} (P)+\mathrm {Cl} (Q)\subseteq \mathrm {Cl} (P+Q),}$

    但可能为严格包含（左右不必相等）。据Rockafellar (1997，第49及75页)，即使两个被加项各已是闭凸集，仍可能是严格包含。若要使闵氏和变成闭，则要取其闭包，即要添加所有收敛序列的极限。
65. Lemaréchal (1973，第38页): Lemaréchal, Claude. Utilisation de la dualité dans les problémes non convexes [在非凸问题使用对偶] (报告). Domaine de Voluceau, Rocquencourt, Le Chesnay, France: IRIA（现INRIA）, Laboratoire de recherche en informatique et automatique: 41. April 1973 （法语）. |issue=被忽略 (帮助) 勒马雷沙尔的实验，日后有下列论文讨论：

    Aardal (1995，第2–3页): Aardal, Karen. Optima interview Claude Lemaréchal [Optima访问克劳德·勒马雷沙尔] (PDF). Optima: Mathematical Programming Society Newsletter. March 1995, 45: 2–4 [2 February 2011]. （原始内容存档 (PDF)于2021-09-09） （英语）.

    Hiriart-Urruty & Lemaréchal (1993，第143–145, 151, 153, and 156页): Hiriart-Urruty, Jean-Baptiste; Lemaréchal, Claude. XII Abstract duality for practitioners [第十二章：实践用的抽象对偶性]. Convex analysis and minimization algorithms, Volume II: Advanced theory and bundle methods [凸分析和最小化算法，第二卷：进阶理论及束法]. Grundlehren der Mathematischen Wissenschaften [数理科学的基本原理] 306. Berlin: Springer-Verlag. 1993: 136–193 （及pp. 334–335所列的文献附注）. ISBN 3-540-56852-2. MR 1295240 （英语）.

66. Ekeland, Ivar. Une estimation a priori en programmation non convexe [非凸规划中的先验估计]. Comptes Rendus Hebdomadaires des Séances de l'Académie des Sciences [（法国）科学院周刊]. Séries A et B. 1974, 279: 149–151. ISSN 0151-0509. MR 0395844 （法语）.
67. Aubin & Ekeland (1976，第226, 233, 235, 238, and 241页): Aubin, J. P.; Ekeland, I. Estimates of the duality gap in nonconvex optimization [估计非凸优化的对偶间隙]. Mathematics of Operations Research. 1976, 1 (3): 225–245. JSTOR 3689565. MR 0449695. doi:10.1287/moor.1.3.225 （英语）.

    Aubin & Ekeland (1976)及Ekeland (1999，第362–364页)也考虑非凸最小值问题的闭凸包，即对原问题的盖图（英语：epigraph (mathematics)）取闭凸包所得的新问题。Di Guglielmo推广到研究非凸多目标优化（英语：Multi-objective optimization）问题的拟凸闭包，即对目标函数的下水平集取凸闭包所得的问题：

    Di Guglielmo (1977，第287–288页): Di Guglielmo, F. Nonconvex duality in multiobjective optimization [多目标优化的非凸对偶]. Mathematics of Operations Research. 1977, 2 (3): 285–291. JSTOR 3689518. MR 0484418. doi:10.1287/moor.2.3.285 （英语）.

68. Schneider & Weil (2008，第45页): Schneider, Rolf; Weil, Wolfgang. Stochastic and integral geometry [随机与积分几何]. Probability and its applications. Springer. 2008. ISBN 978-3-540-78858-4. MR 2455326. doi:10.1007/978-3-540-78859-1 （英语）.
69. Cassels (1975，第433–434页): Cassels, J. W. S. Measures of the non-convexity of sets and the Shapley–Folkman–Starr theorem [集合的非凸度与沙普利-福克曼-斯塔定理]. Mathematical Proceedings of the Cambridge Philosophical Society. 1975, 78 (3): 433–436. MR 0385711. doi:10.1017/S0305004100051884 （英语）.
70. Molchanov (2005，第195–198, 218, 232, 237–238 and 407页): Molchanov, Ilya. 3 Minkowski addition [第3章：闵可夫斯基加法]. Theory of random sets [论随机集]. Probability and its applications. London: Springer-Verlag London Ltd. 2005: 194–240. ISBN 978-1-84996-949-9. MR 2132405. doi:10.1007/1-84628-150-4 （英语）.
71. Puri & Ralescu (1985，第154–155页): Puri, Madan L.; Ralescu, Dan A. Limit theorems for random compact sets in Banach space [巴拿赫空间随机紧集的极限定理]. Mathematical Proceedings of the Cambridge Philosophical Society. 1985, 97 (1): 151–158. Bibcode:1985MPCPS..97..151P. MR 0764504. doi:10.1017/S0305004100062691 （英语）.
72. Weil (1982，第203, and 205–206页): Weil, Wolfgang. An application of the central limit theorem for Banach-space–valued random variables to the theory of random sets [取值于巴拿赫空间随机变量的中央极限定理，应用于随机集理论]. Zeitschrift für Wahrscheinlichkeitstheorie und Verwandte Gebiete [概率论与相关领域期刊]. 1982, 60 (2): 203–208. MR 0663901. doi:10.1007/BF00531823 （英语）.
73. Cerf (1999，第243–244页): Cerf, Raphaël. Large deviations for sums of i.i.d. random compact sets [独立同分布随机紧集之和的大离差]. Proceedings of the American Mathematical Society. 1999, 127 (8): 2431–2436. MR 1487361. doi:10.1090/S0002-9939-99-04788-7  （英语）. 瑟夫（Cerf）用到 Puri & Ralescu (1985，第154–155页)应用沙普利-福克曼引理的结果。
74. Ruzsa (1997，第345页): Ruzsa, Imre Z. The Brunn–Minkowski inequality and nonconvex sets [布伦-闵可夫斯基不等式与非凸集]. Geometriae Dedicata. 1997, 67 (3): 337–348. MR 1475877. doi:10.1023/A:1004958110076 （英语）.
75. Tardella (1990，第478–479页): Tardella, Fabio. A new proof of the Lyapunov convexity theorem [李亚普诺夫凸性定理的新证]. SIAM Journal on Control and Optimization. 1990, 28 (2): 478–481. MR 1040471. doi:10.1137/0328026 （英语）.
76. Vind (1964，第168 and 175页): Vind, Karl. Edgeworth-allocations in an exchange economy with many traders [多交易者交易经济体中的埃奇沃思分配]. International Economic Review. May 1964, 5 (2): 165–77. JSTOR 2525560. doi:10.2307/2525560 （英语）. 1983年诺贝尔奖得主杰拉德·德布鲁有注意维恩（Vind）的论文。Debreu (1991，第4页)写道：

    凸集的概念（即该集合包含连接其任意两点的线段），已经多次成为1964年以前经济理论的核心。引入积分理论研究经济竞争后，得以新眼光看待此事：若经济体的每个参与者，对应商品空间的某个任意集合，而又对一族不重要的参与者取平均，则所得的集合必然为凸。[德布鲁附注：“此为A. A. 李亚普诺夫的定理的直接推论，参见Vind (1964)。”] 但⋯⋯诸价格函数⋯⋯可以因平均而产生的凸性解释。商品空间中，对一族不重要参与者加总可以得到凸性，是经济理论⋯⋯从积分理论得来的观察。 [删节后译文]

    Debreu, Gérard. The Mathematization of economic theory [经济理论的数学化]. The American Economic Review. March 1991, 81 (Presidential address delivered at the 103rd meeting of the American Economic Association, 29 December 1990, Washington, DC): 1–7. JSTOR 2006785 （英语）.

77. Artstein (1980，第172–183页) Artstein (1980)在致敬2008年诺贝尔经济学奖得主罗伯特·奥曼的论文集（英语：Festschrift）重新出版：Artstein, Zvi. 22 Discrete and continuous bang–bang and facial spaces or: Look for the extreme points [第22篇：离散与连续砰砰及面空间，又或：找极值点]. Hart, Sergiu; Neyman, Abraham (编). Game and economic theory: Selected contributions in honor of Robert J. Aumann [赛局与经济理论：致敬罗伯特·J. 奥曼的文选]. Ann Arbor, Mich.: University of Michigan Press. 1995: 449–462. ISBN 0-472-10673-2. （原始内容存档于24 May 2011） （英语）.
78. Mas-Colell (1978，第210页): Mas-Colell, Andreu. A note on the core equivalence theorem: How many blocking coalitions are there? [记核等价定理：有多少个联盟在阻碍？]. Journal of Mathematical Economics. 1978, 5 (3): 207–215. MR 0514468. doi:10.1016/0304-4068(78)90010-1 （英语）.

参考文献

• Anderson, Robert M. Nonconvex preferences and approximate equilibria [非凸偏好与近似均衡] (PDF). Economics 201B: Economic Theory (Handouts) [经济学201B: 经济理论（课堂讲义）]. Robert M. Anderson's homepage (Berkeley, Calif.). 14 March 2005: 1–5 [1 January 2011]. （原始内容存档 (PDF)于2012-03-10） （英语）.
• Arrow, Kenneth J.; Hahn, Frank H. General competitive analysis [一般竞争分析]. Advanced Textbooks in Economics 12 San Francisco, CA: Holden-Day, Inc. Mathematical Economics Texts 6之重印版. Amsterdam: North-Holland. 1980 [1971]. ISBN 0-444-85497-5. MR 0439057 （英语）.
• Artstein, Zvi. Discrete and continuous bang-bang and facial spaces, or: Look for the extreme points [离散与连续砰砰及面空间，又或：找极值点]. SIAM Review. 1980, 22 (2): 172–185. JSTOR 2029960. MR 0564562. doi:10.1137/1022026 （英语）.
• Carter, Michael. Foundations of mathematical economics [数理经济学基础]. Cambridge, Mass.: MIT Press. 2001: xx+649. ISBN 0-262-53192-5. MR 1865841. (作者网站有习题题解). （原始内容存档于15 September 2006） （英语）.
• Diewert, W. E. 12 Duality approaches to microeconomic theory [第12章：微观经济学的对偶进路]. Arrow, Kenneth Joseph; Intriligator, Michael D (编). Handbook of mathematical economics, Volume II [数理经济学手册・卷二]. Handbooks in Economics 1. Amsterdam: North-Holland Publishing Co. 1982: 535–599 [2021-08-31]. ISBN 978-0-444-86127-6. MR 0648778. doi:10.1016/S1573-4382(82)02007-4. （原始内容存档于2012-12-10） （英语）.
• Ekeland, Ivar. Appendix I: An a priori estimate in convex programming [附录一：凸规划的先验估计]. Ekeland, Ivar; Temam, Roger (编). Convex analysis and variational problems [凸分析与变分问题]. Classics in Applied Mathematics 28 Corrected reprinting of the North-Holland. Philadelphia: Society for Industrial and Applied Mathematics (SIAM). 1999: 357–373 [1976]. ISBN 0-89871-450-8. MR 1727362 （英语）.
• Green, Jerry; Heller, Walter P. 1 Mathematical analysis and convexity with applications to economics [第1章：数学分析与凸性，及在经济学之应用]. Arrow, Kenneth Joseph; Intriligator, Michael D (编). Handbook of mathematical economics, Volume I. Handbooks in Economics 1. Amsterdam: North-Holland Publishing Co. 1981: 15–52. ISBN 0-444-86126-2. MR 0634800. doi:10.1016/S1573-4382(81)01005-9 （英语）.
• Guesnerie, Roger. First-best allocation of resources with nonconvexities in production [生产非凸的资源之最优分配]. Cornet, Bernard; Tulkens, Henry (编). Contributions to Operations Research and Economics: The twentieth anniversary of CORE (Papers from the symposium held in Louvain-la-Neuve, January 1987) [运筹学与经济学投稿：二十周年（[[新鲁汶]]1987年举办讨论会的论文集）]. Cambridge, Mass.: MIT Press. 1989: 99–143. ISBN 0-262-03149-3. MR 1104662 （英语）. 网址－维基内链冲突 (帮助)
• Howe, Roger. On the tendency toward convexity of the vector sum of sets [论集合的向量和趋向凸] (PDF) (报告) 538. New Haven, Conn.: Cowles Foundation for Research in Economics, Yale University. 3 November 1979 [1 January 2011]. （原始内容存档 (PDF)于2011-08-07） （英语）.
• Mas-Colell, A. Non-convexity [非凸性]. Eatwell, John; Milgate, Murray; Newman, Peter (编). The new Palgrave: A dictionary of economics [新帕尔格雷夫经济学词典] first. Palgrave Macmillan. 1987: 653–661 [2021-08-31]. ISBN 9780333786765. doi:10.1057/9780230226203.3173. (Mas-Colell个人主页的PDF档). （原始内容存档于2017-11-21） （英语）.
• Rockafellar, R. Tyrrell. Convex analysis [凸分析]. Princeton Landmarks in Mathematics. Princeton, N.J.: Princeton University Press. 1997: xviii+451. ISBN 0-691-01586-4. MR 1451876 （英语）. 1970年(MR274683) Princeton Mathematical Series 28的重印版。
• Schneider, Rolf. Convex bodies: The Brunn–Minkowski theory [凸体：布伦-闵可夫斯基理论]. Encyclopedia of Mathematics and its Applications 44. Cambridge, UK: Cambridge University Press. 1993: xiv+490. ISBN 0-521-35220-7. MR 1216521 （英语）.
• Starr, Ross M. Quasi-equilibria in markets with non-convex preferences (Appendix 2: The Shapley–Folkman theorem, pp. 35–37) [有非凸偏好的市场的准均衡（附录2:沙普利-福克曼定理，pp. 35–37）]. Econometrica. 1969, 37 (1): 25–38. JSTOR 1909201. doi:10.2307/1909201 （英语）.
• Starr, Ross M. 8 Convex sets, separation theorems, and non-convex sets in R^N (new chapters 22 and 25–26 in (2011) second ed.) [第8章：R^N中的凸集、分离定理、非凸集（及2011年第二版新增的第22、25、26诸章）]. General equilibrium theory: An introduction [一般均衡理论：导论] First. Cambridge, UK: Cambridge University Press. 1997: xxiii+250. ISBN 0-521-56473-5. MR 1462618 （英语）.
• Starr, Ross M. Shapley–Folkman theorem [沙普利-福克曼定理]. Durlauf, Steven N.; Blume, Lawrence E (编). The new Palgrave dictionary of economics [新帕尔格雷夫经济学词典] Second. Palgrave Macmillan. 2008: 317–318 (1st ed.) [2021-08-31]. ISBN 978-0-333-78676-5. doi:10.1057/9780230226203.1518. （原始内容存档于2017-03-16） （英语）.

外部链结

• Anderson, Robert M. Nonconvex preferences and approximate equilibria [非凸偏好与近似均衡] (PDF). Economics 201B: Economic Theory (Handouts) [经济学201B: 经济理论（课堂讲义）]. Robert M. Anderson's homepage (Berkeley, Calif.). 14 March 2005: 1–5 [1 January 2011]. （原始内容存档 (PDF)于2012-03-10）.
• Starr, Ross M. 8 Convex sets, separation theorems, and non-convex sets in R^N (Section 8.2.3 Measuring non-convexity, the Shapley–Folkman theorem) [第8章：R^N中的凸集、分离定理、非凸集（第8.2.3小节：量度非凸性、沙普利-福克曼定理）] (PDF). General equilibrium theory: An introduction [一般均衡理论：导论]. September 2009: 3–6 [2021-08-31]. ISBN 9781139174749. MR 1462618. doi:10.1017/CBO9781139174749. （第二版的书稿，来自斯塔在加州大学圣地亚哥分校经济学系的课程）. （原始内容存档 (PDF)于2010-07-01） （英语）.
• Starr, Ross M. Shapley–Folkman theorem [沙普利-福克曼定理]. Durlauf, Steven N.; Blume, Lawrence E (编). The new Palgrave dictionary of economics [新帕尔格雷夫经济学词典] Second. Palgrave Macmillan. 2008: 317–318 (1st ed.) [2021-08-31]. ISBN 978-0-333-78676-5. doi:10.1057/9780230226203.1518. （原始内容存档于2017-03-16） （英语）.