希爾伯特轉換

在数学和信号处理中，希尔伯特变换（英語：Hilbert transform）是一个对函数 u(t) 产生定义域相同的函数 H(u)(t) 的线性算子。

希尔伯特变换在信号处理中很重要，能够导出信号 u(t) 的解析表示。这就意味着将实信号 u(t) 拓展到复平面，使其满足柯西－黎曼方程。 例如，希尔伯特变换引出了傅里叶分析中给定函数的调和共轭，也就是调和分析。等价地说，它是奇异积分算子与傅里叶乘子（英语：Multiplier (Fourier analysis)）的一个例子。

希尔伯特变换最初只对周期函数（也就是圆上的函数）有定义，在这种情况下它就是与希尔伯特核的卷积。然而更常见的情况下，对于定义在实直线 R（上半平面的边界）上的函数，希尔伯特变换是指与柯西核卷积。希尔伯特变换与帕利-维纳定理（英语：Paley–Wiener theorem）有着密切的联系，帕利-维纳定理是将上半平面内的全纯函数与实直线上的函数的傅里叶变换相联系起来的另一种结果。

希爾伯特轉換是以大卫·希尔伯特來命名的，他首先引入了该算子来解决全纯函数的黎曼–希尔伯特问题的一个特殊情况。

希爾伯特轉換結果（紅色）與原來的訊號——方波（藍色）

定義

${\displaystyle u}$ 的希尔伯特变换可以认为是 ${\displaystyle u(t)}$ 与函数 ${\displaystyle h(t)={\frac {1}{\pi t}}:=\delta (jt)}$ 的卷积。由于 ${\displaystyle h(t)}$ 是不可积的，定义卷积的积分不收敛。因而希尔伯特变换是使用柯西主值（这里记为${\displaystyle p.v.}$）定义的。准确说来，函数（或信号） ${\displaystyle u(t)}$ 的希尔伯特变换是：

${\displaystyle H(u)(t)=\operatorname {p.v.} \int _{-\infty }^{\infty }u(\tau )h(t-\tau )\,d\tau ={\frac {1}{\pi }}\ \operatorname {p.v.} \int _{-\infty }^{\infty }{\frac {u(\tau )}{t-\tau }}\,d\tau }$

假设此积分作为主值存在。这就是 u 与缓增分布 p.v. 1/πt 的卷积（由于Schwartz (1950)；参见Pandey (1996，Chapter 3)）。另外，通过改变变量，主值积分可以显式地(Zygmund 1968，§XVI.1)写为：

${\displaystyle H(u)(t)={\frac {2}{\pi }}\lim _{\varepsilon \rightarrow 0}\int _{\varepsilon }^{\infty }{\frac {u(t+\tau )-u(t-\tau )}{2\tau }}\,d\tau .}$

若希尔伯特变换接连用在函数 u 上两次，结果就是负 u：

${\displaystyle H(H(u))(t)=u(-t)}$

假设定义两次迭代的积分都收敛。特别地，逆变换是 −H。可以通过考虑 u(t) 的傅里叶变换的希尔伯特变换效应看出这一事实（参见下面的与傅里叶变换的关系）。

对上半平面的解析函数，希尔伯特变换描述了边界值的实部与虚部之间的关系。也就是说，如果 f(z) 是在 Im z > 0 平面内的解析函数，而 u(t) = Re f(t + 0·i )，假设希尔伯特变换存在，则 Im f(t + 0·i ) = H(u)(t) 取决于一个相加性常数。

頻率響應

希爾伯特轉換之頻率響應由傅立葉變換給出：

${\displaystyle H(\omega )=(-i\cdot \operatorname {sgn}(\omega ))\cdot {\mathcal {F}}\{h\}(\omega )\,}$  

其中

• ${\displaystyle {\mathcal {F}}}$是傅立葉變換，
• i (有時寫作j )是虛數單位，
• ${\displaystyle \omega \,}$是角頻率，以及
• ${\displaystyle \operatorname {sgn}(\omega )={\begin{cases}\ \ 1,&{\mbox{for }}\omega >0,\\\ \ 0,&{\mbox{for }}\omega =0,\\-1,&{\mbox{for }}\omega <0,\end{cases}}}$

即為符号函数。

既然：

${\displaystyle {\mathcal {F}}\{{\widehat {s}}\}(\omega )=H(\omega )\cdot {\mathcal {F}}\{s\}(\omega )}$,

希爾伯特轉換會將負頻率成分${\displaystyle s(t)\,}$偏移+90°，而正頻率成分偏移−90°。

反（逆）希爾伯特轉換

我們也注意到：${\displaystyle H^{2}(\omega )=1^{-}\,}$。因此將上面方程式乘上${\displaystyle -H(\omega )\,}$，可得到：

${\displaystyle {\mathcal {F}}\{s\}(\omega )=-H(\omega )\cdot {\mathcal {F}}\{{\widehat {s}}\}(\omega )}$

從中，可以看出反（逆）希爾伯特轉換

${\displaystyle s(t)=(h*h*h*{\widehat {s}})(t)={\mathcal {H}}^{3}\{{\widehat {s}}\}(t).\,}$

希爾伯特轉換表格

訊號 ${\displaystyle u(t)\,}$	希爾伯特轉換[fn 1] ${\displaystyle H(u)(t)}$
${\displaystyle \sin(t)}$ [fn 2]	${\displaystyle -\cos(t)}$
${\displaystyle \cos(t)}$ [fn 2]	${\displaystyle \sin(t)\,}$
${\displaystyle \exp \left(it\right)}$	${\displaystyle -i\exp \left(it\right)}$
${\displaystyle \exp \left(-it\right)}$	${\displaystyle i\exp \left(-it\right)}$
${\displaystyle 1 \over t^{2}+1}$	${\displaystyle t \over t^{2}+1}$
${\displaystyle e^{-t^{2}}}$	${\displaystyle 2\pi ^{-1/2}F(t)}$ 参见道森积分
Sinc函数 ${\displaystyle \sin(t) \over t}$	${\displaystyle 1-\cos(t) \over t}$
矩形函数 ${\displaystyle \sqcap (t)}$	${\displaystyle {1 \over \pi }\log \left|{t+{1 \over 2} \over t-{1 \over 2}}\right|}$
狄拉克δ函数 ${\displaystyle \delta (t)\,}$	${\displaystyle {1 \over \pi t}:=\delta (jt)}$
指示函数 ${\displaystyle \chi _{[a,b]}(t)\,}$	${\displaystyle {\frac {1}{\pi }}\log \left\vert {\frac {t-a}{t-b}}\right\vert }$

Notes

1. Some authors (e.g., Bracewell) use our −H as their definition of the forward transform. A consequence is that the right column of this table would be negated.
2. The Hilbert transform of the sin and cos functions can be defined in a distributional sense, if there is a concern that the integral defining them is otherwise conditionally convergent. In the periodic setting this result holds without any difficulty.

常數之希爾伯特轉換為零

特性

邊界

若 1<p<∞，則 L^p(R)之希爾伯特轉換為一有界算子，表示存在一常數C_p使得

${\displaystyle \|Hu\|_{p}\leq C_{p}\|u\|_{p}}$

對所有 u∈L^p(R)。這個定理由Riesz (1928，VII)所推得；請一併參見Titchmarsh (1948，Theorem 101)。 最佳常數C_p可由下列算式得到:

${\displaystyle C_{p}={\begin{cases}\tan {\frac {\pi }{2p}}&{\text{for }}1<p\leq 2\\\cot {\frac {\pi }{2p}}&{\text{for }}2<p<\infty \end{cases}}}$

這個結果由(Pichorides 1972)所推得；請一併參見Grafakos (2004，Remark 4.1.8)。上述最佳常數計算方式應用在週期性希爾伯特轉換一樣成立。

希爾伯特轉換的邊界指的是 L^p(R) 對稱級數運算子對於在 L^p(R) 之中 f 的收斂

${\displaystyle S_{R}f=\int _{-R}^{R}{\hat {f}}({\xi })e^{2\pi ix\xi }\,d\xi }$

請參見(Duoandikoetxea 2000，第59頁)。

反自伴性

希爾伯特轉換為一反自伴算子，連結 L^p(R) 與其對偶空間 L^q(R)，其中 p 和 q 為 赫爾德共軛且 1 < p,q < ∞. 以符號表示

${\displaystyle \langle Hu,v\rangle =\langle u,-Hv\rangle }$

對 u ∈ L^p(R) 且 v ∈ L^q(R) (Titchmarsh 1948，Theorem 102).

逆轉換

希爾伯特轉換為一反-對合 (Titchmarsh 1948，第120頁)，意即

${\displaystyle H(H(u))=-u}$

假定每一轉換皆完整定義過。由於 H 保存了 L^p(R)空間，這特別代表希爾伯特轉換在 L^p(R) 上是可逆的，且

${\displaystyle H^{-1}=-H}$

微分

正式上，一個式子其希爾伯特轉換的微分即為其微分的希爾伯特轉換，意即這兩者是可以交換的線性算子

${\displaystyle H\left({\frac {du}{dt}}\right)={\frac {d}{dt}}H(u)}$

此一特性亦可迭代

${\displaystyle H\left({\frac {d^{k}u}{dt^{k}}}\right)={\frac {d^{k}}{dt^{k}}}H(u)}$

給定 u 以及其前k次微分皆屬於L^p(R) (Pandey 1996，§3.3)空間，此項論述為嚴格成立。在頻域上可以輕易驗證這件事情，由於微分在頻域上即為與 ω 之乘積。

旋積

希爾伯特轉換可表示為與一缓增分布之旋積 (Duistermaat & Kolk 2010，第211頁)

${\displaystyle h(t)={\text{p.v. }}{\frac {1}{\pi t}}}$

因此可如此表示

${\displaystyle H(u)=h*u}$

然而，事前此特性可能只有對緊支撐之分布 u定義。由於緊支撐函數在 L^p 上是稠密的，因此此項特性可能嚴格成立。另一角度來看，也可使用 h(t) 其微分之特性來證明

${\displaystyle H(u)(t)={\frac {d}{dt}}\left({\frac {1}{\pi }}(u*\log |\cdot |)(t)\right)}$

在大部分的用途，希爾伯特轉換可被視為是一旋積。舉例而言，旋積與希爾伯特轉換具備下列可交換的特性

${\displaystyle H(u*v)=H(u)*v=u*H(v)}$

若 u 和 v 為緊支撐分布，則此項論述嚴格成立，在這個狀況下

${\displaystyle h*(u*v)=(h*u)*v=u*(h*v)}$

不變性

希爾伯特轉換在空間 L²(R) 上有下列特性

• 可與算子 T_aƒ(x) = ƒ(x + a) 交換，對所有實數 a
• 可與算子 M_λƒ(x) = ƒ(λx) 交換，對所有 λ > 0
• 可與鏡射 Rƒ(x) = ƒ(−x) 反交換

實際上，有更大一部分的算子可與希爾伯特轉換交換。群組 SL(2,R) 由幺正算符 U_g 可在空間 L²(R) 上由以下式子表示

${\displaystyle \displaystyle {U_{g}^{-1}f(x)=(cx+d)^{-1}f\left({ax+b \over cx+d}\right),\,\,\,g={\begin{pmatrix}a&b\\c&d\end{pmatrix}}}}$

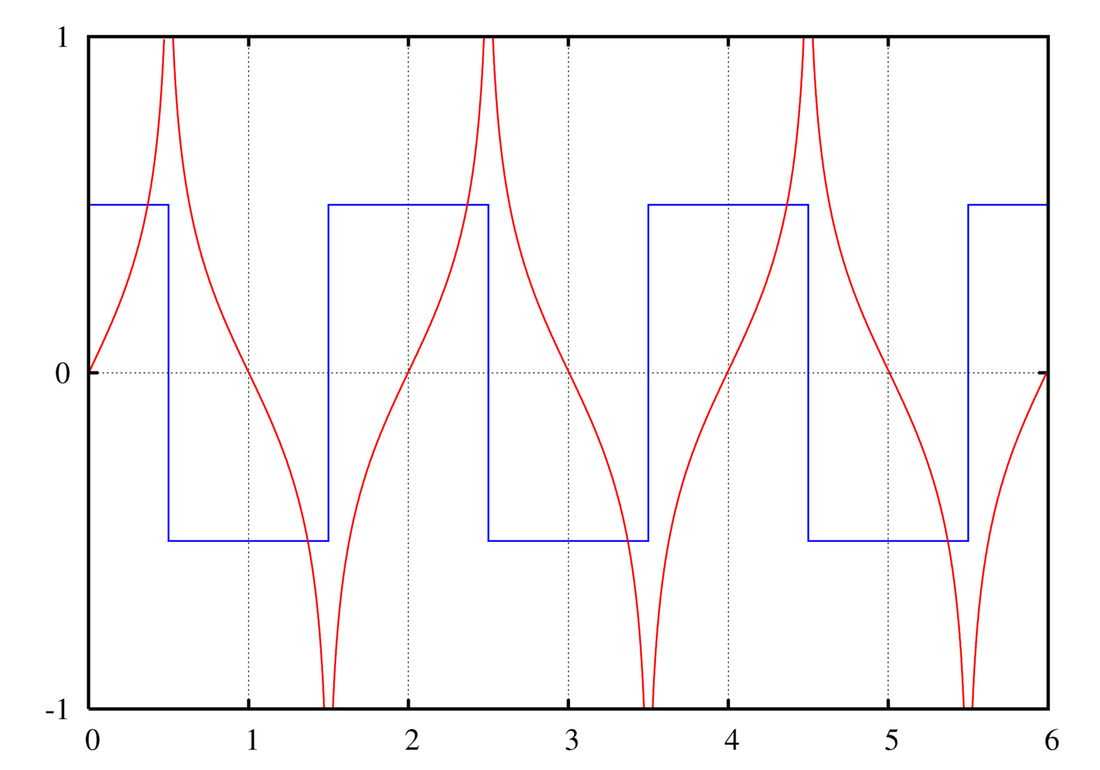
希爾伯特轉換例子

注意：有些作者，例如Bracewell，將我們的${\displaystyle -{\mathcal {H}}}$當作其正轉換的定義。這樣的結果就是下表右行要乘上一個負號。

離散希爾伯特轉換

圖 1: 頻寬被限制在95%奈奎斯特頻率之濾波器頻率響應

圖 2: 高通頻率響應之希爾伯特轉換濾波器

圖 3.

圖 4. cos(wt)函數之希爾伯特轉換為 sin(wt)。此圖顯示了sin(wt)函數與一個利用MATLAB函式庫 hilbert(·)計算之近似希爾伯特轉換的差異

對於一離散函數 u[n]，以及其 離散傅利葉轉換 函數 U(ω)，可推得其希爾伯特轉換為:

${\displaystyle H(u)[n]=\scriptstyle {DTFT}^{-1}\displaystyle \{U(\omega )\cdot \sigma _{H}(\omega )\}}$

其中

${\displaystyle \sigma _{H}(\omega )\ {\stackrel {\mathrm {def} }{=}}\ {\begin{cases}e^{+i\pi /2},&-\pi <\omega <0\\e^{-i\pi /2},&0<\omega <\pi \\0,&\omega =-\pi ,0,\pi \end{cases}}}$

此外，根據摺積定律，另一個相等的方程式為:

${\displaystyle H(u)[n]=u[n]*h[n]}$

其中

${\displaystyle h[n]\ {\stackrel {\mathrm {def} }{=}}\ \scriptstyle {DTFT}^{-1}{\big \{}\displaystyle \sigma _{H}(\omega ){\big \}}={\begin{cases}0,&{\mbox{for }}n{\mbox{ even}}\\{\frac {2}{\pi n}}&{\mbox{for }}n{\mbox{ odd}}\end{cases}}}$

當摺積經由數值運算後，一FIR 近似將取代h[n]，如 圖 1所示，可以見到頻率響應在通帶之兩端(0與奈奎斯特頻率)的陡降，形成一帶通濾波器。其中高頻部分可藉由一FIR濾波器回復，如 圖 2所示。然而實際上，一個經過適當取樣的 u[n] 序列在高頻部分已經不具有可用的分量。當脈衝響應持續越久，低頻部分也可以被回復。

用FIR近似h[n]的時候，交疊儲存法是一個對於很長的u[n] 序列做摺積運算的有效方法。有時候陣列FFT{h[n]}會被σ_H(ω)相對應之取樣序列所取代。如此將會有與週期疊加函數做摺積之效果:

${\displaystyle h_{N}[n]\ {\stackrel {\text{def}}{=}}\ \sum _{m=-\infty }^{\infty }h[n-mN]}$

圖 3比較了h_N[n]之半周期與一相同長度分量之h[n]。兩者之間之差異與兩者之長度皆不短於區段長度(N)之現象為失真的來源，且失真可經由增加區段長度與交疊參數來有效減少。

MATLAB中有一函數 hilbert(u,N)，此函數會回傳一複數序列，其中虛部序列為 u[n]之離散希爾伯特轉換近似，實部序列為原本輸入之序列，所以這樣的複數輸出等於是 u[n]的分析訊號。與前述類似， hilbert(u, N) 只使用來自 sgn(ω)分佈的取樣，因此是與 h_N[n] 的摺積。如前段所述，失真可藉由選擇比實際之u[n]序列更大的N與捨棄適當數量的輸出取樣來有效減少。圖 4為這種失真的一個例子。

相關條目

• 卷積
• 希爾伯特-黃轉換

參考文獻

• Bargmann, V., Irreducible unitary representations of the Lorentz group, Ann. of Math., 1947, 48 (3): 568–640, JSTOR 1969129, doi:10.2307/1969129
• Bedrosian, E., A Product Theorem for Hilbert Transforms (PDF), Rand Corporation Memorandum, December 1962, (RM-3439-PR) [2016-08-05], （原始内容存档 (PDF)于2021-02-25）
• Benedetto, John J. Harmonic analysis and applications. Boca Raton, FL: CRC Press. 1996. ISBN 0849378796.
• Bitsadze, A.V., Boundary value problems of analytic function theory, Hazewinkel, Michiel (编), 数学百科全书, Springer, 2001, ISBN 978-1-55608-010-4.
• Bracewell, R., The Fourier Transform and Its Applications 3rd, McGraw–Hill, 2000, ISBN 0-07-116043-4.
• Calderón, A.P.; Zygmund, A., On the existence of certain singular integrals, Acta Mathematica, 1952, 88 (1): 85–139, doi:10.1007/BF02392130.
• Carlson, Crilly, and Rutledge, Communication Systems 4th, 2002, ISBN 0-07-011127-8.
• Duoandikoetxea, J., Fourier Analysis, American Mathematical Society, 2000, ISBN 0-8218-2172-5.
• Duistermaat, J.J.; Kolk, J.A.C. Kolk, Distributions, Birkhäuser, 2010, ISBN 978-0-8176-4672-1, doi:10.1007/978-0-8176-4675-2.
• Duren, P., Theory of ${\displaystyle H^{p}}$-Spaces, New York: Academic Press, 1970.
• Fefferman, C., Characterizations of bounded mean oscillation, Bull. Amer. Math. Soc., 1971, 77 (4): 587–588, MR 0280994, doi:10.1090/S0002-9904-1971-12763-5.
• Fefferman, C.; Stein, E.M., H^p spaces of several variables, Acta Math., 1972, 129: 137–193, MR 0447953, doi:10.1007/BF02392215.
• Gel'fand, I.M.; Shilov, G.E., Generalized Functions, Vol. 2, Academic Press, 1967.
• Grafakos, Loukas, An Elementary Proof of the Square Summability of the Discrete Hilbert Transform, American Mathematical Monthly (Mathematical Association of America), 1994, 101 (5): 456–458, JSTOR 2974910, doi:10.2307/2974910.
• Grafakos, Loukas, Classical and Modern Fourier Analysis, Pearson Education, Inc.: 253–257, 2004, ISBN 0-13-035399-X.
• Hardy, G. H.; Littlewood, J. E.; Polya, G., Inequalities, Cambridge: Cambridge University Press, 1952, ISBN 0-521-35880-9.
• Hilbert, David, Grundzüge einer allgemeinen Theorie der linearen Integralgleichungen, Chelsea Pub. Co., 1953
• Kak, Subhash, The discrete Hilbert transform, Proc. IEEE, 1970, 58: 585–586 .
• Kak, Subhash, Number theoretic Hilbert transform, Circuits Systems Signal Processing, 2014, 33: 2539–2548 .
• Khvedelidze, B.V., Hilbert transform, Hazewinkel, Michiel (编), 数学百科全书, Springer, 2001, ISBN 978-1-55608-010-4.
• King, Frederick W., Hilbert Transforms 2, Cambridge: Cambridge University Press: 453, 2009, ISBN 978-0-521-51720-1.
• Kress, Rainer, Linear Integral Equations, New York: Springer-Verlag: 91, 1989, ISBN 3-540-50616-0.
• Lang, Serge, SL(2,R), Graduate Texts in Mathematics 105, Springer-Verlag, 1985, ISBN 0-387-96198-4
• Pandey, J.N., The Hilbert transform of Schwartz distributions and applications, Wiley-Interscience, 1996, ISBN 0-471-03373-1
• Pichorides, S., On the best value of the constants in the theorems of Riesz, Zygmund, and Kolmogorov, Studia Mathematica, 1972, 44: 165–179
• Riesz, Marcel, Sur les fonctions conjuguées, Mathematische Zeitschrift, 1928, 27 (1): 218–244, doi:10.1007/BF01171098
• Rosenblum, Marvin; Rovnyak, James, Hardy classes and operator theory, Dover, 1997, ISBN 0-486-69536-0
• Schwartz, Laurent, Théorie des distributions, Paris: Hermann, 1950.
• Schreier, P.; Scharf, L., Statistical signal processing of complex-valued data: the theory of improper and noncircular signals, Cambridge University Press, 2010
• Stein, Elias, Singular integrals and differentiability properties of functions, Princeton University Press, 1970, ISBN 0-691-08079-8.
• Stein, Elias; Weiss, Guido, Introduction to Fourier Analysis on Euclidean Spaces, Princeton University Press, 1971, ISBN 0-691-08078-X.
• Sugiura, Mitsuo, Unitary Representations and Harmonic Analysis: An Introduction, North-Holland Mathematical Library 44 2nd, Elsevier, 1990, ISBN 0444885935
• Titchmarsh, E, Reciprocal formulae involving series and integrals, Mathematische Zeitschrift, 1926, 25 (1): 321–347, doi:10.1007/BF01283842.
• Titchmarsh, E, Introduction to the theory of Fourier integrals 2nd, Oxford University: Clarendon Press, 19481986, ISBN 978-0-8284-0324-5.
• Zygmund, Antoni, Trigonometric series 2nd, Cambridge University Press, 19681988, ISBN 978-0-521-35885-9.

外部連結

• The Discrete Hilbert Transform; A Brief Tutorial_w236 （页面存档备份，存于互联网档案馆）
• Derivation of the boundedness of the Hilbert transform （页面存档备份，存于互联网档案馆）
• Mathworld Hilbert transform （页面存档备份，存于互联网档案馆） — Contains a table of transforms
• Analytic Signals and Hilbert Transform Filters
• 埃里克·韦斯坦因. Titchmarsh theorem. MathWorld.
• Mathias Johansson, "The Hilbert transform" a student level summary to Hilbert transformation. ^{[失效連結]} (via www.archive.org)
• GS256 Lecture 3: Hilbert Transformation, an entry level introduction to Hilbert transformation. ^{[失效連結]} (via www.archive.org)