三角形数

一定数目的点或圆在等距离的排列下可以形成一个等边三角形，这样的数被称为三角形数。比如10个点可以组成一个等边三角形，因此10是一个三角形数：

提示：此条目页的主题不是三角函数。

三角形数

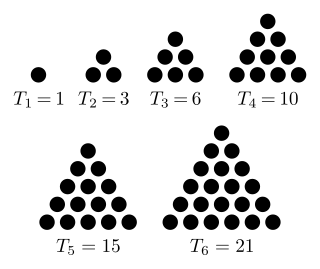
头30个三角形数是1, 3, 6, 10, 15, 21, 28, 36, 45, 55, 66, 78, 91, 105, 120, 136, 153, 171, 190, 210, 231, 253, 276, 300, 325, 351, 378, 406, 435, 465, ...（OEIS数列A000217）。

三角数的二倍的平方根取整，是这个三角数的序数。

性质

• 第n个三角形数的公式是${\displaystyle {\frac {n(n+1)}{2}}}$。
• 第n个三角形数是从1开始的n个自然数的和。
• 所有大于3的三角形数都不是质数。
• 除了0，1，3，21，55以外，三角形数不可能是费波那契数。^{[来源请求]}
• 开始的n个立方数的和是第n个三角形数的平方（举例：1 + 8 + 27 + 64 = 100 = 10²）
• 所有三角形数的倒数之和是2。
• 任何三角形数乘以8再加1是一个平方数。
• 三角数的个位数字不可能是2、4、7、9，数字根不可能是2、4、5、7、8。
• 一部分三角形数（3、10、21、36、55、78……）可以用以下这个公式来表示：${\displaystyle n*(2n+1)}$；而剩下的另一部分（1、6、15、28、45、66……）则可以用${\displaystyle n*(2n-1)}$来表示。
• 一种检验正整数x是否三角形数的方法，是计算：

  ${\displaystyle n={\frac {{\sqrt {8x+1}}-1}{2}}.}$

  如果n是整数，那么x就是第n个三角形数。如果n不是整数，那么x不是三角形数。这个检验法是基于恒等式${\displaystyle 8T_{n}+1=S_{2n+1}.}$

特殊的三角形数

• 55、5,050、500,500、50,005,000……都是三角形数。
• 第11个三角形数（66）、第1111个三角形数（617,716）、第111,111个三角形数（6,172,882,716）、第11,111,111个三角形数（61,728,399,382,716）都是回文式的三角形数，但第111个、第11,111个和第1,111,111个三角形数不是。
• 同时为三角形数及普洛尼克数的数（不定方程${\displaystyle x(x+1)={\frac {y(y+1)}{2}}}$）：最小的几个为0, 6, 210, 7140, 242556, 8239770,……^[1][2]，对应的${\displaystyle x}$值分别为0, 2, 14, 84, 492, 2870,……（OEIS数列A053141），对应的${\displaystyle y}$值分别为0, 3, 20, 119, 696, 4059,……（OEIS数列A001652）。

它与其他数的关系

• 是否在相继出现的三角形数之间至少存在一个素数，在9000000以下的数目是正确的。
• 四面体数是三角形数在立体的推广。
• 两个相继的三角形数之和是平方数。
• 三角平方数是同时为三角形数和平方数的数。
• 三角形数属于一种多边形数。
• 所有偶完美数都是三角形数。
• 任何自然数是最多三个三角形数的和。高斯发现了这个规律，他在1796年7月10日在日记中写道：EYPHKA! num = Δ + Δ + Δ

外部链接

• Hazewinkel, Michiel (编), Arithmetic series, 数学百科全书, Springer, 2001, ISBN 978-1-55608-010-4
• Triangular numbers（页面存档备份，存于互联网档案馆） at cut-the-knot
• There exist triangular numbers that are also square（页面存档备份，存于互联网档案馆） at cut-the-knot
• 埃里克·韦斯坦因. Triangular Number. MathWorld.
• Triangular numbers via 12 days of Christmas by Vi Hart
• Hypertetrahedral Polytopic Roots by Rob Hubbard, including the generalisation to triangular cube roots, some higher dimensions, and some approximate formulae

参考资料

1. Sloane, N.J.A. (编). Sequence A029549 (Triangular numbers that are also pronic numbers). The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
2. pronic numbers. NUMBERS APLENTY. [2021-02-05]. （原始内容存档于2021-02-25）.