外正割

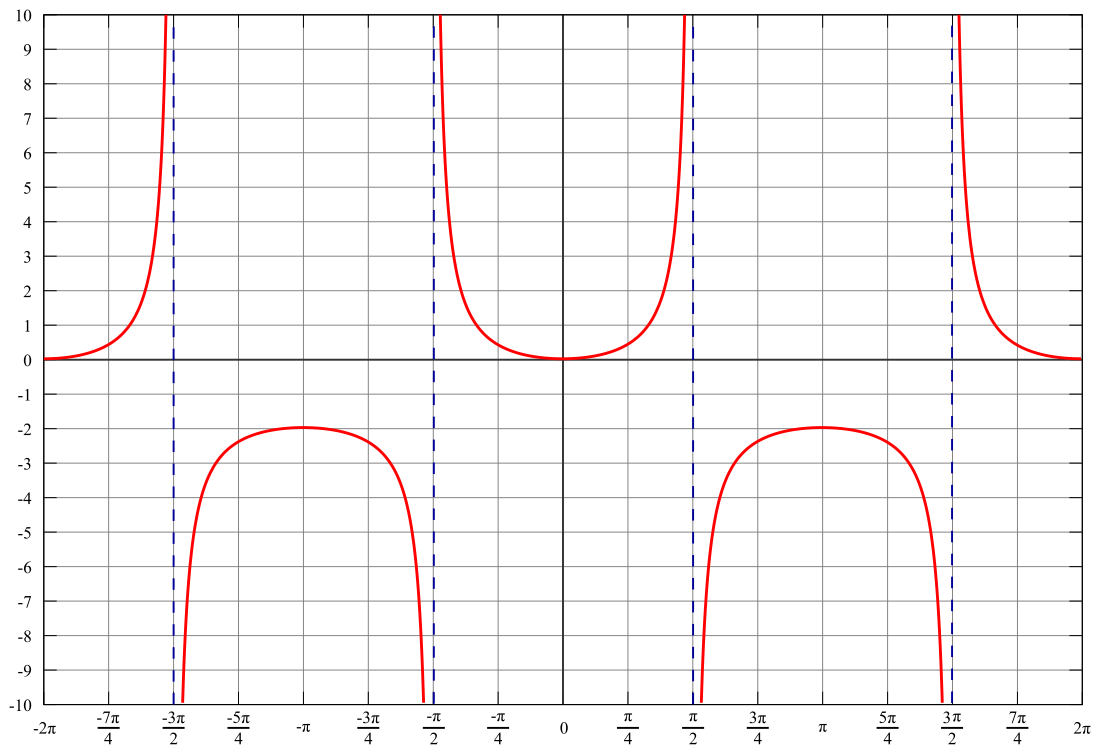
外正割（exsecant^{[1][2][3][4][5][6][7][8]}， 拉丁文：secans exterior^{[9][10][11][12]}） 是一種可以根據正割定義的三角函數，符号通常表示为${\displaystyle \operatorname {exsec} x}$^{[13][14][15][16]}或${\displaystyle \operatorname {exs} x}$^[17]， 曾經在应用在鐵道工程學（英语：Railway_engineering）、测量学、土木工程、天文學和球面几何学等学科，可以幫助提高精度，但如今除了簡化一些計算外很少使用。外正割的函數值比正割函數少1，換句話說，其與正割的關係可以用下列等式表達：^[16]

${\displaystyle \operatorname {exsec} x=\sec(x)-1}$。

外正割
性質
奇偶性	偶
定義域	${\displaystyle \left\{x\in \mathbb {R} |x\neq k\pi +{\tfrac {\pi }{2}},\,k\in \mathbb {Z} \right\}}$ ${\displaystyle \left\{x\in \mathbb {R} |x\neq 180^{\circ }k+90^{\circ },\,k\in \mathbb {Z} \right\}}$
到達域	${\displaystyle -2\geq \operatorname {exsec} x\geq 0}$
周期	${\displaystyle 2\pi }$ （360°）
特定值
當x=0	0
當x=+∞	N/A
當x=-∞	N/A
最大值	+∞
最小值	-∞
其他性質
渐近线	${\displaystyle x=\left(2k+1\right){\tfrac {\pi }{2}}}$ （x=180°k+90°）
根	${\displaystyle 2k\pi }$ （${\displaystyle 360^{\circ }k}$）
臨界點	${\displaystyle k\pi }$ （180°k）
k是一個整數。

在單位圓上，外正割位於正割線上單位圓的外側，因此稱為外正割。 此外，外正割也有exterior secant、 external secant^{[18][19][20][21]}、 outward secant或outer secant等稱呼。

定義

在单位圆上表示的三角函数

在單位圓上，角${\displaystyle \theta }$的外正割可以定義為，在x軸上，從單位圓圓周沿x軸到「角${\displaystyle \theta }$的終邊與單位圓交點的切線」的長度。 由於從角的頂點沿x軸到「角${\displaystyle \theta }$的終邊與單位圓交點的切線」的長度為正割，因此正割與外正割相差1，即外正割為正割扣掉單位圓半徑。

外正割也可以定義為：^[22]

${\displaystyle \operatorname {exsec} (\theta )=\sec(\theta )-1={\frac {1}{\cos(\theta )}}-1}$

用途

直到20世紀80年代，外正割函數在測量學^[7]、鐵路工程（英语：Railway engineering）^[4]（例如佈置鐵路曲線和橫向高度（英语：Cant_(road/rail)））、土木工程、天文學和球面三角學等領域都很重要，但現在很少使用。^[7][22]這主要是因為计算器和计算机的廣泛使用已經消除了對諸如此類的專門函數的三角表的需求。^[7]

定義一個特殊的外正割函數的原因與定義正矢函數的理據相似： 對於小角度 θ，sec(θ) 函數值接近於1，因此使用上述的外正割公式涉及減法時會幾乎會出現兩個幾乎相等的數量的相減，這個過程可能導致導致嚴重的灾难性抵消或數值誤差。 因此，正割函數表需要非常高的精確度才能用於外正割，這使得專用的外正割函數表非常有用。 即使使用计算机，如果使用基於餘弦的定義，對於小角度的外正割來說，浮點誤差可能會成為問題。 在這種極限情況下，更準確的公式應該使用這個恒等式：^[2][3][21]

$${\displaystyle \operatorname {exsec} (\theta )={\frac {1-\cos(\theta )}{\cos(\theta )}}={\frac {\operatorname {versin} (\theta )}{\cos(\theta )}}=\operatorname {versin} (\theta )\sec(\theta )=2\left(\sin \left({\frac {\theta }{2}}\right)\right)^{2}\sec(\theta )}$$

在計算機出現之前，這需要相當費時的乘法運算。

伽利略·伽利莱早在 1632 年就使用了外正割函數，但當時他將其稱為segante（意為正割）^{[23][24][25][26]}。 其拉丁語名稱secans external至少可以追朔到1745年左右才開始有使用紀錄^{[9][10][11][12]}。 而其英語名稱external secant和其縮寫縮寫ex的用法：ex.sec，最早可以追溯到1855 年。 當時查爾斯·哈斯萊特（Charles Haslett）發表了第一個已知最早的外正割函數表^[27][28]。 ex secant和exsec等變體於則需要到1880年才開始有使用紀錄^[18]。1894年後，exsecant一術語的使用則比較少。^[1]

數學性質

导数

${\displaystyle {\frac {\mathrm {d} }{\mathrm {d} \theta }}\operatorname {exsec} (\theta )=\tan(\theta )\sec(\theta )={\frac {\sin(\theta )}{\cos ^{2}(\theta )}}}$^[16]

積分

${\displaystyle \int \operatorname {exsec} (\theta )\,\mathrm {d} \theta =\ln \left[\cos \left({\frac {\theta }{2}}\right)+\sin \left({\frac {\theta }{2}}\right)\right]-\ln \left[\cos \left({\frac {\theta }{2}}\right)-\sin \left({\frac {\theta }{2}}\right)\right]-\theta +C}$^[16]

其他性质

單位圓上的外正割等價於正割減去餘弦再減去正矢

外正割可由單位圓推導：

${\displaystyle \operatorname {exsec} (\theta )=\sec(\theta )-\cos(\theta )-\operatorname {versin} (\theta ).}$

外正割函数可由正切函数表示为：^[22]

${\displaystyle \operatorname {exsec} (\theta )=\tan(\theta )\tan \left({\frac {\theta }{2}}\right).}$

外正割函數與正弦函數的關係為：

${\displaystyle \operatorname {exsec} (\theta )={\frac {1}{\sqrt {1-(\sin(\theta ))^{2}}}}-1.}$

外正割函數可以擴展到複平面。^[16]

其他恆等式

${\displaystyle \lim _{\theta \to 0}{\frac {\operatorname {exsec} (\theta )}{\theta }}=0}$^[4]

${\displaystyle {\frac {\operatorname {versin} (\theta )+\operatorname {coversin} (\theta )}{\operatorname {versin} (\theta )-\operatorname {coversin} (\theta )}}-{\frac {\operatorname {exsec} (\theta )+\operatorname {excsc} (\theta )}{\operatorname {exsec} (\theta )-\operatorname {excsc} (\theta )}}={\frac {2\operatorname {versin} (\theta )\operatorname {coversin} (\theta )}{\operatorname {versin} (\theta )-\operatorname {coversin} (\theta )}}}$^[4]

${\displaystyle (\operatorname {exsec} (\theta )+\operatorname {versin} (\theta ))\,(\operatorname {excsc} (\theta )+\operatorname {coversin} (\theta ))=\sin(\theta )\cos(\theta )}$^[4]

${\displaystyle \operatorname {exsec} (2\theta )={\frac {2\sin ^{2}(\theta )}{1-2\sin ^{2}(\theta )}}}$^[4]

${\displaystyle \operatorname {exsec} (2\theta )\,\cos(2\theta )=\tan(\theta )}$^[4]

${\displaystyle \operatorname {exsec} ^{2}(\theta )+2\operatorname {exsec} (\theta )=\tan ^{2}(\theta )}$^[4]

反外正割

外正割的反函數

反外正割（arcexsecant^[29]）是外正割函數的反函數，符号通常表示为arcexsec^[4][29] 、aexsec^[30][31]、aexs或exsec⁻¹。

反外正割可以定義為：

${\displaystyle \operatorname {arcexsec} (y)=\operatorname {arcsec}(y+1)}$

${\displaystyle \ =\arccos \left({\frac {1}{y+1}}\right)=\arctan({\sqrt {y^{2}+2y}})}$^[29][30][31]（對所有的y ≤ −2或y ≥ 0）^[29]

參見

• 外餘割：角的餘割值減去1
• 正矢：1減去角的餘弦值
• 三角函数

參考文獻

1. Allen, Calvin Frank. Railroad Curves and Earthwork. New York, USA; London, UK: Spon & Chamberlain; E. & F. Spon, Ltd. 1894 [1889] [2015-11-16].
2. Nagle, James C. IV.138.-165.: Transition Curves; Table XIII.: Natural Versines and Exsecants. Field Manual for Railroad Engineers 1. New York, USA: John Wiley and Sons, Chapman and Hall, Limited（英语：Chapman & Hall）. 1897: 110–142;332–354 [2015-11-16].
3. Field Manual for Railroad Engineers (PDF). The Engineer（英语：The Engineer (UK magazine)） (Review). 1897-12-03: 540 [2015-11-17]. （原始内容存档 (PDF)于2015-11-17）.
4. Hall, Arthur Graham; Frink, Fred Goodrich. Review Exercises [100] Secondary Trigonometric Functions. 写于Ann Arbor, Michigan, USA. Trigonometry. Part I: Plane Trigonometry. New York, USA: Henry Holt and Company（英语：Henry Holt and Company） / Norwood Press / J. S. Cushing Co. - Berwick & Smith Co., Norwood, Massachusetts, USA. January 1909: 125 [2017-08-12].
5. Boyer, Carl Benjamin. 5: Commentary on the Paper of E. J. Dijksterhuis (The Origins of Classical Mechanics from Aristotle to Newton). Clagett, Marshall (编). Critical Problems in the History of Science 3. Madison, Milwaukee, and London: University of Wisconsin Press, Ltd.（英语：University_of_Wisconsin_Press）. 1969: 185–190 [1959] [2015-11-16]. ISBN 0-299-01874-1. LCCN 59-5304. 9780299018740.
6. Zucker, Ruth. Chapter 4.3.147: Elementary Transcendental Functions - Circular functions. Abramowitz, Milton; Stegun, Irene Ann (编). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables. Applied Mathematics Series 55 Ninth reprint with additional corrections of tenth original printing with corrections (December 1972); first. Washington D.C.; New York: United States Department of Commerce, National Bureau of Standards; Dover Publications. 1983: 78. ISBN 978-0-486-61272-0. LCCN 64-60036. MR 0167642.

   LCCN 65-12253

   .
7. Calvert, James B. Trigonometry. 2007-09-14 [2004-01-10] [2015-11-08]. （原始内容存档于2007-10-02）.
8. Tapson, Frank. Background Notes on Measures: Angles. 1.4. Cleave Books. 2004 [2015-11-12]. （原始内容存档于2007-02-09）.
9. Patu, Andræâ-Claudio (André Claude); Le Tort, Bartholomæus. Rivard, Franciscus (Dominique-François) , 编. Theses Mathematicæ De Mathesi Generatim. Collegio Dormano–BellovacoCollegio Dormano–Bellovaco（英语：Collegio Dormano–BellovacoCollegio Dormano–Bellovaco） (Collège de Dormans–Beauvais（英语：Collège de Dormans–Beauvais）), Paris: Ph. N. Lottin. February 1745: 6 [2017-08-06] （拉丁语）.
10. Lemonnier, Petro (Pierre). Genneau, Ludovicum (Ludovico); Rollin, Jacobum (Jacques) , 编. Physica generalis. Cursus Philosophicus Ad Scholarum Usum Accomodatus 3 (Collegio Harcuriano（英语：Collegio Harcuriano） (Collège d'Harcourt), Paris). 1750: 303– [2017-08-06] （拉丁语）.
11. Thysbaert, Jan-Frans. Articulus II: De situ lineæ rectæ ad Circularem; & de mensura angulorum, quorum vertex non est in circuli centro. §1. De situ lineæ rectæ ad Circularem. Definitio II: [102]. Geometria elementaria et practica. Lovanii, e typographia academica. 1774: 30, foldout [2017-08-06] （拉丁语）.
12. van Haecht, Joannes. Articulus III: De secantibus circuli: Corollarium III: [109]. Geometria elementaria et practica: quam in usum auditorum. Lovanii, e typographia academica. 1784: 24, foldout [2017-08-06] （拉丁语）.
13. Frye, Albert I. Civil engineer's pocket-book: a reference-book for engineers, contractors and students containing rules, data, methods, formulas and tables 2 (corrected). New York, USA; London, UK: D. Van Nostrand Company（英语：D. Van Nostrand Company）; Constable and Company, Ltd.（英语：Constable and Company, Ltd.）. 1918 [1913] [2015-11-16].
14. Kenyon, Alfred Monroe; Ingold, Louis. Trigonometry. New York, USA: The Macmillan Company（英语：The Macmillan Company）. 1913: 5 [2015-12-08].
15. Hudson, Ralph Gorton; Lipka, Joseph. A Manual of Mathematics. New York, USA: John Wiley & Sons. 1917: 68 [2015-12-08]. （原始内容存档于2023-10-28）.
16. Weisstein, Eric W. (编). Exsecant. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. （英语）.
17. Shaneyfelt, Ted V. 德博士的 Notes About Circles, ज्य, & कोज्य: What in the world is a hacovercosine?. Hilo, Hawaii: University of Hawaii. [2015-11-08]. （原始内容存档于2015-09-19）.
18. Searles, William Henry. Field Engineering - A Hand-book of the Theory and Practice of Railway Surveying, Location, and Construction, designed for the Class-room, Field and Office, and containing a large number of useful tables, original and selected (PDF). New York, USA: John Wiley & Sons. 1880-03-01 [2017-08-13]. （原始内容存档 (PDF)于2017-08-13）. 8th revised edition, 1887 16th edition, 1910
19. Cajori, Florian. A History of Mathematical Notations 2 2 (3rd corrected printing of 1929 issue). Chicago, USA: Open court publishing company（英语：Open court publishing company）. 1952: 173 [March 1929] [2015-11-11]. ISBN 978-1-60206-714-1. 1602067147. (NB. ISBN and link for reprint of 2nd edition by Cosimo, Inc., New York, USA, 2013.)
20. Swanson, Todd; Andersen, Janet; Keeley, Robert. 5 (Trigonometric Functions) (PDF). Precalculus: A Study of Functions and Their Applications. Harcourt Brace & Company（英语：Harcourt Brace & Company）. 1999: 344 [2015-11-12]. （原始内容 (PDF)存档于2003-06-17）.
21. Gottschalk, Walter Helbig. Some Quaint & Curious & Almost Forgotten Trig Functions (PDF). Gottschalk's Gestalts - A Series Illustrating Innovative Forms of the Organization & Exposition of Mathematics 80 (Providence, Rhode Island, USA: Infinite Vistas Press). 2002 [2015-11-17]. PVD RI, GG80. （原始内容存档 (PDF)于2013-09-25）.
22. Oldham, Keith B.; Myland, Jan C.; Spanier, Jerome. 33.13. The Secant sec(x) and Cosecant csc(x) functions - Cognate functions. An Atlas of Functions: with Equator, the Atlas Function Calculator 2. Springer Science+Business Media, LLC. 2009: 336 [1987]. ISBN 978-0-387-48806-6. LCCN 2008937525. doi:10.1007/978-0-387-48807-3.
23. Galilei, Galileo. Dialogo di Galileo Galilei sopra i due massimi sistemi del mondo Tolemaico e Copernicano [Dialogue on the Two Chief World Systems, Ptolemaic and Copernican]. 1632 （意大利语）.
24. Galilei, Galileo. Finocchiaro, Maurice A. , 编. Galileo on the World Systems: A New Abridged Translation and Guide. University of California Press. 1997-05-25: 184 (n130), 184 (n135), 192 (n158) [1632] [2017-07-30]. ISBN 9780520918221. […] Galileo's word is segante (meaning secant), but he clearly intends exsecant; an exsecant is defined as the part of a secant external to the circle […] 含有內容需登入查看的頁面 (link)
25. Hawking, Stephen William (编). On the Shoulders of Giants: The Great Works of Physics and Astronomy. Philadelphia, USA: Running Press（英语：Running Press）. 2002 [2017-07-31]. ISBN 0-7624-1698-X. LCCN 2002100441. 含有內容需登入查看的頁面 (link)
26. Stávek, Jiří. On the Hidden Beauty of Trigonometric Functions. Applied Physics Research (Prague, CZ: Canadian Center of Science and Education). 2017-03-10, 9 (2): 57–64 [2017-02-26]. ISSN 1916-9639. doi:10.5539/apr.v9n2p57 . ISSN 1916-9647. [1]
27. Haslett, Charles. Hackley, Charles W. , 编. The Mechanic's, Machinist's, Engineer's Practical Book of Reference: Containing tables and formulæ for use in superficial and solid mensuration; strength and weight of materials; mechanics; machinery; hydraulics, hydrodynamics; marine engines, chemistry; and miscellaneous recipes. Adapted to and for the use of all classes of practical mechanics. Together with the Engineer's Field Book: Containing formulæ for the various of running and changing lines, locating side tracks and switches, &c., &c. Tables of radii and their logarithms, natural and logarithmic versed sines and external secants, natural sines and tangents to every degree and minute of the quadrant, and logarithms from the natural numbers from 1 to 10,000. New York, USA: James G. Gregory, successor of W. A. Townsend & Co. (Stringer & Townsend). September 1855 [2017-08-13]. […] Still there would be much labor of computation which may be saved by the use of tables of external secants and versed sines, which have been employed with great success recently by the Engineers on the Ohio and Mississippi Railroad（英语：Ohio and Mississippi Railroad）, and which, with the formulas and rules necessary for their application to the laying down of curves, drawn up by Mr. Haslett, one of the Engineers of that Road, are now for the first time given to the public. […] In presenting this work to the public, the Author claims for it the adaptation of a new principle in trigonometrical analysis of the formulas generally used in field calculations. Experience has shown, that versed sines and external secants as frequently enter into calculations on curves as sines and tangents; and by their use, as illustrated in the examples given in this work, it is believed that many of the rules in general use are much simplified, and many calculations concerning curves and running lines made less intricate, and results obtained with more accuracy and far less trouble, than by any methods laid down in works of this kind. The examples given have all been suggested by actual practice, and will explain themselves. […] As a book for practical use in field work, it is confidently believed that this is more direct in the application of rules and facility of calculation than any work now in use. In addition to the tables generally found in books of this kind, the author has prepared, with great labor, a Table of Natural and Logarithmic Versed Sines and External Secants, calculated to degrees, for every minute; also, a Table of Radii and their Logarithms, from 1° to 60°. […] 1856 edition
28. Poor, Henry Varnum (编). PRACTICAL BOOK OF REFERENCE, and Engineer's Field Book. By Charles Haslett, C.E. Edited by Professor Charles W. Hackley, 1 vol. 12mo. Pp. 617. Prico $2.50. Columbia College, N. Y. Stringer & Townsend (PDF). American Railroad Journal - Steam Navigation, Commerce, Mining, Manufacturers (Review). Second Quarto Series (J. H. Schultz & Co.). 1856-03-22, XII (12): 184 [2017-08-14]. Whole No. 1040, Vol. XX. （原始内容存档于2023-10-28）.
29. van Vlijmen, Oscar. Goniology. Eenheden, constanten en conversies. 2005-12-28 [2003] [2015-11-28]. （原始内容存档于2009-10-28）.
30. Simpson, David G. AUXTRIG (Fortran 90 source code). Greenbelt, Maryland, USA: NASA Goddard Space Flight Center. 2001-11-08 [2015-10-26]. （原始内容存档于2008-06-16）.
31. van den Doel, Kees. jass.utils Class Fmath. JASS - Java Audio Synthesis System. 1.25. 2010-01-25 [2015-10-26]. （原始内容存档于2007-09-02）.

外部連結

• 埃里克·韦斯坦因. 外正割. MathWorld.