在幾何學中,複合多面體(英語:Polyhedral compound)又稱為多面體複合物,是由本身與幾個多面體共享的一個共同的幾何中心的多面體。它們是星形多邊形的三維類比,如六角星。
正複合多面體
| 名稱 | 圖像 | 凸包 | 核心 | 對稱群 | Subgroup restricting to one constituent |
對偶性 |
|---|---|---|---|---|---|---|
| 二複合正四面體 星形八面體 |

|
正方體 | 正八面體 | *432 [4,3] Oh |
*332 [3,3] Td |
自身對偶 |
| 五複合正四面體 | 
|
正十二面體 | 正二十面體 | 532 [5,3]+ I |
332 [3,3]+ T |
enantiomorph, or chiral twin |
| 十複合正四面體 | 
|
正十二面體 | 正二十面體 | *532 [5,3] Ih |
332 [3,3] T |
自身對偶 |
| 五複合正六面體 | 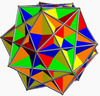
|
正十二面體 | 菱形三十面體 | *532 [5,3] Ih |
3*2 [3,3] Th |
五複合正八面體 |
| 五複合正八面體 | 
|
截半二十面體 | 正二十面體 | *532 [5,3] Ih |
3*2 [3,3] Th |
五複合正六面體 |
對偶-正複合多面體
均勻複合體
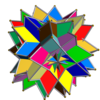
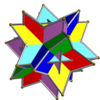
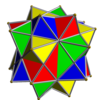
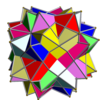
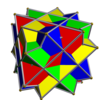
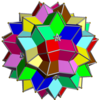
1976年約翰·斯基林發表的均勻多面體中共列出了75個均勻複合體[1]
- 1-19: Miscellaneous (4,5,6,9,17 are the 5 regular compounds)

|
|
|

|

|

|

|

|

|
|
|
|

|

|
|

|

|

|

|
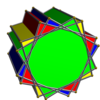
- 20-25: Prism symmetry embedded in prism symmetry,
|

|

|

|

|

|
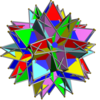
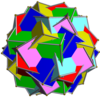
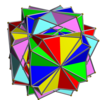
- 26-45: Prism symmetry embedded in octahedral or icosahedral symmetry,

|

|

|
|

|

|

|

|
|

|

|

|

|

|

|

|

|
|

|

|
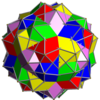
- 46-67: Tetrahedral symmetry embedded in octahedral or icosahedral symmetry,

|
|

|
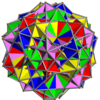
|

|

|

|

|

|

|

|

|

|

|

|
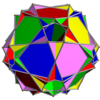
|

|

|

|
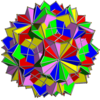
|

|

|
- 68-75: enantiomorph pairs

|

|

|

|

|

|

|

|
廣義的多面體
廣義的多面體也可以存在複合物的形式,但多半為星形鑲嵌的對偶鑲嵌,例如:二複合正六邊形鑲嵌。較常見的有複合鑲嵌圖等圖形。
| 名稱 | 圖像 | 凸包 | 核心 | 對稱群 |
|---|---|---|---|---|
| 複合三角形鑲嵌六邊形鑲嵌 | 
|
鳶形鑲嵌 | 小斜方截半六邊形鑲嵌 | p6, [6,3]+, (632) |
參考文獻
Wikiwand in your browser!
Seamless Wikipedia browsing. On steroids.
Every time you click a link to Wikipedia, Wiktionary or Wikiquote in your browser's search results, it will show the modern Wikiwand interface.
Wikiwand extension is a five stars, simple, with minimum permission required to keep your browsing private, safe and transparent.
Remove ads




