卡馬薩-霍爾姆方程 (Camassa Holm equation)是流體力學中的一個非線性偏微分方程
u
t
+
2
κ
u
x
−
u
x
x
t
+
3
u
u
x
=
2
u
x
u
x
x
+
u
u
x
x
x
.
{\displaystyle u_{t}+2\kappa u_{x}-u_{xxt}+3uu_{x}=2u_{x}u_{xx}+uu_{xxx}.\,}
1993年卡馬薩和霍爾姆以此偏微分方程模擬淺水波[ 1]
其中κ是大於0的參數。
卡馬薩-霍爾姆方程3D動畫 卡馬薩-霍爾姆方程有行波解[ 2]
u
2
:=
(
3
/
2
)
∗
c
∗
(
c
−
2
∗
κ
)
(
c
o
s
h
(
(
1
/
2
)
∗
(
(
c
−
2
∗
κ
)
/
c
)
∗
(
−
x
−
x
0
+
c
∗
t
)
)
2
∗
(
κ
+
c
)
)
{\displaystyle u2:=(3/2)*{\frac {c*(c-2*\kappa )}{(cosh((1/2)*{\sqrt {((c-2*\kappa )/c)}}*(-x-x0+c*t))^{2}*(\kappa +c))}}}
參數：c = 1, x0 = 1, kappa = .3 代人得：
u
(
x
,
t
)
=
.463
c
o
s
h
(
−
.316
∗
x
−
.316
+
.316
∗
t
)
2
{\displaystyle u(x,t)={\frac {.463}{cosh(-.316*x-.316+.316*t)^{2}}}}
Maple 軟件包TWSolution可提供多種行波解[ 3]
sech 展開
g
[
2
]
:=
u
(
x
,
t
)
=
−
(
1
/
2
)
∗
k
a
p
p
a
+
C
5
∗
s
e
c
h
(
C
1
+
(
1
/
2
)
∗
s
q
r
t
(
3
)
∗
x
−
(
1
/
4
)
∗
k
a
p
p
a
∗
s
q
r
t
(
3
)
∗
t
)
2
{\displaystyle g[2]:={u(x,t)=-(1/2)*kappa+_{C}5*sech(_{C}1+(1/2)*sqrt(3)*x-(1/4)*kappa*sqrt(3)*t)^{2}}}
g
[
3
]
:=
u
(
x
,
t
)
=
−
(
1
/
2
)
∗
k
a
p
p
a
+
C
5
∗
s
e
c
h
(
C
1
−
(
1
/
2
)
∗
s
q
r
t
(
3
)
∗
x
+
(
1
/
4
)
∗
k
a
p
p
a
∗
s
q
r
t
(
3
)
∗
t
)
2
{\displaystyle g[3]:={u(x,t)=-(1/2)*kappa+_{C}5*sech(_{C}1-(1/2)*sqrt(3)*x+(1/4)*kappa*sqrt(3)*t)^{2}}}
g
[
4
]
:=
u
(
x
,
t
)
=
C
4
+
(
−
(
3
/
2
)
∗
C
4
−
(
3
/
4
)
∗
k
a
p
p
a
)
∗
s
e
c
h
(
C
1
+
(
1
/
2
∗
I
)
∗
s
q
r
t
(
3
)
∗
x
−
(
1
/
4
∗
I
)
∗
k
a
p
p
a
∗
s
q
r
t
(
3
)
∗
t
)
2
{\displaystyle g[4]:={u(x,t)=_{C}4+(-(3/2)*_{C}4-(3/4)*kappa)*sech(_{C}1+(1/2*I)*sqrt(3)*x-(1/4*I)*kappa*sqrt(3)*t)^{2}}}
g
[
5
]
:=
u
(
x
,
t
)
=
C
4
+
(
−
(
3
/
2
)
∗
C
4
−
(
3
/
4
)
∗
k
a
p
p
a
)
∗
s
e
c
h
(
C
1
−
(
1
/
2
∗
I
)
∗
s
q
r
t
(
3
)
∗
x
+
(
1
/
4
∗
I
)
∗
k
a
p
p
a
∗
s
q
r
t
(
3
)
∗
t
)
2
{\displaystyle g[5]:={u(x,t)=_{C}4+(-(3/2)*_{C}4-(3/4)*kappa)*sech(_{C}1-(1/2*I)*sqrt(3)*x+(1/4*I)*kappa*sqrt(3)*t)^{2}}}
g
[
6
]
:=
u
(
x
,
t
)
=
−
(
C
3
−
4
∗
C
3
∗
C
2
2
+
2
∗
k
a
p
p
a
∗
C
2
)
/
(
C
2
∗
(
3
+
4
∗
C
2
2
)
)
+
24
∗
C
2
∗
(
2
∗
C
3
+
k
a
p
p
a
∗
C
2
)
∗
s
e
c
h
(
C
1
+
C
2
∗
x
+
C
3
∗
t
)
2
/
(
16
∗
C
2
4
−
9
)
{\displaystyle g[6]:={u(x,t)=-(_{C}3-4*_{C}3*_{C}2^{2}+2*kappa*_{C}2)/(_{C}2*(3+4*_{C}2^{2}))+24*_{C}2*(2*_{C}3+kappa*_{C}2)*sech(_{C}1+_{C}2*x+_{C}3*t)^{2}/(16*_{C}2^{4}-9)}}
Camassa Holm equation traveling wave sech plot5
Camassa Holm equation traveling wave sech plot4
Camassa Holm equation traveling wave sech plot6
exp 展開
g
[
2
]
:=
u
(
x
,
t
)
=
−
(
1
/
9
)
∗
s
q
r
t
(
3
)
∗
C
3
−
(
1
/
3
)
∗
k
a
p
p
a
+
C
5
∗
e
x
p
(
C
1
−
s
q
r
t
(
3
)
∗
x
+
C
3
∗
t
)
{\displaystyle g[2]:={u(x,t)=-(1/9)*sqrt(3)*_{C}3-(1/3)*kappa+_{C}5*exp(_{C}1-sqrt(3)*x+_{C}3*t)}}
g
[
3
]
:=
u
(
x
,
t
)
=
(
1
/
9
)
∗
s
q
r
t
(
3
)
∗
C
3
−
(
1
/
3
)
∗
k
a
p
p
a
+
C
5
∗
e
x
p
(
C
1
+
s
q
r
t
(
3
)
∗
x
+
C
3
∗
t
)
{\displaystyle g[3]:={u(x,t)=(1/9)*sqrt(3)*_{C}3-(1/3)*kappa+_{C}5*exp(_{C}1+sqrt(3)*x+_{C}3*t)}}
g
[
5
]
:=
u
(
x
,
t
)
=
−
(
1
/
3
)
∗
s
q
r
t
(
3
)
∗
C
3
−
(
1
/
3
)
∗
k
a
p
p
a
+
C
7
∗
(
e
x
p
(
C
1
−
(
1
/
3
)
∗
s
q
r
t
(
3
)
∗
x
+
C
3
∗
t
)
)
3
{\displaystyle g[5]:={u(x,t)=-(1/3)*sqrt(3)*_{C}3-(1/3)*kappa+_{C}7*(exp(_{C}1-(1/3)*sqrt(3)*x+_{C}3*t))^{3}}}
Camassa Holm equation traveling wave exp plot2
Camassa Holm equation traveling wave exp plot3
Camassa Holm equation traveling wave exp plot5
csch 展開
g
[
2
]
:=
u
(
x
,
t
)
=
−
(
1
/
2
)
∗
k
a
p
p
a
+
C
5
∗
c
s
c
h
(
C
1
+
(
1
/
2
)
∗
s
q
r
t
(
3
)
∗
x
−
(
1
/
4
)
∗
k
a
p
p
a
∗
s
q
r
t
(
3
)
∗
t
)
2
{\displaystyle g[2]:={u(x,t)=-(1/2)*kappa+_{C}5*csch(_{C}1+(1/2)*sqrt(3)*x-(1/4)*kappa*sqrt(3)*t)^{2}}}
g
[
4
]
:=
u
(
x
,
t
)
=
C
4
+
(
(
3
/
2
)
∗
C
4
+
(
3
/
4
)
∗
k
a
p
p
a
)
∗
c
s
c
h
(
C
1
+
(
1
/
2
∗
I
)
∗
s
q
r
t
(
3
)
∗
x
−
(
1
/
4
∗
I
)
∗
k
a
p
p
a
∗
s
q
r
t
(
3
)
∗
t
)
2
{\displaystyle g[4]:={u(x,t)=_{C}4+((3/2)*_{C}4+(3/4)*kappa)*csch(_{C}1+(1/2*I)*sqrt(3)*x-(1/4*I)*kappa*sqrt(3)*t)^{2}}}
g
[
6
]
:=
u
(
x
,
t
)
=
−
(
C
3
−
4
∗
C
3
∗
C
2
2
+
2
∗
k
a
p
p
a
∗
C
2
)
/
(
C
2
∗
(
4
∗
C
2
2
+
3
)
)
−
24
∗
C
2
∗
(
2
∗
C
3
+
k
a
p
p
a
∗
C
2
)
∗
c
s
c
h
(
C
1
+
C
2
∗
x
+
C
3
∗
t
)
2
/
(
16
∗
C
2
4
−
9
)
{\displaystyle g[6]:={u(x,t)=-(_{C}3-4*_{C}3*_{C}2^{2}+2*kappa*_{C}2)/(_{C}2*(4*_{C}2^{2}+3))-24*_{C}2*(2*_{C}3+kappa*_{C}2)*csch(_{C}1+_{C}2*x+_{C}3*t)^{2}/(16*_{C}2^{4}-9)}}
Camassa Holm equation traveling wave csch plot2
Camassa Holm equation traveling wave csch plot4
Camassa Holm equation traveling wave csch plot6
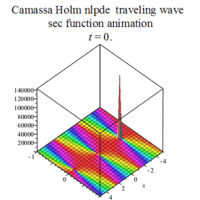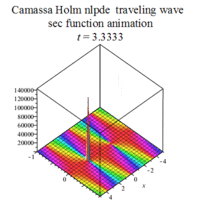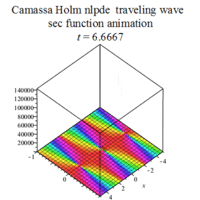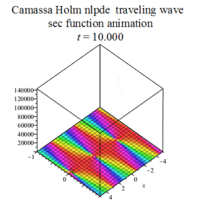
sec 展開
g
[
3
]
:=
u
(
x
,
t
)
=
−
(
1
/
2
)
∗
k
a
p
p
a
+
C
5
∗
s
e
c
(
C
1
−
(
1
/
2
∗
I
)
∗
s
q
r
t
(
3
)
∗
x
+
(
1
/
4
∗
I
)
∗
k
a
p
p
a
∗
s
q
r
t
(
3
)
∗
t
)
2
{\displaystyle g[3]:={u(x,t)=-(1/2)*kappa+_{C}5*sec(_{C}1-(1/2*I)*sqrt(3)*x+(1/4*I)*kappa*sqrt(3)*t)^{2}}}
g
[
5
]
:=
u
(
x
,
t
)
=
C
4
+
(
−
(
3
/
2
)
∗
C
4
−
(
3
/
4
)
∗
k
a
p
p
a
)
∗
s
e
c
(
C
1
−
(
1
/
2
)
∗
s
q
r
t
(
3
)
∗
x
+
(
1
/
4
)
∗
k
a
p
p
a
∗
s
q
r
t
(
3
)
∗
t
)
2
{\displaystyle g[5]:={u(x,t)=_{C}4+(-(3/2)*_{C}4-(3/4)*kappa)*sec(_{C}1-(1/2)*sqrt(3)*x+(1/4)*kappa*sqrt(3)*t)^{2}}}
g
[
6
]
:=
u
(
x
,
t
)
=
(
C
3
+
4
∗
C
3
∗
C
2
2
+
2
∗
k
a
p
p
a
∗
C
2
)
/
(
C
2
∗
(
4
∗
C
2
2
−
3
)
)
−
24
∗
C
2
∗
(
2
∗
C
3
+
k
a
p
p
a
∗
C
2
)
∗
s
e
c
(
C
1
+
C
2
∗
x
+
C
3
∗
t
)
2
/
(
16
∗
C
2
4
−
9
)
{\displaystyle g[6]:={u(x,t)=(_{C}3+4*_{C}3*_{C}2^{2}+2*kappa*_{C}2)/(_{C}2*(4*_{C}2^{2}-3))-24*_{C}2*(2*_{C}3+kappa*_{C}2)*sec(_{C}1+_{C}2*x+_{C}3*t)^{2}/(16*_{C}2^{4}-9)}}
JacobiSN 展開
g
[
3
]
:=
u
(
x
,
t
)
=
(
1
/
9
∗
I
)
∗
s
q
r
t
(
3
)
∗
C
4
−
(
1
/
3
)
∗
k
a
p
p
a
+
C
6
∗
s
i
n
(
C
2
−
I
∗
s
q
r
t
(
3
)
∗
x
+
C
4
∗
t
)
{\displaystyle g[3]:={u(x,t)=(1/9*I)*sqrt(3)*_{C}4-(1/3)*kappa+_{C}6*sin(_{C}2-I*sqrt(3)*x+_{C}4*t)}}
g
[
4
]
:=
u
(
x
,
t
)
=
−
(
2
/
9
∗
I
)
∗
s
q
r
t
(
3
)
∗
C
4
−
(
1
/
2
)
∗
C
7
−
(
1
/
3
)
∗
k
a
p
p
a
+
C
7
∗
s
i
n
(
C
2
+
(
1
/
2
∗
I
)
∗
s
q
r
t
(
3
)
∗
x
+
C
4
∗
t
)
2
{\displaystyle g[4]:={u(x,t)=-(2/9*I)*sqrt(3)*_{C}4-(1/2)*_{C}7-(1/3)*kappa+_{C}7*sin(_{C}2+(1/2*I)*sqrt(3)*x+_{C}4*t)^{2}}}
Camassa Holm equation traveling wave Jacobish plot4
{\displaystyle }
{\displaystyle }
{\displaystyle }
{\displaystyle }
{\displaystyle }
![{\displaystyle g[2]:={u(x,t)=-(1/2)*kappa+_{C}5*sech(_{C}1+(1/2)*sqrt(3)*x-(1/4)*kappa*sqrt(3)*t)^{2}}}](http://wikimedia.org/api/rest_v1/media/math/render/svg/534172915910d0fc9c287c8363b2e61ef423e779)
![{\displaystyle g[3]:={u(x,t)=-(1/2)*kappa+_{C}5*sech(_{C}1-(1/2)*sqrt(3)*x+(1/4)*kappa*sqrt(3)*t)^{2}}}](http://wikimedia.org/api/rest_v1/media/math/render/svg/9e932e98b1b8811e79dad09058f8b3deca8e540a)
![{\displaystyle g[4]:={u(x,t)=_{C}4+(-(3/2)*_{C}4-(3/4)*kappa)*sech(_{C}1+(1/2*I)*sqrt(3)*x-(1/4*I)*kappa*sqrt(3)*t)^{2}}}](http://wikimedia.org/api/rest_v1/media/math/render/svg/d9dd47880d20786016e07300ec2f09fd323aa321)
![{\displaystyle g[5]:={u(x,t)=_{C}4+(-(3/2)*_{C}4-(3/4)*kappa)*sech(_{C}1-(1/2*I)*sqrt(3)*x+(1/4*I)*kappa*sqrt(3)*t)^{2}}}](http://wikimedia.org/api/rest_v1/media/math/render/svg/3fc3e6d7fdac8c02e851279b85db5d9efc8fd42a)
![{\displaystyle g[6]:={u(x,t)=-(_{C}3-4*_{C}3*_{C}2^{2}+2*kappa*_{C}2)/(_{C}2*(3+4*_{C}2^{2}))+24*_{C}2*(2*_{C}3+kappa*_{C}2)*sech(_{C}1+_{C}2*x+_{C}3*t)^{2}/(16*_{C}2^{4}-9)}}](http://wikimedia.org/api/rest_v1/media/math/render/svg/2cf35aad5008a313ede50470e1ec54cf32b56ba9)
Camassa Holm equation traveling wave sech plot5
Camassa Holm equation traveling wave sech plot4
Camassa Holm equation traveling wave sech plot6
![{\displaystyle g[2]:={u(x,t)=-(1/9)*sqrt(3)*_{C}3-(1/3)*kappa+_{C}5*exp(_{C}1-sqrt(3)*x+_{C}3*t)}}](http://wikimedia.org/api/rest_v1/media/math/render/svg/38ab183e8d4ab924898db1e69a3f34de513ed54c)
![{\displaystyle g[3]:={u(x,t)=(1/9)*sqrt(3)*_{C}3-(1/3)*kappa+_{C}5*exp(_{C}1+sqrt(3)*x+_{C}3*t)}}](http://wikimedia.org/api/rest_v1/media/math/render/svg/32311524dfa46f57ca661976ff6c302086cfa1a0)
![{\displaystyle g[5]:={u(x,t)=-(1/3)*sqrt(3)*_{C}3-(1/3)*kappa+_{C}7*(exp(_{C}1-(1/3)*sqrt(3)*x+_{C}3*t))^{3}}}](http://wikimedia.org/api/rest_v1/media/math/render/svg/550164212c72c3cd51596a9a7fb8ff512338598a)
Camassa Holm equation traveling wave exp plot2
Camassa Holm equation traveling wave exp plot3
Camassa Holm equation traveling wave exp plot5
![{\displaystyle g[2]:={u(x,t)=-(1/2)*kappa+_{C}5*csch(_{C}1+(1/2)*sqrt(3)*x-(1/4)*kappa*sqrt(3)*t)^{2}}}](http://wikimedia.org/api/rest_v1/media/math/render/svg/6f4fa0e3881d22b4d48c7134eefa28662582ef55)
![{\displaystyle g[4]:={u(x,t)=_{C}4+((3/2)*_{C}4+(3/4)*kappa)*csch(_{C}1+(1/2*I)*sqrt(3)*x-(1/4*I)*kappa*sqrt(3)*t)^{2}}}](http://wikimedia.org/api/rest_v1/media/math/render/svg/c775cad9f9ca951e3baf130a3e58fa48dd799766)
![{\displaystyle g[6]:={u(x,t)=-(_{C}3-4*_{C}3*_{C}2^{2}+2*kappa*_{C}2)/(_{C}2*(4*_{C}2^{2}+3))-24*_{C}2*(2*_{C}3+kappa*_{C}2)*csch(_{C}1+_{C}2*x+_{C}3*t)^{2}/(16*_{C}2^{4}-9)}}](http://wikimedia.org/api/rest_v1/media/math/render/svg/8b38f929a34ddb5848d9d8bc986b4b0950bac352)
Camassa Holm equation traveling wave csch plot2
Camassa Holm equation traveling wave csch plot4
Camassa Holm equation traveling wave csch plot6
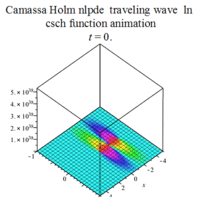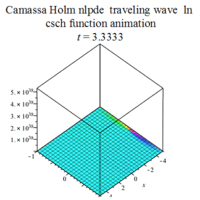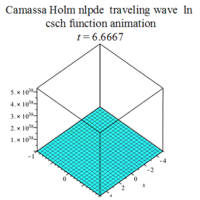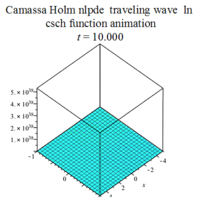
![{\displaystyle g[3]:={u(x,t)=-(1/2)*kappa+_{C}5*sec(_{C}1-(1/2*I)*sqrt(3)*x+(1/4*I)*kappa*sqrt(3)*t)^{2}}}](http://wikimedia.org/api/rest_v1/media/math/render/svg/e61ab8bed7a3dd0f6e463174c766cdf2892cf0d0)
![{\displaystyle g[5]:={u(x,t)=_{C}4+(-(3/2)*_{C}4-(3/4)*kappa)*sec(_{C}1-(1/2)*sqrt(3)*x+(1/4)*kappa*sqrt(3)*t)^{2}}}](http://wikimedia.org/api/rest_v1/media/math/render/svg/828ea8e5628054133fa8e4ec5fa2d6b3c7382a69)
![{\displaystyle g[6]:={u(x,t)=(_{C}3+4*_{C}3*_{C}2^{2}+2*kappa*_{C}2)/(_{C}2*(4*_{C}2^{2}-3))-24*_{C}2*(2*_{C}3+kappa*_{C}2)*sec(_{C}1+_{C}2*x+_{C}3*t)^{2}/(16*_{C}2^{4}-9)}}](http://wikimedia.org/api/rest_v1/media/math/render/svg/691ce8f8aa4d9ad74c84385711f12bd19c71de5e)
Camassa Holm equation traveling wave sec plot3
Camassa Holm equation traveling wave sec plot5
![{\displaystyle g[3]:={u(x,t)=(1/9*I)*sqrt(3)*_{C}4-(1/3)*kappa+_{C}6*sin(_{C}2-I*sqrt(3)*x+_{C}4*t)}}](http://wikimedia.org/api/rest_v1/media/math/render/svg/3c4024e0ac596717eb08ab385b10e6300a8d86d4)
![{\displaystyle g[4]:={u(x,t)=-(2/9*I)*sqrt(3)*_{C}4-(1/2)*_{C}7-(1/3)*kappa+_{C}7*sin(_{C}2+(1/2*I)*sqrt(3)*x+_{C}4*t)^{2}}}](http://wikimedia.org/api/rest_v1/media/math/render/svg/d6a2965a23d0f49880344231a3b22ee483f6bb28)
