么半範疇

張量範疇（tensor category），或曰么半範疇（monoidal category）， 直覺地講，是個配上張量積的阿貝爾範疇（abelian category），可當作環的範疇化。

定義

數學中，一個張量範疇（tensor category，或稱么半範疇 monoidal category）是一個包含單一個對象的雙範疇）bicategory）。 更具體的描述：一個張量範疇是

• 一個範疇 ${\displaystyle \mathbb {C} }$;
• 被賦予張量積，即一個二元函子

${\displaystyle \otimes :\mathbb {C} \times \mathbb {C} \to \mathbb {C} }$;

• 被賦予一個單位對象 ${\displaystyle I}$;
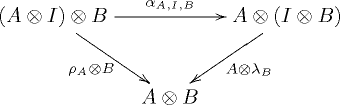
• 被賦予三組自然同構映射：
  • 結合子${\displaystyle \alpha }$: ${\displaystyle \alpha _{A,B,C}:(A\otimes B)\otimes C\to A\otimes (B\otimes C)}$;
  • 左/右單位子: 自然同構映射 ${\displaystyle \lambda }$, ${\displaystyle \rho }$:

${\displaystyle \lambda _{A}:I\otimes A\to A}$,

${\displaystyle \rho _{A}:A\otimes I\to A}$;

• 滿足以下相容條件:

每${\displaystyle A}$, ${\displaystyle B}$, ${\displaystyle C}$, ${\displaystyle D}$ ${\displaystyle \in }$ ${\displaystyle \mathbb {C} }$,

和

都交換.///

在這以上兩道相容條件下，任何以結合子,左右單位子和張量積組成的圖表都交換，因為 Mac Lane 凝聚定理(Mac Lane's coherence theorem): 每個么半範疇都 么半等價(monoidally equivalent) 於一嚴格么半範疇（見下）.

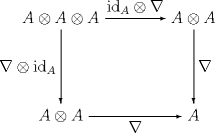
嚴格么半範疇

嚴格么半範疇(strict monoidal category) 是個么半範疇 ，其自然態射 ${\displaystyle \alpha }$, ${\displaystyle \lambda }$ 和 ${\displaystyle \rho }$ 都是恆等影射.

取任一 範疇 ${\displaystyle \mathbb {C} }$, 我們可構築其 自由嚴格么半範疇 ${\displaystyle \Sigma (\mathbb {C} )}$:

• 對象：其每一對象是一串由${\displaystyle \mathbb {C} }$裏面的對象組成之有限序列 ${\displaystyle (A_{1},\ldots ,A_{n}}$)；
• 態射：當且僅當${\displaystyle n=m}$時，我們在二個對象 ${\displaystyle (A_{1},\ldots ,A_{n})}$ 和 ${\displaystyle (B_{1},\ldots ,B_{m})}$ 之間定義 態射:每 ${\displaystyle \Sigma (\mathbb {C} )}$-態射 是一串由 ${\displaystyle \mathbb {C} }$-態射組成的有限序列 ${\displaystyle (f_{1}:A_{1}\to B_{1},\ldots ,f_{n}:A_{n}\to B_{n})}$ ；
• 張量積： 二個${\displaystyle \Sigma (\mathbb {C} )}$-對象${\displaystyle (A_{1},\ldots ,A_{n})}$ 及 ${\displaystyle (B_{1},\ldots ,B_{m})}$之張量積， 我們定義為 此二有限序列之串接(concatenation) ${\displaystyle (A_{1},\ldots ,A_{n},B_{1},\ldots ,B_{m})}$ ； 同樣地任何二 ${\displaystyle \Sigma (\mathbb {C} )}$-態射之張量積， 我們定義為其串接。

按：此算符 ${\displaystyle \Sigma }$ ，向由任一 範疇 ${\displaystyle \mathbb {C} }$ 配上 ${\displaystyle \Sigma (\mathbb {C} )}$，可推廣到 ${\displaystyle {\textbf {Cat}}}$上的嚴格-2-單子 （strict 2-monad）。

例

取任一範疇，若以其平常範疇積作張量積，以其終對象作單位對象，則成為一個張量範疇。 亦可取任一範疇，以其餘積（co-product）作張量積，以其始對象作單位對象，亦成一個張量範疇。 （此二例實為對稱么半範疇結構。） 但亦有許多張量範疇（例如：${\displaystyle R}$-Mod，如下），其張量積 既非 範疇積 亦非 範疇餘積。

以下舉張量範疇二例——向量空間範疇和集合範疇——並表明其類比：

${\displaystyle R}$-Mod	Set
取任一域 或交換環 ${\displaystyle R}$, 各 ${\displaystyle R}$-模 所成之 範疇 ${\displaystyle R}$-模 (若R 為一域， 則 R-模即 R－向量空間) 是一 對稱么半範疇；其張量積 ⊗ 與單位對象為：${\displaystyle R}$.	範疇 集 為一對稱么半範疇賦有張量積 × 與單位對象 {*}.
單元結合代數為${\displaystyle R}$-模之 一對象，賦上態射 ${\displaystyle \nabla :A\otimes A\rightarrow A}$ 與 ${\displaystyle \eta :R\rightarrow A}$ 並滿足以下條件:	A 么半群 為一對象 M ，配上態射 ${\displaystyle \circ :M\times M\rightarrow M}$與 ${\displaystyle 1:\{*\}\rightarrow M}$ 並滿足
and	與
.	.
A 餘代數(coalgebra) 是一個 對象 C ，被賦予 態射 ${\displaystyle \Delta :C\rightarrow C\otimes C}$ 和 ${\displaystyle \epsilon :C\rightarrow R}$ 並滿足以下條件:	集內每一對象（即每一集合）S， 都被賦予 態射 ${\displaystyle \Delta :S\rightarrow S\times S}$ 和 ${\displaystyle \epsilon :S\rightarrow \{*\}}$ 滿足以下條件:
and	and
.	.
	此 ε 是唯一的，因為 ${\displaystyle \{*\}}$ （即一元集合）是個終對象.

相關的結構

• 很多張量範疇更進一步有 辮, 交換態射 or 封閉等結構. 詳見下述參考。
• 么半函子（英語：monoidal functor）為二張量範疇（么半範疇）間、保存張量積結構之函子； 么半態射為二么半函子間之態射（自然變換 (natural transformations)）。
• 一般么半群之概念可推廣成么半範疇中的么半對象（英語：monoid object）。尤其者，可視一嚴格么半範疇作 範疇之「範疇」 Cat中的么半對象（並以卡氏積為么半結構）。
• 上有界交半格 構成一嚴格對稱么半範疇：其積為交，而單位元則為頂。

應用

• 么半範疇被用以定義直覺主義線性邏輯的積性部分模型。它們也是凝態物理中拓撲序的數學基礎。
• 張量範疇是二維保角場論和拓撲量子場論之代數框架.

參考

• Mac Lane, Saunders (1963). "Natural Associativity and Commutativity". Rice University Studies 49, 28–46.
• Kelly, G. Max (1964). "On MacLane's Conditions for Coherence of Natural Associativities, Commutativities, etc." Journal of Algebra 1, 397–402
• Joyal, André; Street, Ross (1993). "Braided Tensor Categories". Advances in Mathematics 102, 20–78.
• Mac Lane, Saunders (1997), Categories for the Working Mathematician (2nd ed.). New York: Springer-Verlag.
• Baez, John, Definitions （頁面存檔備份，存於互聯網檔案館）
• : <<Braided Category>>, <<Encyclopaedia of Mathematics>>,Springer On-line Reference Works （頁面存檔備份，存於互聯網檔案館）