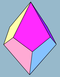
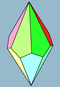
三方偏方面體

在幾何學中，三方偏方面體（英語：Trigonal Trapezohedron）又稱為又稱三方偏四角面體^[1]、三角鳶形多面體（英語：Trigonal Deltohedron）或雙反三角錐（英語：Trigonal Antidipyramid）是一個由六個全等的菱形組成的立體圖形，是六面體的一種，亦是平行六面體的特例，因其可視為由六個全等且等邊長的平行四邊形所組成。因為所有的邊緣都必須具有相同的長度，因此每一個三方偏方面體也是鳶形多面體。

三方偏方面體

類別	偏方面體
對偶多面體	三角反稜柱
數學表示法
考克斯特符號 （英語：Coxeter-Dynkin diagram）
性質
面	6 菱形
邊	12
頂點	8
歐拉特徵數	F=6, E=12, V=8 （χ=2）
組成與佈局
面的佈局 （英語：Face configuration）	3,3,3,3
對稱性
對稱群	D3d, [2+,6], (2*3), order 12
旋轉對稱群 （英語：Rotation_groups）	D3, [2,6]+, (223), order 6
特性
凸、面可遞
閱 論 編

三方偏方面體是最簡單的偏方面體無窮序列（即：三方偏方面體、四方偏方面體、五方、六方、七方......）即最簡單的反稜柱對偶多面體的無窮序列（二方偏方面體已退化為四面體）。

若三方偏方面體組成的菱形不只等邊且等角，此種三方偏方面體就是一個正六面體，即正方體或立方體，因為其面為正方形，因此若三方偏方面體的面維正方形就會是正多面體，反之，立方體就是三方偏方面體中的一個特例。

相關多面體

利用兩個四面體擴張八面體而建立的三方偏方面體，可以視為一個由十二個正三角形組成的非嚴格凸十二面體，然而其三角形兩兩共面形成60度菱形的面，因此不算詹森多面體，但可以算是一種擬詹森多面體。

偏方面體家族

2	3	4	5	6	7	8	9	10	11	12	...
球面投影

三方偏方面體是三角反角柱的對偶多面體，而三角反角柱可以由三角形二面體透過扭稜變換構造而來，因此與三角形二面體具有相同的對稱性，其可以衍生出一些相關的多面體：

半正三角形二面體球面多面體

對稱群（英語：List of spherical symmetry groups）：[3,2], (*322)							[3,2]+, (322)
{3,2}	t{3,2}	r{3,2}	2t{3,2}=t{2,3}	2r{3,2}={2,3}	rr{3,2}	tr{3,2}	sr{3,2}
半正對偶
V32	V62	V32	V4.4.3	V23	V4.4.3	V4.4.6	V3.3.3.3

參見

• 截對角三方偏方面體

參考文獻

1. 蘇偉昭. 晶形與其對應平面展開圖演算法與實作 (PDF). 物理教育學刊. 2019-12-01, 20 (2): 49–70 [2023-01-11]. ISSN 1998-7544. doi:10.6212/CPE.201912_20(2).0004.

• 埃里克·韋斯坦因. Trapezohedron. MathWorld.