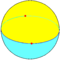
在几何学中,六阶五边形镶嵌是由五边形组成的双曲面正镶嵌图,每六个五边形共用一个顶点。在施莱夫利符号用{5,6}表示。六阶五形镶嵌即每个顶点皆为六个五边形的公共顶点,顶点周围包含了六个不重叠的五边形,一个五边形内角108度,六个五边形超过了360度,因此无法因此无法在平面作出,但可以在双曲面上作出。
交错涂色
该镶嵌也可以透过在[(5,5,3)]对称性中以两种颜色替五边形交错涂色而构成,其表示为 t1(5,5,3)。
对称性
这个镶嵌代表一个由六条镜射线定义一个正六边形基本域的万花筒,且五条镜射线相交于一点。 这由五个三阶交叉反射性在轨型符号被称为(*33333)。
相关多面体与镶嵌
该镶嵌在拓朴学上和顶点图是(5n)的一系列的镶嵌的一部份。
该镶嵌在拓朴学中也和每个顶点有著六个面的多面体及镶嵌相关, 施莱夫利符号皆为{n,6},而考斯特符号为![]()
![]()
![]()
![]()
![]() ,从n到无穷。
,从n到无穷。
| 正六边形/五边形镶嵌 | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 对称性:[6,5], (*652) | [6,5]+, (652) | [6,5+], (5*3) | [1+,6,5], (*553) | ||||||||

|

|

|

|

|

|

|

|

| |||
| {6,5} | t{6,5} | r{6,5} | 2t{6,5}=t{5,6} | 2r{6,5}={5,6} | rr{6,5} | tr{6,5} | sr{6,5} | s{5,6} | h{6,5} | ||
| 对偶镶嵌 | |||||||||||

|

|

|

|

|

|

|
|||||
| V65 | V5.12.12 | V5.6.5.6 | V6.10.10 | V56 | V4.5.4.6 | V4.10.12 | V3.3.5.3.6 | V3.3.3.5.3.5 | V(3.5)5 | ||
参见
参考资料
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 19, The Hyperbolic Archimedean Tessellations)
- Chapter 10: Regular honeycombs in hyperbolic space. The Beauty of Geometry: Twelve Essays. Dover Publications. 1999. ISBN 0-486-40919-8. LCCN 99035678.
外部链接
- 埃里克·韦斯坦因. Hyperbolic tiling. MathWorld.
- 埃里克·韦斯坦因. Poincaré hyperbolic disk. MathWorld.
- Hyperbolic and Spherical Tiling Gallery(页面存档备份,存于互联网档案馆)
- KaleidoTile 3: Educational software to create spherical, planar and hyperbolic tilings (页面存档备份,存于互联网档案馆)
- Hyperbolic Planar Tessellations, Don Hatch(页面存档备份,存于互联网档案馆)
Wikiwand in your browser!
Seamless Wikipedia browsing. On steroids.
Every time you click a link to Wikipedia, Wiktionary or Wikiquote in your browser's search results, it will show the modern Wikiwand interface.
Wikiwand extension is a five stars, simple, with minimum permission required to keep your browsing private, safe and transparent.