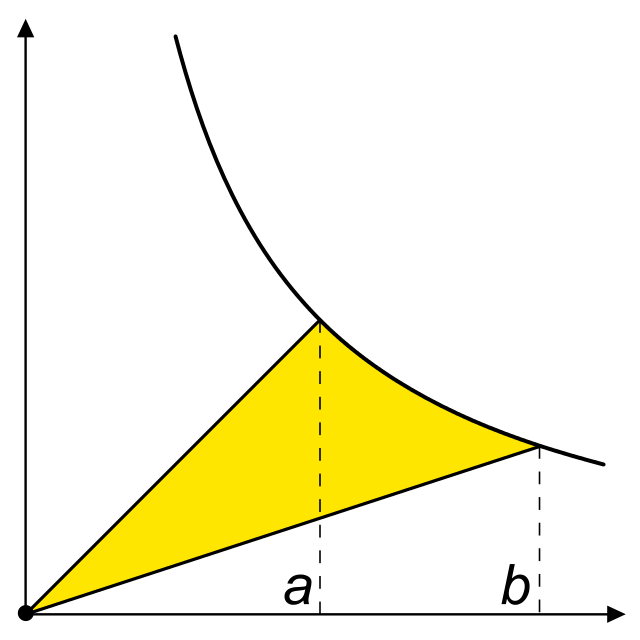
双曲线扇形 是笛卡尔平面
{
(
x
,
y
)
}
{\displaystyle \{(x,y)\}}
(
a
,
1
a
)
{\textstyle (a,{\frac {1}{a}})}
(
b
,
1
b
)
{\textstyle (b,{\frac {1}{b}})}
双曲线
x
y
=
1
{\displaystyle xy=1}
a
=
1
{\displaystyle a=1}
b
>
1
{\displaystyle b>1}
ln
(
b
)
{\displaystyle \ln(b)}
[ 2] 双曲角 。当直角双曲线下的两段面积相等时,
x
{\displaystyle x}
等比数列 ,
x
2
x
1
=
x
1
x
0
=
k
{\textstyle {\frac {x_{2}}{x_{1}}}={\frac {x_{1}}{x_{0}}}=k}
y
{\displaystyle y}
x
2
x
1
=
x
1
x
0
=
1
k
{\textstyle {\frac {x_{2}}{x_{1}}}={\frac {x_{1}}{x_{0}}}={\frac {1}{k}}}
约翰·纳皮尔 在1614年[ 3] 约斯特·比尔吉 在6年后[ 4] 对数表 ,当时通过对接近1的底数的大量乘幂 运算,来找到指定范围和精度的对数 和所对应的真数。当时还没出现有理数幂的概念,按后世的观点,约翰·纳皮尔 的底数0.999999910000000 相当接近
1
e
{\textstyle {\frac {1}{e}}}
[ 5] 约斯特·比尔吉 的底数1.000110000 相当接近自然对数的底数
e
{\displaystyle e}
约翰·纳皮尔 用了20年时间进行相当于数百万次乘法的计算,亨利·布里格斯 [ 6] 常用对数 表的编制。
形如
f
(
x
)
=
x
p
{\displaystyle f(x)=x^{p}}
反导数 ,除了特殊情况
p
=
−
1
{\displaystyle p=-1}
弓形面积 双曲线扇形 ;其他情况都由1635年发表的卡瓦列里弓形面积公式 [ 7] 阿基米德 完成(抛物线的弓形面积 ),双曲线的弓形面积需要发明一个新函数。1647年圣文森特的格列高利
x
y
=
1
{\displaystyle xy=1}
[
a
,
b
]
{\displaystyle [a,b]}
双曲线扇形 同
[
c
,
d
]
{\displaystyle [c,d]}
a
b
=
c
d
{\textstyle {\frac {a}{b}}={\frac {c}{d}}}
x
=
1
{\displaystyle x=1}
x
=
t
{\displaystyle x=t}
f
(
t
)
{\displaystyle f(t)}
[ 8]
f
(
t
u
)
=
f
(
t
)
+
f
(
u
)
{\displaystyle f(tu)=f(t)+f(u)\,}
1649年,萨拉萨的阿尔丰斯·安东尼奥 伊萨克·牛顿 推广了二项式定理 ,他将
1
1
+
x
{\textstyle {\frac {1}{1+x}}}
尼古拉斯·麦卡托 在1668年出版的著作《Logarithmotechnia》中[ 9] 麦卡托级数 。
大约1730年,欧拉 定义互为逆函数的指数函数 和自然对数为[ 10] [ 11]
e
x
=
lim
n
→
∞
(
1
+
x
n
)
n
,
{\displaystyle e^{x}=\lim _{n\rightarrow \infty }\left(1+{\frac {x}{n}}\right)^{n},}
ln
(
x
)
=
lim
n
→
∞
n
(
x
1
n
−
1
)
{\displaystyle \ln(x)=\lim _{n\rightarrow \infty }n\left(x^{\frac {1}{n}}-1\right)}
1742年威廉·琼斯 发表了现在的幂 指数 概念[ 12]
ln
(
1
)
=
∫
1
1
1
t
d
t
=
0
{\displaystyle \ln(1)=\int _{1}^{1}{\frac {1}{t}}\,dt=0\,}
ln
(
−
1
)
=
i
π
{\displaystyle \operatorname {ln} (-1)=i\pi \,}
(参见复数对数 )
ln
(
x
)
<
ln
(
y
)
f
o
r
0
<
x
<
y
{\displaystyle \ln(x)<\ln(y)\quad {\rm {for}}\quad 0<x<y\,}
lim
x
→
0
ln
(
1
+
x
)
x
=
1
{\displaystyle \lim _{x\to 0}{\frac {\ln(1+x)}{x}}=1\,}
ln
(
x
y
)
=
y
ln
(
x
)
{\displaystyle \ln(x^{y})=y\,\ln(x)\,}
x
−
1
x
≤
ln
(
x
)
≤
x
−
1
f
o
r
x
>
0
{\displaystyle {\frac {x-1}{x}}\leq \ln(x)\leq x-1\quad {\rm {for}}\quad x>0\,}
ln
(
1
+
x
α
)
≤
α
x
f
o
r
x
≥
0
,
α
≥
1
{\displaystyle \ln {(1+x^{\alpha })}\leq \alpha x\quad {\rm {for}}\quad x\geq 0,\alpha \geq 1\,}
More information
证明
lim
h
→
0
ln
(
1
+
h
)
h
=
lim
h
→
0
ln
(
1
+
h
)
−
ln
1
h
=
d
d
x
ln
x
|
x
=
1
=
1
{\displaystyle \lim _{h\to 0}{\frac {\ln(1+h)}{h}}=\lim _{h\to 0}{\frac {\ln(1+h)-\ln 1}{h}}={\frac {d}{dx}}\ln x{\Bigg |}_{x=1}=1}
Close
尽管自然对数没有简单的连分数 ,但有一些广义连分数 如:
ln
(
1
+
x
)
=
x
1
1
−
x
2
2
+
x
3
3
−
x
4
4
+
x
5
5
−
⋯
=
x
1
−
0
x
+
1
2
x
2
−
1
x
+
2
2
x
3
−
2
x
+
3
2
x
4
−
3
x
+
4
2
x
5
−
4
x
+
⋱
{\displaystyle {\begin{aligned}\ln(1+x)&={\frac {x^{1}}{1}}-{\frac {x^{2}}{2}}+{\frac {x^{3}}{3}}-{\frac {x^{4}}{4}}+{\frac {x^{5}}{5}}-\cdots \\&={\cfrac {x}{1-0x+{\cfrac {1^{2}x}{2-1x+{\cfrac {2^{2}x}{3-2x+{\cfrac {3^{2}x}{4-3x+{\cfrac {4^{2}x}{5-4x+\ddots }}}}}}}}}}\\\end{aligned}}}
ln
(
1
+
x
y
)
=
x
y
+
1
x
2
+
1
x
3
y
+
2
x
2
+
2
x
5
y
+
3
x
2
+
⋱
=
2
x
2
y
+
x
−
(
1
x
)
2
3
(
2
y
+
x
)
−
(
2
x
)
2
5
(
2
y
+
x
)
−
(
3
x
)
2
7
(
2
y
+
x
)
−
⋱
{\displaystyle {\begin{aligned}\ln \left(1+{\frac {x}{y}}\right)&={\cfrac {x}{y+{\cfrac {1x}{2+{\cfrac {1x}{3y+{\cfrac {2x}{2+{\cfrac {2x}{5y+{\cfrac {3x}{2+\ddots }}}}}}}}}}}}\\&={\cfrac {2x}{2y+x-{\cfrac {(1x)^{2}}{3(2y+x)-{\cfrac {(2x)^{2}}{5(2y+x)-{\cfrac {(3x)^{2}}{7(2y+x)-\ddots }}}}}}}}\\\end{aligned}}}
这些连分数特别是最后一个对接近1的值快速收敛。但是,更大的数的自然对数,可以轻易的用这些更小的数的自然对数的加法来计算,带有类似的快速收敛。
例如,因为
2
=
1.25
3
×
1.024
{\displaystyle 2=1.25^{3}\times 1.024}
2的自然对数 可以计算为:
ln
2
=
3
ln
(
1
+
1
4
)
+
ln
(
1
+
3
125
)
=
6
9
−
1
2
27
−
2
2
45
−
3
2
63
−
⋱
+
6
253
−
3
2
759
−
6
2
1265
−
9
2
1771
−
⋱
.
{\displaystyle {\begin{aligned}\ln 2&=3\ln \left(1+{\frac {1}{4}}\right)+\ln \left(1+{\frac {3}{125}}\right)\\&={\cfrac {6}{9-{\cfrac {1^{2}}{27-{\cfrac {2^{2}}{45-{\cfrac {3^{2}}{63-\ddots }}}}}}}}+{\cfrac {6}{253-{\cfrac {3^{2}}{759-{\cfrac {6^{2}}{1265-{\cfrac {9^{2}}{1771-\ddots }}}}}}}}.\\\end{aligned}}}
进而,因为
10
=
1.25
10
×
1.024
3
{\displaystyle 10=1.25^{10}\times 1.024^{3}}
ln
10
=
10
ln
(
1
+
1
4
)
+
3
ln
(
1
+
3
125
)
=
20
9
−
1
2
27
−
2
2
45
−
3
2
63
−
⋱
+
18
253
−
3
2
759
−
6
2
1265
−
9
2
1771
−
⋱
.
{\displaystyle {\begin{aligned}\ln 10&=10\ln \left(1+{\frac {1}{4}}\right)+3\ln \left(1+{\frac {3}{125}}\right)\\&={\cfrac {20}{9-{\cfrac {1^{2}}{27-{\cfrac {2^{2}}{45-{\cfrac {3^{2}}{63-\ddots }}}}}}}}+{\cfrac {18}{253-{\cfrac {3^{2}}{759-{\cfrac {6^{2}}{1265-{\cfrac {9^{2}}{1771-\ddots }}}}}}}}.\\\end{aligned}}}
例如哈代 和赖特 所著的《数论入门》"Introduction to the theory of numbers" (1.7, Sixth edition, Oxford 2008)的注解 "log x is, of course the 'Napierian' logarithm of x, to base e. 'Common' logarithms have no mathematical interest."(log x 当然是以e为基,x的“纳皮尔 ”对数。“常用”对数在数学上毫无重要。) 证明:从1到b 积分1/x ,增加三角形{(0, 0), (1, 0), (1, 1)},并减去三角形{(0, 0), (b , 0), (b , 1/b )}。
选取接近e的底数b,对数表涉及的bx 为单调增函数,定义域为0到1而值域为1到b;选取接近1/e的底数b,对数表涉及的bx 为单调减函数,定义域为0到∞而值域为1到0。
以
10
1
2
54
{\displaystyle 10^{\frac {1}{2^{54}}}}
博纳文图拉·卡瓦列里 在1635年的《Geometria indivisibilibus continuorum nova quadam ratione promota》中给出定积分 :
∫
0
a
x
n
d
x
=
1
n
+
1
a
n
+
1
n
≥
0
,
{\displaystyle \int _{0}^{a}x^{n}\,dx={\tfrac {1}{n+1}}\,a^{n+1}\qquad n\geq 0,}
不定积分 形式为:
∫
x
n
d
x
=
1
n
+
1
x
n
+
1
+
C
n
≠
−
1.
{\displaystyle \int x^{n}\,dx={\tfrac {1}{n+1}}\,x^{n+1}+C\qquad n\neq -1.}
皮埃尔·德·费马 、罗贝瓦尔的吉尔 埃万杰利斯塔·托里拆利 。 设a=1,x轴上[a,b]两点对应的双曲线线段与原点围成的双曲线扇形 面积为f(b),[c,d]对应的扇形面积为f(d)-f(c),d=bc,即为f(bc)-f(c),当且仅当f(bc)=f(b)+f(c)时,两双曲线扇形面积相等。 J. J. O'Connor; E. F. Robertson, The number e , The MacTutor History of Mathematics archive, September 2001 [2009-02-02 ] , (原始内容 存档于2012-02-19)
卡瓦列里弓形面积公式,对于负数值的n (x 的负数幂),由于在x = 0处有个奇点 ,因此定积分的下限为1,而不是0,即为:
∫
1
a
x
n
d
x
=
1
n
+
1
(
a
n
+
1
−
1
)
n
≠
−
1.
{\displaystyle \int _{1}^{a}x^{n}\,dx={\tfrac {1}{n+1}}(a^{n+1}-1)\qquad n\neq -1.}
欧拉 的自然对数定义:
ln
(
x
)
=
lim
n
→
∞
n
(
x
1
/
n
−
1
)
=
lim
n
→
−
1
1
n
+
1
(
x
n
+
1
−
1
)
{\displaystyle {\begin{aligned}\ln(x)&=\lim _{n\rightarrow \infty }n(x^{1/n}-1)\\&=\lim _{n\rightarrow -1}{\tfrac {1}{n+1}}(x^{n+1}-1)\\\end{aligned}}}
Maor, Eli, e: The Story of a Number, Princeton University Press , 2009, ISBN 978-0-691-14134-3 ,sections 1, 1.Eves, Howard Whitley , An introduction to the history of mathematics, The Saunders series 6th, Philadelphia: Saunders, 1992, ISBN 978-0-03-029558-4Boyer, Carl B. , A History of Mathematics, New York: John Wiley & Sons , 1991, ISBN 978-0-471-54397-8
(
1
+
1
n
)
x
=
(
(
1
+
1
n
)
n
)
x
n
{\displaystyle \left(1+{\frac {1}{n}}\right)^{x}=\left(\left(1+{\frac {1}{n}}\right)^{n}\right)^{\frac {x}{n}}}
Kline, Morris , Calculus: an intuitive and physical approach, Dover books on mathematics, New York: Dover Publications , 1998, ISBN 978-0-486-40453-0Ratcliffe, John, Foundations of Hyperbolic Manifolds , Graduate Texts in Mathematics 149 , Springer: 99, 2006 [2014-03-28 ] , ISBN 9780387331973原始内容 存档于2014-01-12), That the area of a hyperbolic triangle is proportional to its angle defect first appeared in Lambert's monograph Theorie der Parallellinien , which was published posthumously in 1786.
John B. Conway, Functions of one complex variable , 2nd edition, Springer, 1978.
Serge Lang , Complex analysis , 3rd edition, Springer-Verlag, 1993.Gino Moretti, Functions of a Complex Variable , Prentice-Hall, Inc., 1964.
Donald Sarason, Complex function theory (页面存档备份 ,存于互联网档案馆 )
E. T. Whittaker G. N. Watson , A Course in Modern Analysis , fourth edition, Cambridge University Press, 1927.