Subgroup
Subset of a group that forms a group itself From Wikipedia, the free encyclopedia
In group theory, a branch of mathematics, a subset of a group G is a subgroup of G if the members of that subset form a group with respect to the group operation in G.
Formally, given a group G under a binary operation ∗, a subset H of G is called a subgroup of G if H also forms a group under the operation ∗. More precisely, H is a subgroup of G if the restriction of ∗ to H × H is a group operation on H. This is often denoted H ≤ G, read as "H is a subgroup of G".
The trivial subgroup of any group is the subgroup {e} consisting of just the identity element.[1]
A proper subgroup of a group G is a subgroup H which is a proper subset of G (that is, H ≠ G). This is often represented notationally by H < G, read as "H is a proper subgroup of G". Some authors also exclude the trivial group from being proper (that is, H ≠ {e}).[2][3]
If H is a subgroup of G, then G is sometimes called an overgroup of H.
The same definitions apply more generally when G is an arbitrary semigroup, but this article will only deal with subgroups of groups.
Subgroup tests
Suppose that G is a group, and H is a subset of G. For now, assume that the group operation of G is written multiplicatively, denoted by juxtaposition.
- Then H is a subgroup of G if and only if H is nonempty and closed under products and inverses. Closed under products means that for every a and b in H, the product ab is in H. Closed under inverses means that for every a in H, the inverse a−1 is in H. These two conditions can be combined into one, that for every a and b in H, the element ab−1 is in H, but it is more natural and usually just as easy to test the two closure conditions separately.[4]
- When H is finite, the test can be simplified: H is a subgroup if and only if it is nonempty and closed under products. These conditions alone imply that every element a of H generates a finite cyclic subgroup of H, say of order n, and then the inverse of a is an−1.[4]
If the group operation is instead denoted by addition, then closed under products should be replaced by closed under addition, which is the condition that for every a and b in H, the sum a + b is in H, and closed under inverses should be edited to say that for every a in H, the inverse −a is in H.
Basic properties of subgroups
- The identity of a subgroup is the identity of the group: if G is a group with identity eG, and H is a subgroup of G with identity eH, then eH = eG.
- The inverse of an element in a subgroup is the inverse of the element in the group: if H is a subgroup of a group G, and a and b are elements of H such that ab = ba = eH, then ab = ba = eG.
- If H is a subgroup of G, then the inclusion map H → G sending each element a of H to itself is a homomorphism.
- The intersection of subgroups A and B of G is again a subgroup of G.[5] For example, the intersection of the x-axis and y-axis in under addition is the trivial subgroup. More generally, the intersection of an arbitrary collection of subgroups of G is a subgroup of G.
- The union of subgroups A and B is a subgroup if and only if A ⊆ B or B ⊆ A. A non-example: is not a subgroup of because 2 and 3 are elements of this subset whose sum, 5, is not in the subset. Similarly, the union of the x-axis and the y-axis in is not a subgroup of
- If S is a subset of G, then there exists a smallest subgroup containing S, namely the intersection of all of subgroups containing S; it is denoted by ⟨S⟩ and is called the subgroup generated by S. An element of G is in ⟨S⟩ if and only if it is a finite product of elements of S and their inverses, possibly repeated.[6]
- Every element a of a group G generates a cyclic subgroup ⟨a⟩. If ⟨a⟩ is isomorphic to (the integers mod n) for some positive integer n, then n is the smallest positive integer for which an = e, and n is called the order of a. If ⟨a⟩ is isomorphic to then a is said to have infinite order.
- The subgroups of any given group form a complete lattice under inclusion, called the lattice of subgroups. (While the infimum here is the usual set-theoretic intersection, the supremum of a set of subgroups is the subgroup generated by the set-theoretic union of the subgroups, not the set-theoretic union itself.) If e is the identity of G, then the trivial group {e} is the minimum subgroup of G, while the maximum subgroup is the group G itself.

Cosets and Lagrange's theorem
Summarize
Perspective
Given a subgroup H and some a in G, we define the left coset aH = {ah : h in H}. Because a is invertible, the map φ : H → aH given by φ(h) = ah is a bijection. Furthermore, every element of G is contained in precisely one left coset of H; the left cosets are the equivalence classes corresponding to the equivalence relation a1 ~ a2 if and only if is in H. The number of left cosets of H is called the index of H in G and is denoted by [G : H].
Lagrange's theorem states that for a finite group G and a subgroup H,
where |G| and |H| denote the orders of G and H, respectively. In particular, the order of every subgroup of G (and the order of every element of G) must be a divisor of |G|.[7][8]
Right cosets are defined analogously: Ha = {ha : h in H}. They are also the equivalence classes for a suitable equivalence relation and their number is equal to [G : H].
If aH = Ha for every a in G, then H is said to be a normal subgroup. Every subgroup of index 2 is normal: the left cosets, and also the right cosets, are simply the subgroup and its complement. More generally, if p is the lowest prime dividing the order of a finite group G, then any subgroup of index p (if such exists) is normal.
Example: Subgroups of Z8
Let G be the cyclic group Z8 whose elements are
and whose group operation is addition modulo 8. Its Cayley table is
| + | 0 | 4 | 2 | 6 | 1 | 5 | 3 | 7 |
|---|---|---|---|---|---|---|---|---|
| 0 | 0 | 4 | 2 | 6 | 1 | 5 | 3 | 7 |
| 4 | 4 | 0 | 6 | 2 | 5 | 1 | 7 | 3 |
| 2 | 2 | 6 | 4 | 0 | 3 | 7 | 5 | 1 |
| 6 | 6 | 2 | 0 | 4 | 7 | 3 | 1 | 5 |
| 1 | 1 | 5 | 3 | 7 | 2 | 6 | 4 | 0 |
| 5 | 5 | 1 | 7 | 3 | 6 | 2 | 0 | 4 |
| 3 | 3 | 7 | 5 | 1 | 4 | 0 | 6 | 2 |
| 7 | 7 | 3 | 1 | 5 | 0 | 4 | 2 | 6 |
This group has two nontrivial subgroups: ■ J = {0, 4} and ■ H = {0, 4, 2, 6} , where J is also a subgroup of H. The Cayley table for H is the top-left quadrant of the Cayley table for G; The Cayley table for J is the top-left quadrant of the Cayley table for H. The group G is cyclic, and so are its subgroups. In general, subgroups of cyclic groups are also cyclic.[9]
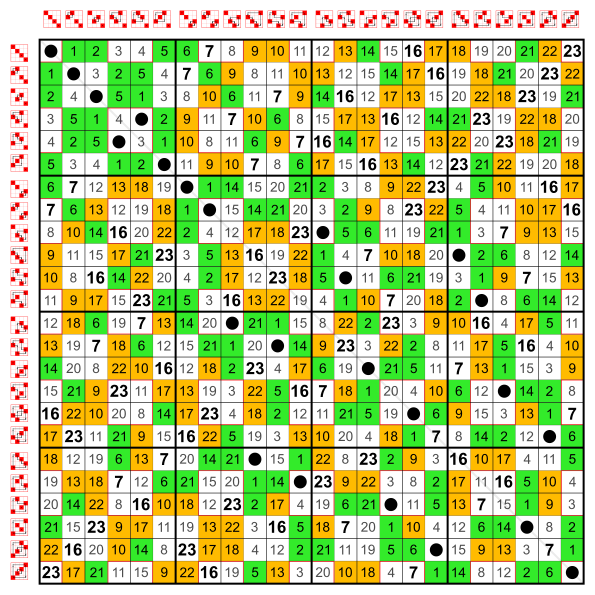
Example: Subgroups of S4
Summarize
Perspective
S4 is the symmetric group whose elements correspond to the permutations of 4 elements.
Below are all its subgroups, ordered by cardinality.
Each group (except those of cardinality 1 and 2) is represented by its Cayley table.
24 elements
Like each group, S4 is a subgroup of itself.
 |
|
12 elements
The alternating group contains only the even permutations.
It is one of the two nontrivial proper normal subgroups of S4. (The other one is its Klein subgroup.)

Subgroups:

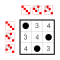

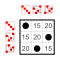
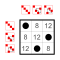
8 elements
 Subgroups:   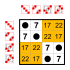 |  Subgroups:    |  Subgroups:    |
6 elements
 Subgroup:  |  Subgroup:  |  Subgroup:  |  Subgroup:  |
4 elements
 |  |  |  (normal subgroup) |
 |  |  |
3 elements
 | 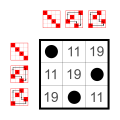 |  |
 |
2 elements
Each permutation p of order 2 generates a subgroup {1, p}.
These are the permutations that have only 2-cycles:
- There are the 6 transpositions with one 2-cycle. (green background)
- And 3 permutations with two 2-cycles. (white background, bold numbers)
1 element
The trivial subgroup is the unique subgroup of order 1.
Other examples
- The even integers form a subgroup of the integer ring the sum of two even integers is even, and the negative of an even integer is even.
- An ideal in a ring R is a subgroup of the additive group of R.
- A linear subspace of a vector space is a subgroup of the additive group of vectors.
- In an abelian group, the elements of finite order form a subgroup called the torsion subgroup.
See also
Notes
References
Wikiwand - on
Seamless Wikipedia browsing. On steroids.









![{\displaystyle [G:H]={|G| \over |H|}}](http://wikimedia.org/api/rest_v1/media/math/render/svg/0e64b76d0605e6c7891273e65da28b5e431d4ea4)




