热门问题
时间线
聊天
视角
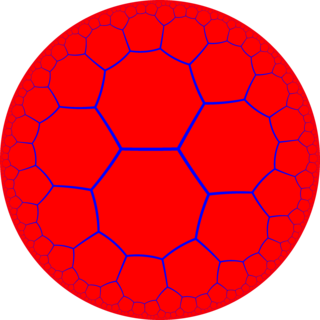
正八邊形鑲嵌
来自维基百科,自由的百科全书
Remove ads
在幾何學中,正八邊形鑲嵌(英語:Octagonal tiling)是一種由正八邊形拼合,並且將正八邊形重複排列組合,並讓圖形完全拼合,而且沒有空隙或重疊的幾何構造,每個頂點皆為三個正八邊形的公共頂點,以頂點圖8.8.8或83表示。
正八邊形鑲嵌是一種雙曲正鑲嵌,在施萊夫利符號中用{8,3}表示。
Remove ads
表面塗色
就如同平面上的正六邊形鑲嵌,正八邊形鑲嵌也具有3種不同的半正表面塗色,都可以由威佐夫結構面對稱構造出来。(h,k)表示一種表面塗色的面周期性重複,以正八邊形距離h、k計數,h在前、k在後。
相關多面體及鑲嵌
 {8,2} |
 {8,3} |
 {8,4} |
 {8,5} |
 {8,6} |
 {8,7} |
 {8,8} |
... |  {8,∞} |
Remove ads
參見
參考文獻
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 19, The Hyperbolic Archimedean Tessellations)
- Chapter 10: Regular honeycombs in hyperbolic space. The Beauty of Geometry: Twelve Essays. Dover Publications. 1999. ISBN 0-486-40919-8. LCCN 99035678.
外部連結
- 埃里克·韦斯坦因. Hyperbolic tiling. MathWorld.
- 埃里克·韦斯坦因. Poincaré hyperbolic disk. MathWorld.
- Hyperbolic and Spherical Tiling Gallery (页面存档备份,存于互联网档案馆)
- KaleidoTile 3: Educational software to create spherical, planar and hyperbolic tilings (页面存档备份,存于互联网档案馆)
- Hyperbolic Planar Tessellations, Don Hatch (页面存档备份,存于互联网档案馆)
Wikiwand - on
Seamless Wikipedia browsing. On steroids.
Remove ads