外觀數列 (Look-and-say sequence),又被稱為莫里斯數列 (Morris number sequence)、螞蟻數列 ,其第n 項描述了第n -1項的數字分布。它以1開始:
一、1:讀作「1個1」,即11
二、11:讀作「2個1」,即21
三、21:讀作「1個2、1個1」,即1211
四、1211:讀作「1個1、1個2、2個1」,即111221
五、111221:讀作「3個1、2個2、1個1」,即312211 1, 11, 21, 1211, 111221, 312211, 13112221, 1113213211, ... (OEIS 數列A005150 ) 如果從 0 至 9 中的任選一個d 數字生成這個數列,那么可以確定d 會保留在每一項的最后一位,如果d 不是1的話,那么這個數列是:
d , 1d , 111d , 311d , 13211d , 111312211d , 31131122211d , …伊蘭·瓦爾迪把 d = 3 時的數列稱為康威數列 [ 1] OEIS 數列A006715 )。(d = 2 時的數列見A006751
2,12,1112,3112,132112,1113122112,...
3,13,1113,3113,132113,1113122113,...
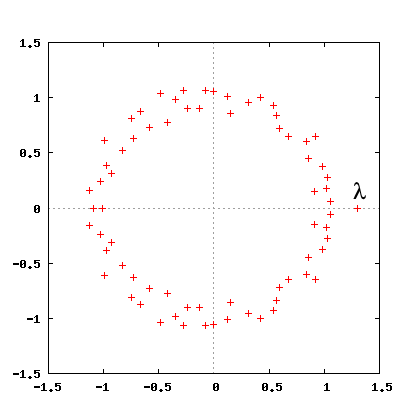
画在复平面 上的康威多项式的根 。最右处标注λ的实根为康威常数。 除了1,2,3之外,沒有其他數字,除非初始的種子使用了其他數字,或者初始種子包含連續三個以上的相同數字。
這個數列的增長是无界的。但是如果使用 22 來生成這個數列,可以得到一個退化 的數列:22, 22, 22, 22, ... (OEIS 數列A010861 )
每生成下一項,數字大約增大30%。設
L
i
{\displaystyle L_{i}}
i
{\displaystyle i}
L
i
+
1
L
i
→
λ
{\displaystyle {\frac {L_{i+1}}{L_{i}}}\rightarrow \lambda }
其中
λ
=
1.303577269034296
…
{\displaystyle \lambda =1.303577269034296\ldots }
OEIS 數列A014715 )稱為康威常數
x
71
−
x
69
−
2
x
68
−
x
67
+
2
x
66
+
2
x
65
+
x
64
−
x
63
−
x
62
−
x
61
−
x
60
−
x
59
+
{\displaystyle x^{71}-x^{69}-2x^{68}-x^{67}+2x^{66}+2x^{65}+x^{64}-x^{63}-x^{62}-x^{61}-x^{60}-x^{59}+\,}
2
x
58
+
5
x
57
+
3
x
56
−
2
x
55
−
10
x
54
−
3
x
53
−
2
x
52
+
6
x
51
+
6
x
50
+
x
49
+
9
x
48
−
3
x
47
−
{\displaystyle 2x^{58}+5x^{57}+3x^{56}-2x^{55}-10x^{54}-3x^{53}-2x^{52}+6x^{51}+6x^{50}+x^{49}+9x^{48}-3x^{47}-\,}
7
x
46
−
8
x
45
−
8
x
44
+
10
x
43
+
6
x
42
+
8
x
41
−
5
x
40
−
12
x
39
+
7
x
38
−
7
x
37
+
7
x
36
+
x
35
−
{\displaystyle 7x^{46}-8x^{45}-8x^{44}+10x^{43}+6x^{42}+8x^{41}-5x^{40}-12x^{39}+7x^{38}-7x^{37}+7x^{36}+x^{35}-\,}
3
x
34
+
10
x
33
+
x
32
−
6
x
31
−
2
x
30
−
10
x
29
−
3
x
28
+
2
x
27
+
9
x
26
−
3
x
25
+
14
x
24
−
8
x
23
−
{\displaystyle 3x^{34}+10x^{33}+x^{32}-6x^{31}-2x^{30}-10x^{29}-3x^{28}+2x^{27}+9x^{26}-3x^{25}+14x^{24}-8x^{23}-\,}
7
x
21
+
9
x
20
+
3
x
19
−
4
x
18
−
10
x
17
−
7
x
16
+
12
x
15
+
7
x
14
+
2
x
13
−
12
x
12
−
4
x
11
−
{\displaystyle 7x^{21}+9x^{20}+3x^{19}-4x^{18}-10x^{17}-7x^{16}+12x^{15}+7x^{14}+2x^{13}-12x^{12}-4x^{11}-\,}
2
x
10
+
5
x
9
+
x
7
−
7
x
6
+
7
x
5
−
4
x
4
+
12
x
3
−
6
x
2
+
3
x
−
6
=
0
{\displaystyle 2x^{10}+5x^{9}+x^{7}-7x^{6}+7x^{5}-4x^{4}+12x^{3}-6x^{2}+3x-6=0\,}
這個數列最初出現在約翰·何頓·康威 1986年論文 The Weird and Wonderful Chemistry of Audioactive Decay [ 2] Open Problems in Communication and Computation ISBN 0-387-96621-8 )。它的靈感來自壓縮方法RLE (Run-length encoding)。
莫里斯數列得名於密碼學家羅伯特·莫里斯
![]() A006751)
A006751)