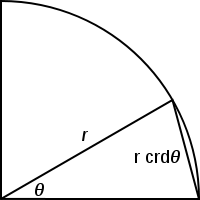
最早已知的弦函數表由喜帕恰斯 編制,其列出了每7+ 1 / 2 度的弦函數值 。
在公元二世紀,亞歷山大的托勒密 在他的天文學書《天文學大成 》中編制了弦函數的函數表——托勒密全弦表 ,其給出了從1 / 2 1 / 2 [ 10] 托勒密全弦表 所列出的值是弦函數的60倍,例如
crd
60
∘
=
1
{\displaystyle \operatorname {crd} 60^{\circ }=1}
[ 12]
弦函數與現代常用的正弦函數之關係可以看做是正弦函數代入半角公式 的結果。
crd
θ
=
2
sin
(
θ
2
)
{\displaystyle \operatorname {crd} \ \theta =2\sin \left({\frac {\theta }{2}}\right)}
[ 13] [ 註 1] 上述等式只成立於0 < θ ≤ π 0 < θ ≤ 180
正如現代三角學是建立在正弦函數的基礎上一樣,古代三角學也是建立在和弦函數的基礎上。
據說喜帕恰斯寫了一本十二卷的關於弦函數的著作,雖然現在全部都失傳了,但想必人們對弦函數有一定的了解。[ 15]
托勒密所建立的托勒密全弦表 是紀錄特定圓心角θ °
chord
(
θ
)
{\displaystyle \operatorname {chord} (\theta )}
[ 16] [ 17]
chord
(
θ
)
=
120
sin
(
θ
∘
2
)
=
60
⋅
(
2
sin
(
π
θ
∘
360
radians
)
)
.
{\displaystyle {\begin{aligned}&\operatorname {chord} (\theta )=120\sin \left({\frac {\theta ^{\circ }}{2}}\right)\\={}&60\cdot \left(2\,\sin \left({\frac {\pi \theta ^{\circ }}{360}}{\text{ radians}}\right)\right).\end{aligned}}}
d
d
θ
crd
(
θ
)
=
cos
(
θ
2
)
{\displaystyle {\frac {\mathrm {d} }{\mathrm {d} \theta }}\operatorname {crd} (\theta )=\cos \left({\frac {\theta }{2}}\right)}
0 < θ ≤ π [ 註 2]
d
d
θ
crd
(
θ
)
=
sin
(
θ
)
2
−
2
cos
(
θ
)
=
2
sin
(
θ
)
(
1
−
cos
(
θ
)
)
+
2
sin
(
θ
)
cos
(
θ
)
2
sin
2
(
θ
)
+
(
1
−
cos
(
θ
)
)
2
{\displaystyle {\frac {\mathrm {d} }{\mathrm {d} \theta }}\operatorname {crd} (\theta )={\frac {\sin(\theta )}{\sqrt {2-2\cos(\theta )}}}={\frac {2\sin(\theta )(1-\cos(\theta ))+2\sin(\theta )\cos(\theta )}{2{\sqrt {\sin ^{2}(\theta )+(1-\cos(\theta ))^{2}}}}}}
∫
crd
(
θ
)
d
θ
=
−
4
cos
(
θ
2
)
+
C
{\displaystyle \int \operatorname {crd} (\theta )\,\mathrm {d} \theta =-4\cos \left({\frac {\theta }{2}}\right)+C}
0 < θ ≤ π [ 註 2]
∫
crd
(
θ
)
d
θ
=
−
2
2
−
2
cos
(
θ
)
cot
(
θ
2
)
+
C
{\displaystyle \int \operatorname {crd} (\theta )\,\mathrm {d} \theta =-2{\sqrt {2-2\cos(\theta )}}\cot \left({\frac {\theta }{2}}\right)+C}
crd
(
0
)
=
0
{\displaystyle \operatorname {crd} \left(0\right)=0}
crd
(
π
15
)
=
crd
(
12
∘
)
=
30
−
6
5
−
6
+
2
5
4
{\displaystyle \operatorname {crd} \left({\frac {\pi }{15}}\right)=\operatorname {crd} \left(12^{\circ }\right)={\frac {{\sqrt {30-6{\sqrt {5}}}}-{\sqrt {6+2{\sqrt {5}}}}}{4}}}
[ 13]
crd
(
π
5
)
=
crd
(
36
∘
)
=
5
−
1
2
{\displaystyle \operatorname {crd} \left({\frac {\pi }{5}}\right)=\operatorname {crd} \left(36^{\circ }\right)={\frac {{\sqrt {5}}-1}{2}}}
[ 13]
crd
(
π
3
)
=
crd
(
60
∘
)
=
1
{\displaystyle \operatorname {crd} \left({\frac {\pi }{3}}\right)=\operatorname {crd} \left(60^{\circ }\right)=1}
[ 13]
crd
(
2
π
5
)
=
crd
(
72
∘
)
=
10
−
2
5
2
{\displaystyle \operatorname {crd} \left({\frac {2\pi }{5}}\right)=\operatorname {crd} \left(72^{\circ }\right)={\frac {10-2{\sqrt {5}}}{2}}}
[ 13]
crd
(
π
2
)
=
crd
(
90
∘
)
=
2
{\displaystyle \operatorname {crd} \left({\frac {\pi }{2}}\right)=\operatorname {crd} \left(90^{\circ }\right)={\sqrt {2}}}
[ 13]
crd
(
3
π
5
)
=
crd
(
108
∘
)
=
6
+
2
5
2
{\displaystyle \operatorname {crd} \left({\frac {3\pi }{5}}\right)=\operatorname {crd} \left(108^{\circ }\right)={\frac {6+2{\sqrt {5}}}{2}}}
[ 13]
crd
(
2
π
3
)
=
crd
(
120
∘
)
=
3
{\displaystyle \operatorname {crd} \left({\frac {2\pi }{3}}\right)=\operatorname {crd} \left(120^{\circ }\right)={\sqrt {3}}}
[ 13]
crd
(
4
π
5
)
=
crd
(
144
∘
)
=
10
+
2
5
2
{\displaystyle \operatorname {crd} \left({\frac {4\pi }{5}}\right)=\operatorname {crd} \left(144^{\circ }\right)={\frac {10+2{\sqrt {5}}}{2}}}
[ 13]
crd
(
π
)
=
crd
(
180
∘
)
=
2
{\displaystyle \operatorname {crd} \left(\pi \right)=\operatorname {crd} \left(180^{\circ }\right)=2}
弦函數的反函數 弦函數的反函數可以定義如下:
crd
−
1
y
=
2
arcsin
y
2
{\displaystyle \operatorname {crd} ^{-1}\ y=2\arcsin {\frac {y}{2}}}
在這定義下只有0 ≤ y ≤ 2
或者用
crd
θ
=
2
−
2
cos
θ
{\displaystyle \operatorname {crd} \ \theta ={\sqrt {2-2\cos \theta }}}
crd
−
1
y
=
arccos
2
−
y
2
2
{\displaystyle \operatorname {crd} ^{-1}\ y=\arccos {\frac {2-y^{2}}{2}}}
反弦函數有時會簡稱為acrd[ 6]
因此已知弦長 可以回推圓心角的角度:
[ 21]
θ
=
2
arcsin
c
2
r
{\displaystyle \theta =2\arcsin {\frac {c}{2r}}}
其中,其中c是弦長、r是圓的半徑。
正弦(sin)、餘弦(cos)、全弦(crd)、正矢(versin)、和半正矢(haversin)函數的函數圖形 弦函數的值域 範圍在0到2之間,類似的函數還有正矢函數 (versin),值域範圍也在0到2之間,但函數圖形 略有差異。
弦函數在範圍0到π 正弦函數 0到π / 2 正弦 、正切 、正割 、正矢 )同樣是從零開始遞增的函數。
弧長(arc θ crd θ sin θ 與弦函數(crd θ arc θ θ 圓弧 之弧長 [ 5] 這個概念有時稱為正弧 [ 22] ,對應於割圓八線 中正角的弧 arc θ crd θ [ 5] arc θ
arc θ crd θ
在單位圓上,arc θ 2π θ 2π 最小正同界角 的弧度值。
割圓八線 。正角對應的弧為正弧(乙丙)、餘角對應的弧為餘弧(乙庚)相同的概念在割圓八線 中也存在。
arc θ 正弧 」,即正角(θ arc θ 也存在對應的餘弧(coarc θ 直角 與正角之差所對應的弧[ 23] coarc θ = arc(π / 2 θ ) [ 22] [ 24] [ 24]
事實上,將crd函數定義為
crd
θ
=
2
sin
(
θ
2
)
{\displaystyle \operatorname {crd} \ \theta =2\sin \left({\frac {\theta }{2}}\right)}
2
sin
(
θ
2
)
{\displaystyle 2\sin \left({\frac {\theta }{2}}\right)}
0 < θ ≤ π 0 < θ ≤ 180 此等式是使用
crd
θ
=
2
sin
(
θ
2
)
{\displaystyle \operatorname {crd} \ \theta =2\sin \left({\frac {\theta }{2}}\right)}
[ 註 1] 0 < θ ≤ π 0 < θ ≤ 180
Folkerts, Menso and Launert, Dieter and Thom, Andreas. Jost Bürgi's method for calculating sines. Historia mathematica (Elsevier). 2016, 43 (2): 133–147.
Ganikhodjaev, Nasir and Husain, Ahmad Hamidi. Al-Kāshī, the first scientist who introduced dynamical system. AIP Conference Proceedings (AIP Publishing). 2018, 1974 (1).
Simpson, David G. AUXTRIG (FORTRAN-90 source code). Greenbelt, Maryland, USA: NASA Goddard Space Flight Center. 2001-11-08 [2015-10-26 ] . (原始内容存档 于2008-06-16). 方愷. 平三角附測量 (PDF) . 代數通藝錄 (成文堂刊本). 1890, 卷十三 [2023-11-06 ] . (原始内容存档 (PDF) 于2023-03-26).