热门问题
时间线
聊天
视角
抛物柱面坐标系
来自维基百科,自由的百科全书
Remove ads
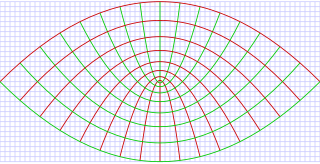
抛物柱面坐标系(英语:Parabolic cylindrical coordinates)是一种三维正交坐标系。往 z-轴方向延伸二维的抛物线坐标系 ,则可得到抛物柱面坐标系。其坐标曲面是共焦的抛物柱面。抛物柱面坐标可以应用于许多物理问题。例如,物体边缘的位势论。

Remove ads
基本定义
直角坐标 可以用抛物柱面坐标 表示为
- 、
- 、
- ;
其中, , 。
- ,
而坐标 为常数的曲线形成共焦的,凹性往 -y-轴的抛物柱面:
- 。
这些抛物柱面的焦线的位置都在 z-轴。
径向距 的公式为
- 。
当解析经典力学的反平方连心力问题时,假若采用抛物柱面坐标的哈密顿-亚可比方程式,则会用到这很有用的公式。参阅 拉普拉斯-龙格-冷次向量[锚点失效]。
Remove ads
标度因子
抛物柱面坐标 与 的标度因子相等;而 的标度因子是 1 :
- 、
- 。
无穷小体积元素是
- 。
- 。
其它微分算子,像 、 ,都可以用 坐标表示,只要将标度因子代入在正交坐标系条目内对应的一般公式。
Remove ads
应用
抛物柱面坐标有一个经典的应用,这是在解析像拉普拉斯方程或亥姆霍兹方程这类的偏微分方程式。在这些方程式里,抛物柱面坐标允许分离变数法的使用。个典型的例题是,有一块半无限的平板导体,请问其周围的电场为什么?应用抛物柱面坐标,我们可以精致地分析这例题。
参阅
参考文献
- Philip M. Morse, Herman Feshbach. Methods of Theoretical Physics, Part I. New York: McGraw-Hill. 1953: p. 658. ISBN 0-07-043316-X.
- Henry Margenau, Murphy GM. The Mathematics of Physics and Chemistry. New York: D. van Nostrand. 1956: pp. 186–187.
- Korn GA, Korn TM. Mathematical Handbook for Scientists and Engineers. New York: McGraw-Hill. 1961: p. 181. ASIN B0000CKZX7.
- Sauer R, Szabó I. Mathematische Hilfsmittel des Ingenieurs. New York: Springer Verlag. 1967: p. 96.
- Zwillinger D. Handbook of Integration. Boston, MA: Jones and Bartlett. 1992: p. 114. ISBN 0-86720-293-9. Same as Morse & Feshbach (1953), substituting uk for ξk。
- Moon P, Spencer DE. Parabolic-Cylinder Coordinates (μ, ν, z). Field Theory Handbook, Including Coordinate Systems, Differential Equations, and Their Solutions corrected 2nd ed., 3rd print ed. New York: Springer-Verlag. 1988: pp. 21–24 (Table 1.04). ISBN 978-0387184302.
Remove ads
外部链接
Wikiwand - on
Seamless Wikipedia browsing. On steroids.
Remove ads
























