中點
来自维基百科,自由的百科全书
中點是線段上與兩端點距離相等的一點。 在直角座標系中,若兩端點的座標分別為、,則中點的座標為:
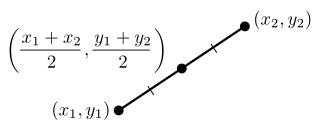
在n度空間中,若兩點的座標分別為、,則中點的座標為 :
中點尺規作圖
利用直尺和圓規,可以畫出一個線段的中點。步驟如下:
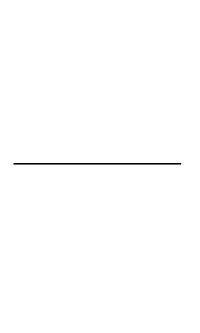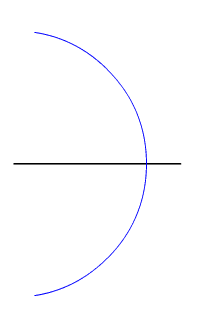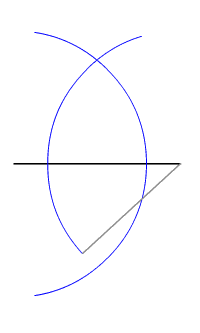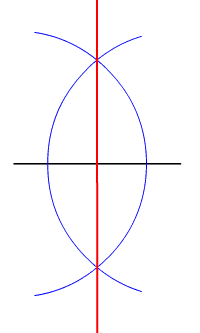
事實上只需要兩個圓的半徑相等,並且都大於線段長度的一半就可以了。
參見
| 這是一篇關於幾何學的小作品。您可以透過編輯或修訂擴充其內容。 |
Wikiwand - on
Seamless Wikipedia browsing. On steroids.
来自维基百科,自由的百科全书
中點是線段上與兩端點距離相等的一點。 在直角座標系中,若兩端點的座標分別為、,則中點的座標為:

在n度空間中,若兩點的座標分別為、,則中點的座標為 :
利用直尺和圓規,可以畫出一個線段的中點。步驟如下:
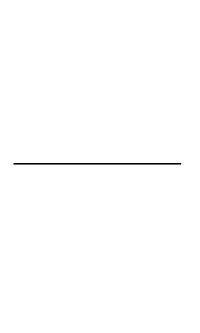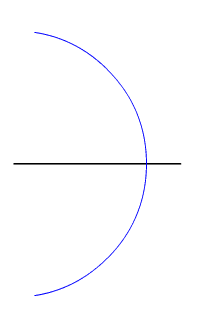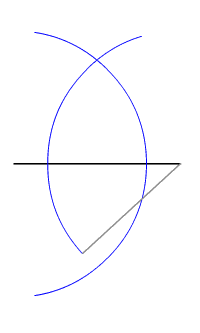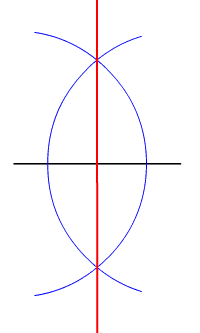
事實上只需要兩個圓的半徑相等,並且都大於線段長度的一半就可以了。
| 這是一篇關於幾何學的小作品。您可以透過編輯或修訂擴充其內容。 |
Seamless Wikipedia browsing. On steroids.