線性代數基本定理

線性代數基本定理是秩為 r 的 m×n 矩陣A的奇異值分解:

${\displaystyle A=U\Sigma V^{T}\ }$

對於矩陣${\displaystyle A\in \mathbf {R} ^{m\times n}}$ (${\displaystyle A}$有${\displaystyle m}$行及${\displaystyle n}$列)產生了四個基本線性子空間:

子空間名字	定義	包含於	維數	基
列空間、值域或像	${\displaystyle \mathrm {im} (A)}$ 或 ${\displaystyle \mathrm {range} (A)}$	${\displaystyle \mathbf {R} ^{m}}$	${\displaystyle r}$	${\displaystyle \mathbf {U} }$ 的前 ${\displaystyle r}$ 列
左零空間或上核	${\displaystyle \mathrm {ker} (A^{T})}$ 或 ${\displaystyle \mathrm {null} (A^{T})}$	${\displaystyle \mathbf {R} ^{m}}$	${\displaystyle m-r}$	${\displaystyle \mathbf {U} }$ 的後 ${\displaystyle m-r}$ 列
行空間或余象	${\displaystyle \mathrm {im} (A^{T})}$ 或 ${\displaystyle \mathrm {range} (A^{T})}$	${\displaystyle \mathbf {R} ^{n}}$	${\displaystyle r}$	${\displaystyle \mathbf {V} }$ 的前 ${\displaystyle r}$ 列
零空間或核	${\displaystyle \mathrm {ker} (A)}$ 或 ${\displaystyle \mathrm {null} (A)}$	${\displaystyle \mathbf {R} ^{n}}$	${\displaystyle n-r}$	${\displaystyle \mathbf {V} }$ 的後 ${\displaystyle n-r}$ 列

Secondly:

1. In ${\displaystyle \mathbf {R} ^{n}}$, ${\displaystyle \mathrm {ker} (A)=(\mathrm {im} (A^{T}))^{\perp }}$, 也就是, 零空間與行空間的正交補相同.
2. In ${\displaystyle \mathbf {R} ^{m}}$, ${\displaystyle \mathrm {ker} (A^{T})=(\mathrm {im} (A))^{\perp }}$, 也就是, 左零空間為列空間的正交補.

矩陣A的四個基本子空間.

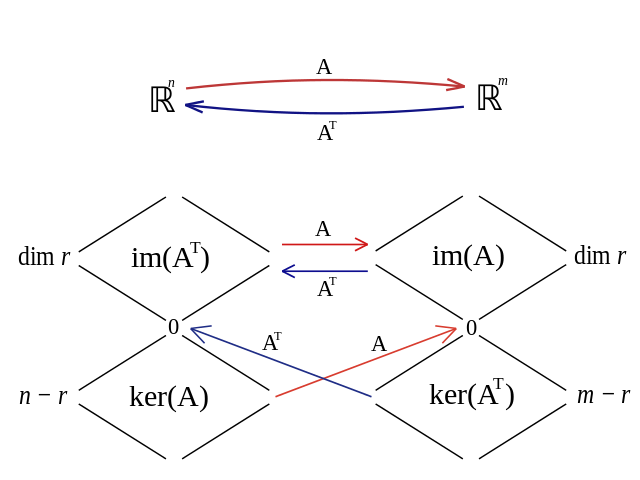
子空間的維數遵從秩-零化度定理.

進一步, 所有這些空間本質地定義於– 不必考慮基的選擇 – 抽象向量空間, 算子, 對偶空間${\displaystyle A\colon V\to W}$ 與${\displaystyle A^{*}\colon W^{*}\to V^{*}}$: ${\displaystyle A^{*}}$的核與像是${\displaystyle A}$的上核與余象.

參見

• 秩－零化度定理
• 閉值域定理

參考文獻

• Strang, Gilbert. Linear Algebra and Its Applications. 3rd ed. Orlando: Saunders, 1988.
• Strang, Gilbert, The fundamental theorem of linear algebra (PDF), American Mathematical Monthly, 1993, 100 (9): 848–855, JSTOR 2324660, doi:10.2307/2324660^{[失效連結]}

外部連結

• Gilbert Strang, MIT Linear Algebra Lecture on the Four Fundamental Subspaces （頁面存檔備份，存於互聯網檔案館）, from MIT OpenCourseWare