环带多面体

环带多面体 （全对称多面体）是一种每个面都相对称、相等或与正对的（即将两个面的中心连起可过内接球或外接球球心）面互相对称的立体。

环带多面体对于空间的填充

由闵可夫斯基和构成环带多面体

排列构成环状多面体

环带多面体的种类

• 截角八面体有6个正方形面和8个六边形面。
• 大斜方截半立方体有12个正方形面、8个六面形面和6个八边形面。
• 大斜方截半二十面体有30个正方形面、20个六边形面和12个十边形面。

另外，某些卡塔兰立体（半正多面体的对偶多面体）也同样是环带多面体：

• 菱形十二面体是截半立方体的对偶多面体。
• 菱形三十面体是截半二十面体的对偶多面体。

其他有菱形面的环带多面体：

• 菱形二十面体
• 菱面体
• 菱形九十面体

名称(环带多面体)	立体图示	对称群	正多边形面	面 的可递性	边 的可递性	顶点的可递性	空间填充(space filling)	Number of generators
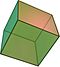
正方体 4.4.4		Oh群	有	有	有	有	有	3
六角柱 4.4.6		D6h群	有	无	无	有	有	4
棱柱 4.4.2n ${\displaystyle (n>3)}$		Dnh群	有	无	无	有	无	n+1
截角八面体 4.6.6		Oh群	有	无	无	有	有
大斜方截半立方体 4.6.8		Oh群	有	无	无	有	无
大斜方截半二十面体 4.6.10		Ih群	有	无	无	有	无
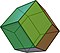
菱形十二面体 V3.4.3.4		Oh群	无	有	有	无	有
菱形三十面体 V3.5.3.5		Ih群	无	有	有	无	无	6
菱形六角化十二面体		D4h群	无	无	无	无	有	5
截角菱形十二面体		Oh群	无	无	无	无	无

环带多面体的分解

虽然多面体通不常能以相同的体积分解、组合成其他多面体(请参考希尔伯特第三问题)。 但任两个环带多面体却得以同体积被切割、重新组合成另一方。

参考资料

• Coxeter, H. S. M. The Classification of Zonohedra by Means of Projective Diagrams. J. Math. Pures Appl. 1962, 41: 137–156.
• Eppstein, David. Zonohedra and zonotopes. Mathematica in Education and Research. 1996, 5 (4): 15–21 [2007-12-07]. （原始内容存档于2021-01-04）.
• Grünbaum, Branko. Arrangements and Spreads. Number 10 in Regional Conf. Series in Mathematics, 美国数学学会. 1972.
• Fedorov, E. S. Elemente der Gestaltenlehre. Zeitschrift für Krystallographie und Mineralogie. 1893, 21: 671–694.
• Shephard, G. C. Space-filling zonotopes. Mathematika（英语：Mathematika）. 1974, 21: 261–269.
• Taylor, Jean E. Zonohedra and generalized zonohedra. 美国数学月刊. 1992, 99: 108–111. doi:10.2307/2324178.

外部链接

• 埃里克·韦斯坦因. Zonohedron. MathWorld.
• Eppstein, David. The Geometry Junkyard: Zonohedra and Zonotopes. [2007-12-07]. （原始内容存档于2020-03-16）.
• Hart, George W. Virtual Polyhedra: Zonohedra. [2007-12-07]. （原始内容存档于2005-11-18）.
• 埃里克·韦斯坦因. Primary Parallelohedron. MathWorld.
• Bulatov, Vladimir. Zonohedral Polyhedra Completion. [2007-12-07]. （原始内容存档于2011-08-07）.