偶极子

在电磁学里，有两种偶极子（英语：Dipole）：

• 电偶极子是两个分隔一段距离，电量相等，正负相反的电荷。
• 磁偶极子是一圈封闭循环的电流。例如一个有常定电流运行的线圈。

地球磁场可以近似为一个磁偶极子的磁场。但是，图内的 N 和 S 符号分别标示地球的地理北极和地理南极。这标示法很容易引起困惑。实际而言，地球的磁偶极矩的方向，是从地球位于地理北极附近的地磁北极，指向位于地理南极附近的地磁南极；而磁偶极子的方向则是从指南极指向指北极。

电极偶子的等值线图。等值曲面清楚地区分于图内。

偶极子的性质可以用它的偶极矩描述。

电偶极矩（${\displaystyle \mathbf {p} }$）由负电荷指向正电荷，大小等于正电荷量乘以正负电荷之间的距离。磁偶极矩（${\displaystyle \mathbf {m} }$）的方向，根据右手法则，是大拇指从载流回路的平面指出的方向，而其它手指则指向电流运行方向，磁偶极矩的大小等于电流乘以线圈面积。

除了载流回路以外，电子和许多基本粒子都拥有磁偶极矩。它们都会产生磁场，与一个非常小的载流回路产生的磁场完全相同。但是，现时大多数的科学观点认为这个磁偶极矩是电子的自然性质，而非由载流回路生成。

永久磁铁的磁偶极矩来自于电子内禀的磁偶极矩。长条形的永久磁铁称为条形磁铁，其两端称为指北极和指南极，其磁偶极矩的方向是由指南极朝向指北极。这常规与地球的磁偶极矩恰巧相反：地球的磁偶极矩的方向是从地球的地磁北极指向地磁南极。地磁北极位于北极附近，实际上是指南极，会吸引磁铁的指北极；而地磁南极位于南极附近，实际上是指北极，会吸引磁铁的指南极。罗盘磁针的指北极会指向地磁北极；条形磁铁可以当作罗盘使用，条形磁铁的指北极会指向地磁北极。

根据当前的观察结果，磁偶极子产生的机制只有两种，载流回路和量子力学自旋。科学家从未在实验里找到任何磁单极子存在的证据。

物理偶极子、点偶极子、近似偶极子

分开有限距离的两个异性电荷的电场线。

有限直径的载流回圈的磁场线。

任意点偶极子（电偶极子、磁偶极子、声偶极子等等）的场线。

一个物理电偶极子是由两个等电量的异性点电荷构成的。在距离远超于两个点电荷相隔距离之处，物理电偶极子所产生的电场，可以近似为其电偶极矩所产生的电场。令物理电偶极子的两个点电荷相隔距离趋向于 0 ，同时保持其电偶极矩不变，则极限就是点电偶极子，又称为纯电偶极子。物理电偶极子产生的电场的多极展开式中，一次项目就是点电偶极子产生的电场。物理电偶极子的电偶极矩 ${\displaystyle \mathbf {p} }$ 是

${\displaystyle \mathbf {p} =q\mathbf {d} }$ ；

其中，${\displaystyle q}$ 是每个电荷的带电量绝对值，${\displaystyle \mathbf {d} }$ 是从负电荷到正电荷的位移向量。

到现今为止，虽然还没有找到任何磁单极子存在的证据，科学家可以在电子和许多基本粒子的物理行为中，找到以量子力学的自旋形式存在的磁偶极子。点磁偶极子所产生的磁场的形态与点电偶极子所产生的电场的形态完全相同。非常小的载流回路可以近似为点磁偶极子。物理磁偶极子 ${\displaystyle \mathbf {m} }$ 的磁偶极矩是

${\displaystyle \mathbf {m} =I\mathbf {a} }$ ；

其中，${\displaystyle I}$ 是运行于载流回路的电流，${\displaystyle \mathbf {a} }$ 是载流回路的面积向量。

任何电荷或电流组态都具有偶极矩，其对应的偶极子所产生的向量场，是那个组态在远距离的最好近似。偶极子项只是多极展开式中的一项。当单极矩等于 0 时（对磁案例而言，此情况永远成立，因为磁单极子不存在），在远距离 ${\displaystyle r}$ 时，偶极子项（第二项）是最主要的项；其向量场值衰减率为 ${\displaystyle 1/r^{3}}$ ，作为比较，单极矩项的递减率为 ${\displaystyle 1/r^{2}}$ ，第三项的衰减率为 ${\displaystyle 1/r^{4}}$ ，第 ${\displaystyle n}$ 项的递减率为 ${\displaystyle 1/r^{(n+1)}}$ 。

分子的电偶极矩

很多分子都拥有电偶极矩。这是因为正负电荷的不均匀分布。例如，

（正价） H-Cl （负价）

拥有永久电偶极矩的分子称为极化分子。假若一个分子带有感应电偶极子，则称此分子被极化。彼得·德拜是最先研究分子的电偶极子的物理化学家。为了纪念他的贡献，电偶极矩的测量单位被命名为德拜。

分子的电偶极子又分为以下三种（参阅分子间作用力）：

• 永久电偶极子：假若一个分子内的几个原子的电荷分布不均，电负性差异很大，则电负性较大的原子会吸引电子更接近自己，因而使得所占据区域变得更具负性；另外电负性较小的原子的区域会变得更具正性。这样，正、负电荷中心始终不重合，就形成了永久电偶极子。
• 瞬时电偶极子：有时候，电子会恰巧地比较集中于分子内的某一个区域，这偶发状况会产生暂时的电偶极子。
• 感应电偶极子：当施加外电场于一个分子时，感应这外电场的作用，分子内部正常的电子云形状会被改变，因而产生电偶极子。其伴随的电偶极矩等于外电场和极化性的乘积。

常见的化学化合物在气态的电偶极矩，采用德拜单位：^[1]

• 二氧化碳：0
• 一氧化碳：0.112
• 臭氧：0.53
• 光气：1.17
• 水蒸气：1.85
• 氰化氢：2.98
• 氨基氰：4.27
• 溴化钾：10.41

这些数值可从相对电容率的测量值计算求得。当分子因为对称性而使得浄电偶极矩被抵消，则设定电偶极矩为 0 。电偶极矩最大值在 10 到 11 这值域内。知道电偶极矩值，科学家可以推论分子的分子结构。例如，数据显示出，二氧化碳是一个线性分子；而臭氧则不是。

电偶极子的电场

球坐标 ${\displaystyle (r,\ \theta ,\ \phi )}$ 与直角坐标 ${\displaystyle (x,\ y,\ z)}$ 之间的关系。

假设电偶极子 ${\displaystyle \mathbf {p} }$ 的位置是原点 ${\displaystyle \mathbf {O} }$ ，则在任意位置 ${\displaystyle \mathbf {r} }$ ，此电偶极子产生的电势 ${\displaystyle \Phi (\mathbf {r} )}$ 是

${\displaystyle \Phi (\mathbf {r} )={\frac {1}{4\pi \epsilon _{0}}}\,{\frac {\mathbf {p} \cdot {\hat {\mathbf {r} }}}{r^{2}}}}$ ；

其中，${\displaystyle \epsilon _{0}}$ 是真空电容率。

这公式的右手边项目是任意静电势多极展开式的第二个项目。假若这任意静电势是由纯电偶极子产生，则这项目是多极展开式的唯一不消失项目。

电偶极子 ${\displaystyle \mathbf {p} }$ 所产生的电场 ${\displaystyle \mathbf {E} }$ 为

${\displaystyle {\begin{aligned}\mathbf {E} =-\nabla \Phi &={\frac {1}{4\pi \epsilon _{0}r^{3}}}\left(3(\mathbf {p} \cdot {\hat {\mathbf {r} }}){\hat {\mathbf {r} }}-\mathbf {p} \right)\\&={\frac {p}{4\pi \epsilon _{0}r^{3}}}(2\cos \theta {\hat {\mathbf {r} }}+\sin \theta {\hat {\boldsymbol {\theta }}})\end{aligned}}}$ ；

其中，${\displaystyle \theta }$ 是 ${\displaystyle \mathbf {r} }$ 和 ${\displaystyle \mathbf {p} }$ 之间的夹角。

注意到这个方程式并不完全正确，这是因为电偶极子的电势有一个奇点在它所处的位置（原点 ${\displaystyle \mathbf {O} }$ ）。更仔细地推导，可以得到电场为^[2]

${\displaystyle {\begin{aligned}\mathbf {E} =-\nabla \Phi &={\frac {1}{4\pi \epsilon _{0}r^{3}}}\left(3(\mathbf {p} \cdot {\hat {\mathbf {r} }}){\hat {\mathbf {r} }}-\mathbf {p} \right)-{\frac {\mathbf {p} }{3\epsilon _{0}}}\delta ^{3}(\mathbf {r} )\\&={\frac {p}{4\pi \epsilon _{0}r^{3}}}(2\cos \theta {\hat {\mathbf {r} }}+\sin \theta {\hat {\boldsymbol {\theta }}})-{\frac {\mathbf {p} }{3\epsilon _{0}}}\delta ^{3}(\mathbf {r} )\end{aligned}}}$ ；

其中，${\displaystyle \delta ^{3}(\mathbf {r} )}$ 是三维狄拉克δ函数

导引

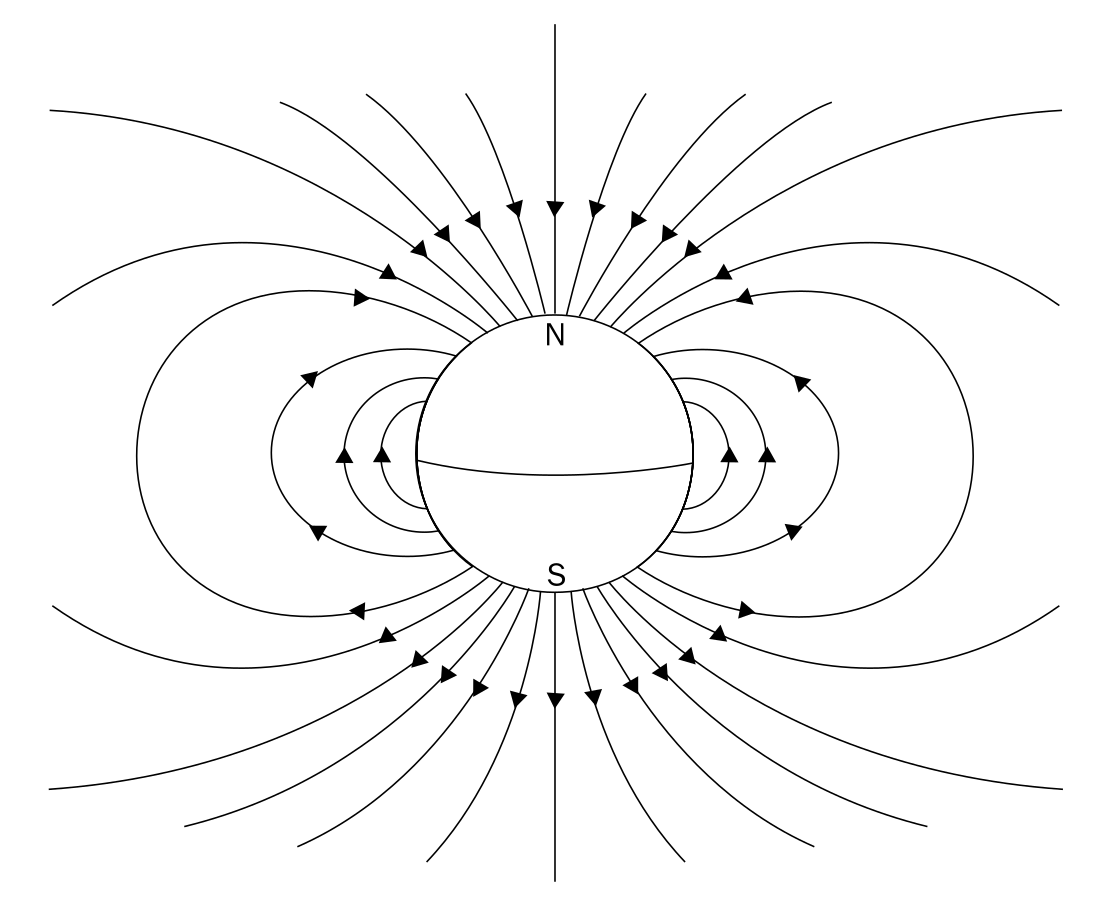
从计算电偶极子所产生的电场的平均值，可以得到正确答案。设定以原点 ${\displaystyle \mathbf {O} }$ 为圆心，半径为 ${\displaystyle b}$ 的球体 ${\displaystyle \mathbb {V} }$ 。电偶极子所产生于这球体的电场，其平均值为：

${\displaystyle \langle \mathbf {E} \rangle ={\frac {3}{4\pi b^{3}}}\int _{\mathbb {V} }\mathbf {E} \mathrm {d} ^{3}\mathbf {r} ={\frac {3}{4\pi b^{3}}}\int _{0}^{b}\int _{0}^{2\pi }\int _{0}^{\pi }{\frac {p}{4\pi \epsilon _{0}r^{3}}}(2\cos \theta {\hat {\mathbf {r} }}+\sin \theta {\hat {\boldsymbol {\theta }}})r^{2}\sin \theta \ \mathrm {d} \theta \mathrm {d} \phi \mathrm {d} r}$ 。

注意到球坐标单位向量与直角坐标单位向量之间的关系：

${\displaystyle {\hat {\mathbf {r} }}={\hat {\mathbf {x} }}\sin \theta \cos \phi +{\hat {\mathbf {y} }}\sin \theta \sin \phi +{\hat {\mathbf {z} }}\cos \theta }$ 、

${\displaystyle {\hat {\boldsymbol {\theta }}}={\hat {\mathbf {x} }}\cos \theta \cos \phi +{\hat {\mathbf {y} }}\cos \theta \sin \phi -{\hat {\mathbf {z} }}\sin \theta }$ 。

将这两个关系式代入前面积分式，可以得到

${\displaystyle \langle \mathbf {E} \rangle }$	${\displaystyle ={\frac {3p}{16\pi ^{2}\epsilon _{0}b^{3}}}\int _{0}^{b}\int _{0}^{2\pi }\int _{0}^{\pi }{\frac {1}{r^{3}}}}$	${\displaystyle [3\sin \theta \cos \theta \cos \phi {\hat {\mathbf {x} }}}$
		${\displaystyle +3\sin \theta \cos \theta \sin \phi {\hat {\mathbf {y} }}}$
		${\displaystyle +(2\cos ^{2}\theta -\sin ^{2}\theta ){\hat {\mathbf {z} }}]}$	${\displaystyle r^{2}\sin \theta \ \mathrm {d} \theta \mathrm {d} \phi \mathrm {d} r}$。

注意到这积分式的x-分量与y-分量都等于零，只剩下z-分量：

${\displaystyle {\begin{aligned}\langle \mathbf {E} \rangle &={\frac {3p}{16\pi ^{2}\epsilon _{0}b^{3}}}\int _{0}^{b}\int _{0}^{2\pi }\int _{0}^{\pi }{\frac {1}{r}}(2\cos ^{2}\theta -\sin ^{2}\theta ){\hat {\mathbf {z} }}\sin \theta \ \mathrm {d} \theta \mathrm {d} \phi \mathrm {d} r\\&={\frac {3p{\hat {\mathbf {z} }}}{8\pi \epsilon _{0}b^{3}}}\int _{0}^{b}{\frac {1}{r}}\ \mathrm {d} r\int _{0}^{\pi }(2\sin \theta \cos ^{2}\theta -\sin ^{3}\theta )\ \mathrm {d} \theta \end{aligned}}}$ 。

对于径向坐标 ${\displaystyle r}$ 积分会得到

${\displaystyle \int _{0}^{b}{\frac {1}{r}}\ \mathrm {d} r=-\infty }$ ！

但对于天顶角 ${\displaystyle \theta }$ 积分则会得到

${\displaystyle \int _{0}^{\pi }(2\sin \theta \cos ^{2}\theta -\sin ^{3}\theta )\ \mathrm {d} \theta =\int _{0}^{\pi }(2\sin \theta \cos ^{2}\theta -\sin ^{3}\theta )\ \mathrm {d} \theta =0}$ ！

由此可知，从这运算无法得到 ${\displaystyle \langle \mathbf {E} \rangle }$ 的正确值。这是因为电偶极子的电势有一个奇点在它所处的位置（原点 ${\displaystyle \mathbf {O} }$ ），电场的方程式并不完全正确。必须特别小心地计算，才能得到正确答案。应用向量恒等式 ${\displaystyle \oint _{\mathbb {S} }\psi \ \mathrm {d} \mathbf {S} =\int _{\mathbb {V} }\nabla \psi \ \mathrm {d} V}$ ，则作用于这球体 ${\displaystyle \mathbb {V} }$ 的电场，其平均值为：

${\displaystyle \langle \mathbf {E} \rangle ={\frac {3}{4\pi b^{3}}}\int _{\mathbb {V} }\mathbf {E} \ \mathrm {d} ^{3}\mathbf {r} =-\ {\frac {3}{4\pi b^{3}}}\int _{\mathbb {V} }\nabla \phi \ \mathrm {d} ^{3}\mathbf {r} =-\ {\frac {3}{4\pi b^{3}}}\oint _{\mathbb {S} }\phi \ \mathrm {d} \mathbf {S} }$ ；

其中，${\displaystyle \mathbb {S} }$ 是球体 ${\displaystyle \mathbb {V} }$ 的表面。

将电势 ${\displaystyle \phi }$ 的方程式代入，注意到 ${\displaystyle \mathrm {d} \mathbf {S} ={\hat {\mathbf {r} }}\ b^{2}\sin \theta \ \mathrm {d} \theta \mathrm {d} \phi }$ ，则可得到

${\displaystyle \langle \mathbf {E} \rangle =-\ {\frac {3}{(4\pi )^{2}b\epsilon _{0}}}\oint _{\mathbb {S} }\left[\int _{\mathbb {V} '}{\frac {\rho (\mathbf {r} ')}{|b{\hat {\mathbf {r} }}-\mathbf {r} '|}}\ \mathrm {d} ^{3}\mathbf {r} '\right]{\hat {\mathbf {r} }}\ \sin \theta \ \mathrm {d} \theta \mathrm {d} \phi }$ ；

其中，${\displaystyle \rho (\mathbf {r} ')}$ 是在源位置 ${\displaystyle \mathbf {r} '}$ 的电荷密度， ${\displaystyle \mathbb {V} '}$ 是源积分体积，设定与 ${\displaystyle \mathbb {V} }$ 相同，${\displaystyle {\hat {\mathbf {r} }}}$ 是场位置的单位向量，从表面 ${\displaystyle \mathbb {S} }$ 垂直往外指出。

场位置与源位置之间距离的倒数以球谐函数 ${\displaystyle Y_{\ell m}(\theta ,\phi )}$ 作多极展开为

${\displaystyle {\frac {1}{|b{\hat {\mathbf {r} }}-\mathbf {r} '|}}=\sum _{\ell =0}^{\infty }\sum _{m=-\ell }^{\ell }{\frac {4\pi }{2\ell +1}}{\frac {r^{\prime \ell }}{b^{\ell +1}}}Y_{\ell m}^{*}(\theta ',\phi ')Y_{\ell m}(\theta ,\phi ),\qquad r'<b}$ ；

其中，${\displaystyle b{\hat {\mathbf {r} }}}$ 与 ${\displaystyle \mathbf {r} '}$ 的球坐标分别为 ${\displaystyle (b,\theta ,\phi )}$ 与 ${\displaystyle (r',\theta ',\phi ')}$ 。

单位向量 ${\displaystyle {\hat {\mathbf {r} }}}$ 以球谐函数表示为

${\displaystyle {\begin{aligned}{\hat {\mathbf {r} }}&={\hat {\mathbf {x} }}\sin \theta \cos \phi +{\hat {\mathbf {y} }}\sin \theta \sin \phi +{\hat {\mathbf {z} }}\cos \theta \\&={\hat {\mathbf {x} }}\left[-{\sqrt {\frac {2\pi }{3}}}(-Y_{1,-1}^{*}+Y_{11}^{*})\right]+{\hat {\mathbf {y} }}\left[-{\sqrt {\frac {2\pi }{3}}}(-Y_{1,-1}^{*}-Y_{11}^{*})\right]+{\hat {\mathbf {z} }}{\sqrt {\frac {4\pi }{3}}}Y_{10}^{*}\\\end{aligned}}}$ 。

应用球谐函数的正交归一性

${\displaystyle \int _{0}^{2\pi }\int _{0}^{\pi }Y_{\ell 'm'}^{*}(\theta ,\phi )Y_{\ell m}(\theta ,\phi )\sin \theta \ \mathrm {d} \theta \mathrm {d} \phi =\delta _{\ell \ell '}\delta _{mm'}}$ ，

可以得到 ${\displaystyle \langle \mathbf {E} \rangle }$ 与这球体的电偶极子 ${\displaystyle \mathbf {p} }$ 之间的关系式：

${\displaystyle \langle \mathbf {E} \rangle =-\ {\frac {1}{4\pi b^{3}\epsilon _{0}}}\int _{\mathbb {V} '}\mathbf {r} '\rho (\mathbf {r} ')\ \mathrm {d} ^{3}\mathbf {r} '=-\ {\frac {\mathbf {p} }{4\pi b^{3}\epsilon _{0}}}}$ 。

也就是说，

${\displaystyle \int _{\mathbb {V} }\mathbf {E} \ \mathrm {d} ^{3}\mathbf {r} =-\ {\frac {\mathbf {p} }{3\epsilon _{0}}}}$ 。

为了满足这性质，必需对于电偶极子 ${\displaystyle \mathbf {p} }$ 所产生的电场 ${\displaystyle \mathbf {E} }$ ，在其方程式内再添加一个项目：

${\displaystyle {\begin{aligned}\mathbf {E} &={\frac {1}{4\pi \epsilon _{0}r^{3}}}\left(3(\mathbf {p} \cdot {\hat {\mathbf {r} }}){\hat {\mathbf {r} }}-\mathbf {p} \right)-{\frac {\mathbf {p} }{3\epsilon _{0}}}\delta ^{3}(\mathbf {r} )\\&={\frac {p}{4\pi \epsilon _{0}r^{3}}}(2\cos \theta {\hat {\mathbf {r} }}+\sin \theta {\hat {\boldsymbol {\theta }}})-{\frac {\mathbf {p} }{3\epsilon _{0}}}\delta ^{3}(\mathbf {r} )\end{aligned}}}$ 。

这样，在计算 ${\displaystyle \langle \mathbf {E} \rangle }$ 时，就能够得到明确无误的答案。

磁偶极子的磁场

参见：载流回路产生的磁场

假设磁偶极矩为 ${\displaystyle \mathbf {m} }$ 的磁偶极子，其位置是在原点，则在任意位置 ${\displaystyle \mathbf {r} }$ ，磁偶极子的向量势 ${\displaystyle \mathbf {A} }$ 是

${\displaystyle \mathbf {A} (\mathbf {r} )={\frac {\mu _{0}}{4\pi r^{2}}}(\mathbf {m} \times {\hat {\mathbf {r} }})}$ ；

其中，${\displaystyle \mu _{0}}$ 是磁常数。

这磁偶极子所产生的磁场 ${\displaystyle \mathbf {B} }$ 为

${\displaystyle \mathbf {B} =\nabla \times \mathbf {A} }$ 。

由于磁偶极子的向量势有一个奇点在它所处的位置（原点 ${\displaystyle \mathbf {O} }$ ），必须特别小心地计算，才能得到正确答案。更仔细地推导，可以得到磁场为^[2]

${\displaystyle \mathbf {B} (\mathbf {r} )={\frac {\mu _{0}}{4\pi r^{3}}}\left(3(\mathbf {m} \cdot {\hat {\mathbf {r} }}){\hat {\mathbf {r} }}-\mathbf {m} \right)+{\frac {2\mu _{0}\mathbf {m} }{3}}\delta ^{3}(\mathbf {r} )}$ 。

任意磁场的多极展开式中，带头项目就是这公式右手边的第一个项目，偶极子项目。磁场没有单极子项目。在远距离，这公式近似任何类似磁偶极子的组态所产生的磁场。

偶极磁场的狄拉克δ函数项目造成了原子能级分裂，因而形成了超精细结构（hyperfine structure）^[3]。在天文学里，氢原子的超精细结构给出了21公分谱线，在电磁辐射的无线电波范围，是除了3K背景辐射以外，宇宙弥漫最广阔的电磁辐射。从复合纪元（recombination）至再电离纪元（reionization）之间的天文学研究，只能依靠观测21公分谱线无线电波。

作用于偶极极子的力矩

将一磁偶极子放在均匀磁场，或将电偶极子放在均匀电场，偶极子的两端会分别各产生一个力，两个大小相等而方向相反的力产生力矩 ${\displaystyle {\boldsymbol {\tau }}}$ ：

${\displaystyle {\boldsymbol {\tau }}=\mathbf {p} \times \mathbf {E} }$ 、

${\displaystyle {\boldsymbol {\tau }}=\mathbf {m} \times \mathbf {B} }$ 。

力矩倾向将偶极子的方向与向量场的方向排向同一方向，偶极子的位能是

${\displaystyle U=-\mathbf {p} \cdot \mathbf {E} }$ 、

${\displaystyle U=-\mathbf {m} \cdot \mathbf {B} }$ 。

在计算时，我们常假设偶极子两端之间的距离是无穷小，即点偶极子。

偶极辐射

一个震荡电偶极子的电场的即时演化。

参见：偶极子天线

在静电学和静磁学之外，很重要的物理领域是含时偶极子。

当一个电偶极子在做谐振荡时，其电偶极矩可以表示为 ${\displaystyle \mathbf {p} =\mathbf {p'(\mathbf {r} )} e^{-i\omega t}}$ ；其中，${\displaystyle \omega }$ 是角频率。在真空里，它产生的电场和磁场分别为

${\displaystyle \mathbf {E} ={\frac {1}{4\pi \varepsilon _{0}}}\left\{{\frac {\omega ^{2}}{c^{2}r}}{\hat {\mathbf {r} }}\times \mathbf {p} \times {\hat {\mathbf {r} }}+\left({\frac {1}{r^{3}}}-{\frac {i\omega }{cr^{2}}}\right)\left[3{\hat {\mathbf {r} }}({\hat {\mathbf {r} }}\cdot \mathbf {p} )-\mathbf {p} \right]\right\}e^{i\omega r/c}}$ 、

${\displaystyle \mathbf {B} ={\frac {\omega ^{2}}{4\pi \varepsilon _{0}c^{3}}}{\hat {\mathbf {r} }}\times \mathbf {p} \left(1-{\frac {c}{i\omega r}}\right){\frac {e^{i\omega r/c}}{r}}}$ 。

在离开偶极子很远的位置（${\displaystyle r\omega /c\gg 1}$），向量场的形式近似一个辐射的球面波：

${\displaystyle \mathbf {B} ={\frac {\omega ^{2}}{4\pi \varepsilon _{0}c^{3}}}({\hat {\mathbf {r} }}\times \mathbf {p} ){\frac {e^{i\omega r/c}}{r}}}$ 、

${\displaystyle \mathbf {E} =c\mathbf {B} \times {\hat {\mathbf {r} }}}$ 。

经过时间平均，产生的总辐射功率 ${\displaystyle P}$ 为

${\displaystyle P={\frac {\omega ^{4}}{12\pi \varepsilon _{0}c^{3}}}|\mathbf {p} |^{2}}$ 。

功率的分布并不具有均向性，而是集中于垂直于电偶极矩的方向。

量子力学的电偶极子算符

试想一群粒子，数量为 ${\displaystyle N}$ ，电荷量和位置分别为 ${\displaystyle q_{i}}$ 和 ${\displaystyle \mathbf {r} _{i}}$ ，${\displaystyle i=1,\,2,\,\dots ,\,N}$ 。例如，这个群集可能是一个分子，由电荷量为 ${\displaystyle -e}$ 的电子，和电荷量为 ${\displaystyle eZ_{j}}$ 的原子核所构成；其中，${\displaystyle Z_{j}}$ 是第 ${\displaystyle j}$ 个原子核的原子序。这个群集的电偶极子的量子算符 ${\displaystyle {\mathfrak {p}}}$ 是

${\displaystyle {\mathfrak {p}}=\sum _{i=1}^{N}\,q_{i}\,\mathbf {r} _{i}}$ 。

参阅

• 电介质
• 永电体
• 印度洋电偶极子（Indian Ocean Dipole）
• 自旋磁矩（spin magnetic moment）
• 轴多极矩（Axial multipole moments）
• 圆柱多极矩（Cylindrical multipole moments）
• 球多极矩
• 拉普拉斯展开式（位势论）（Laplace expansion (potential)）
• 勒让德多项式