直纹曲面

在几何学中，如果一个曲面上的任意一点上均有至少一条直线经过，则称该曲面为直纹曲面（英语：Ruled Surface）。另一种常见的说法是，如果一个曲面可以由一条直线通过连续运动构成，则可称其为直纹曲面。以三维欧几里德空间为例，最常见的直纹曲面是平面、柱面、锥面和马鞍面。著名的莫比乌斯环也是直纹曲面。

单叶双曲面的任意一点上均有两条直线经过。这类曲面被称为双重直纹曲面。

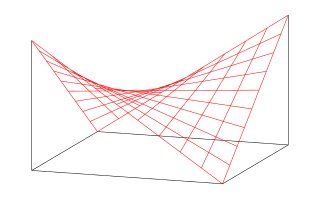
假如一个曲面上的任意一点均有两条不同的直线经过，那么称该曲面为双重直纹曲面（英语：Doubly Ruled Surface）。双曲抛物面和单叶双曲面（右图）即为双重直纹曲面的典型例子。对于曲面上每个点均有三条或更多的直线经过的曲面，可称为三重和多重直纹曲面。不过在三维欧几里得空间中，除了平面以外，不存在这样的直纹曲面。

微分几何中的直纹曲面

参数表示

一个直纹螺旋曲面

如果将直纹曲面看作一条连续运动的直线所经过的点, 那么可将曲面表达为一个如下述形式的参数方程:

${\displaystyle S(t,u)=p(t)+ur(t)\ }$

其中${\displaystyle S(t,u)}$为面上的任意点，${\displaystyle p(t)}$为沿着面上一曲线移动之点，${\displaystyle r(t)}$为随${\displaystyle t}$变动之单位向量。举例来说，如果我们用下列式子

${\displaystyle {\begin{aligned}p(t)&=(\cos(2t),\sin(2t),0)\\r(t)&=(\cos t\cos 2t,\cos t\sin 2t,\sin t)\end{aligned}}}$

则可得莫比乌斯带。另一种参数表示法为：

${\displaystyle S(t,u)=(1-u)p(t)+uq(t)}$

其中${\displaystyle p}$及${\displaystyle q}$为两条处于面上之不相交曲线。当 ${\displaystyle p(t)}$ 及${\displaystyle q(t)}$以定速沿着二歪斜线移动时，${\displaystyle S}$为一双曲抛物面或是单叶双曲面。

可展曲面

主条目：可展曲面

可展曲面即为高斯曲率处处为零的曲面。另一种常见的表述方法是，一个可展曲面的每一部分都可以不经压缩或者拉伸而展开成为一个平面。三维欧氏空间中的完备可展曲面一定是直纹曲面。然而，相同前提下的直纹曲面不一定是可展曲面，单叶双曲面便是一例。四维欧氏空间存在不是直纹曲面的可展曲面。

代数几何中的直纹曲面

方程z=xy表示一个双重直纹曲面:双曲抛物面

建筑领域中的应用

日本兵库县神户市的地标建筑——神户港塔

大多数热力发电厂的冷却塔结构都是单叶双曲面形状。由于单叶双曲面是一种双重直纹曲面（Ruled surface），它可以用直的钢梁建造。这样既可减少风的阻力，又可以用最少的材料来维持结构的完整。

参见

引用

• Barth, Wolf P.; Hulek, Klaus; Peters, Chris A.M.; Van de Ven, Antonius, Compact Complex Surfaces, Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge. 4, Springer-Verlag, Berlin, 2004, ISBN 978-3-540-00832-3, MR 2030225
• Beauville, Arnaud, Complex algebraic surfaces, London Mathematical Society Student Texts 34 2nd, Cambridge University Press, 1996, ISBN 978-0-521-49510-3, MR 1406314
• Sharp, John, D-Forms, Tarquin, 2008. Models exploring rules surfaces Review: Jrnl of Mathematics and the Arts 3 (2009), 229-230 ISBN 978-1-899618-87-3
• Edge, W. L., The Theory of Ruled Surfaces, Cambridge, University Press, 1931. Review: Bull. Amer. Math. Soc. 37 (1931), 791-793, doi:10.1090/S0002-9904-1931-05248-4
• Hilbert, David; Cohn-Vossen, Stephan, Geometry and the Imagination 2nd, New York: Chelsea, 1952, ISBN 978-0-8284-1087-8.
• Iskovskikh, V.A., Ruled surface, Hazewinkel, Michiel (编), 数学百科全书, Springer, 2001, ISBN 978-1-55608-010-4

外部链接

• 埃里克·韦斯坦因. Ruled Surface. MathWorld.
• Ruled surface pictures from the University of Arizona （页面存档备份，存于互联网档案馆）
• Examples of developable surfaces on the Rhino3DE website （页面存档备份，存于互联网档案馆）