동역학계

동적계(動的系, dynamical system) 또는 동역학계(動力學系)는 수학의 한 분야로서, 매개변수에 따른 변화 과정으로 정의된다. 현대적 의미에서의 동적계 연구는 미국의 수학자 조지 데이비드 버코프에서 시작된다. 오늘날 동적계 연구는 주로 수학 분야에서 다뤄지고 있으나 실제로 수론, 추계학, 동역학, 생물학등 광범위하게 적용되고 있다.

로렌즈 끌개(Lorenz attractor)

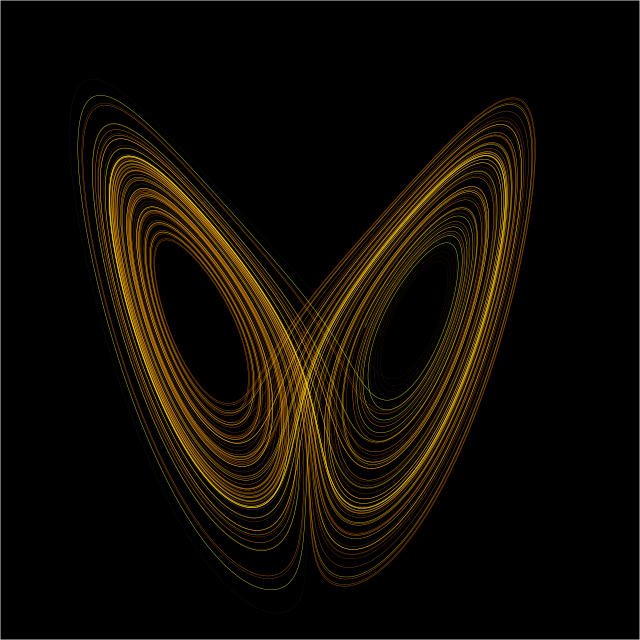
일반적으로 시공간 변화에 따라 이산과 연속체로 구별된다. 즉, 이산 동적계(Discrete Dynamical System)와 연속 동적계(Continuum Dynamical System)로 나뉘어 연구되고 있다. 일반적으로 미분방정식에서 연속 동적계를 다루고 있으며, 위상수학에서 이산, 연속 동적계를 모두 다루고 있다. 특히, 이 두가지를 혼합하여 연구하는 경우 연속-이산 동적계 또는 혼합 동적계(Hybrid Dynamical System)로 표현되고 있다.

정의

• 동적계는 일반적으로 공집합이 아닌 시공간 또는 상태공간 집합 ${\displaystyle X}$에서, ${\displaystyle T=\mathbb {N} _{0},\mathbb {Z} ,\mathbb {R} _{0}^{+}}$ 또는 ${\displaystyle \mathbb {R} }$로 부터의 튜플 ${\displaystyle (T,X,f)}$이고, ${\displaystyle X}$에서 ${\displaystyle T}$의 연산 ${\displaystyle f\colon \,T\times X\to X}$에 대해 모든 상태 ${\displaystyle x\in X}$ 그리고 모든 시공간 ${\displaystyle t_{1},t_{2}\in T}$에서 다음이 성립된다:

1. ${\displaystyle f(0,x)=x}$   (동일성)
2. ${\displaystyle f(t_{2},f(t_{1},x))=f(t_{2}+t_{1},x)}$   (반집합성)

• ${\displaystyle T=\mathbb {N} _{0}}$ 또는 ${\displaystyle T=\mathbb {Z} }$ 일 때, ${\displaystyle (T,X,f)}$는 시간이산적 또는 이산적, 그리고 ${\displaystyle T=\mathbb {R} _{0}^{+}}$ 또는 ${\displaystyle T=\mathbb {R} }$ 이면, ${\displaystyle (T,X,f)}$을 시간연속적 또는 연속적이라 한다. 그밖에 ${\displaystyle T=\mathbb {Z} }$ 또는 ${\displaystyle T=\mathbb {R} }$이면, ${\displaystyle (T,X,f)}$는 실시간적 또는 가역적이라 한다.

• 임의의 ${\displaystyle x\in X}$에 대해 자취 ${\displaystyle \beta _{x}\colon \,T\to X,\,t\mapsto \beta _{x}(t):=f(t,x)}$는 ${\displaystyle x=\beta _{x}(0)}$의 움직임, 그리고 집합 ${\displaystyle O(x):=\{\beta _{x}(t)\mid t\in T\}}$는 ${\displaystyle x}$의 궤도라 한다. 그리고 ${\displaystyle (T,X,f)}$이 가역적일 때, ${\displaystyle x}$의 양의 반궤도는 ${\displaystyle O^{+}(x):=\{\beta _{x}(t)\mid t\in T\cap \mathbb {R} _{0}^{+}\}}$이고, ${\displaystyle O^{-}(x):=\{\beta _{x}(t)\mid -t\in T\cap \mathbb {R} _{0}^{+}\}}$는 음의 반궤도가 된다.

• 상태 공간 ${\displaystyle X}$ 이 공집합이 아닌 거리 공간이고, 각 시점 ${\displaystyle t\in T}$이 ${\displaystyle \varphi _{t}\colon \,X\to X,\,x\mapsto \varphi _{t}(x):=f(t,x)}$을 갖는 변환 ${\displaystyle \varphi _{t}\colon \,X\to X,\,x\mapsto \varphi _{t}(x):=f(t,x)}$이 연속일 때, 이산 동적계 ${\displaystyle (T,X,f)}$는 연속이다. 상태 공간 ${\displaystyle X}$ 이 거리 공간이고, 각 시점을 갖는 변환 및 각 상태의 움직임이 연속일 때, 연속 동적계 ${\displaystyle (T,X,f)}$는 연속이다. 이산 동적계와 연속 동적계의 연속조건을 모두 만족할 때, 혼합 동적계라한다.

응용

물리학

해밀토니안 계, 미분가능한 유체에서 적용된다.

생물학

질병의 전염성을 다루는 SIR-Model(Susceptible-Infected-Recovered-Model)에서 적용된다.

같이 보기

• 인지 모델
• 진동
• 샤르코우스키 정리
• 시스템 다이내믹스
• 체계 이론

참고 문헌

• G.D. Birkhoff: Dynamical Systems. Rev. Ed.. AMS, Providence, RI, 1966.
• J. Guckenheimer, Ph. Holmes: Nonlinear Oscillations, Dynamical Systems and Bifurcations of Vector Fields. Corr. 3rd printing. Springer, New York 1990. ISBN 3-540-90819-6
• Diederich Hinrichsen, Anthony J. Pritchard: Mathematical Systems Theory I - Modelling, State Space Analysis, Stability and Robustness. Springer, 2005.
• Wolfgang Metzler: Nichtlineare Dynamik und Chaos, B.G. Teubner, Stuttgart–Leipzig 1998. ISBN 3-519-02391-1
• R.S. Sreenivas, B.H. Krogh: ON Condition/Event Systems with Discrete State Realization. Discrete Event Dynamic Systems: Theory and Application 1. 1991. p. 209ff.
• J. de Vries: Elements of Topological Dynamics. Springer, 1993.