Flattening

"Ellipticity" redirects here. For ellipticity in differential calculus, see elliptic operator. For other uses, see Flattening (disambiguation).

Flattening is a measure of the compression of a circle or sphere along a diameter to form an ellipse or an ellipsoid of revolution (spheroid) respectively. Other terms used are ellipticity, or oblateness. The usual notation for flattening is ${\displaystyle f}$ and its definition in terms of the semi-axes ${\displaystyle a}$ and ${\displaystyle b}$ of the resulting ellipse or ellipsoid is

${\displaystyle f={\frac {a-b}{a}}.}$

A circle of radius a compressed to an ellipse.

A sphere of radius a compressed to an oblate ellipsoid of revolution.

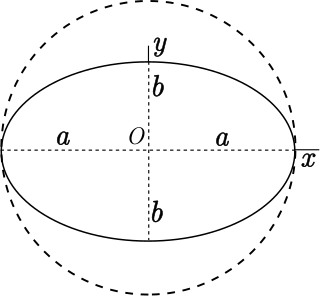
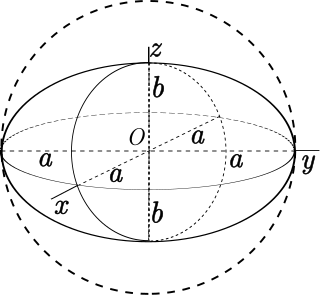
The compression factor is ${\displaystyle b/a}$ in each case; for the ellipse, this is also its aspect ratio.

Definitions

There are three variants: the flattening ${\displaystyle f,}$^[1] sometimes called the first flattening,^[2] as well as two other "flattenings" ${\displaystyle f'}$ and ${\displaystyle n,}$ each sometimes called the second flattening,^[3] sometimes only given a symbol,^[4] or sometimes called the second flattening and third flattening, respectively.^[5]

In the following, ${\displaystyle a}$ is the larger dimension (e.g. semimajor axis), whereas ${\displaystyle b}$ is the smaller (semiminor axis). All flattenings are zero for a circle (a = b).

(First) flattening	${\displaystyle f}$	${\displaystyle {\frac {a-b}{a}}}$	Fundamental. Geodetic reference ellipsoids are specified by giving ${\displaystyle {\frac {1}{f}}\,\!}$
Second flattening	${\displaystyle f'}$	${\displaystyle {\frac {a-b}{b}}}$	Rarely used.
Third flattening	${\displaystyle n}$	${\displaystyle {\frac {a-b}{a+b}}}$	Used in geodetic calculations as a small expansion parameter.[6]

Identities

The flattenings can be related to each-other:

${\displaystyle {\begin{aligned}f={\frac {2n}{1+n}},\\[5mu]n={\frac {f}{2-f}}.\end{aligned}}}$

The flattenings are related to other parameters of the ellipse. For example,

${\displaystyle {\begin{aligned}{\frac {b}{a}}&=1-f={\frac {1-n}{1+n}},\\[5mu]e^{2}&=2f-f^{2}={\frac {4n}{(1+n)^{2}}},\\[5mu]f&=1-{\sqrt {1-e^{2}}},\end{aligned}}}$

where ${\displaystyle e}$ is the eccentricity.

See also

• Earth flattening
• Eccentricity (mathematics) § Ellipses
• Equatorial bulge
• Ovality
• Planetary flattening
• Sphericity
• Roundness (object)