Loading AI tools
In the history of physics, "On the quantum-theoretical reinterpretation of kinematical and mechanical relationships" (German: Über quantentheoretische Umdeutung kinematischer und mechanischer Beziehungen), also known as the Umdeutung (reinterpretation) paper,[1][2] was a breakthrough article in quantum mechanics written by Werner Heisenberg, which appeared in Zeitschrift für Physik in September 1925.
In the article, Heisenberg tried to explain the energy levels of a one-dimensional anharmonic oscillator, avoiding the concrete but unobservable representations of electron orbits by using observable parameters such as transition probabilities for quantum jumps, which necessitated using two indexes corresponding to the initial and final states.[3]
Mathematically, Heisenberg showed the need of non-commutative operators. This insight would later become the basis for Heisenberg's uncertainty principle.
This article was followed by the paper by Pascual Jordan and Max Born of the same year,[4] and by the 'three-man paper' (German: drei Männer Arbeit) by Born, Heisenberg and Jordan in 1926.[5][1][6] These articles laid the groundwork for matrix mechanics that would come to substitute old quantum theory, leading to the modern quantum mechanics. Heisenberg received the Nobel Prize in Physics in 1932 for his work on developing quantum mechanics.[7]
Heisenberg was 23 years old when he worked on the article while recovering from hay fever on the island of Heligoland, corresponding with Wolfgang Pauli on the subject. When asked for his opinion of the manuscript, Pauli responded favorably, but Heisenberg said that he was still "very uncertain about it". In July 1925, he sent the manuscript to Max Born to review and decide whether to submit it for publication.[8]
When Born read the article, he recognized the formulation as one which could be transcribed and extended to the systematic language of matrices. Born, with the help of his assistant and former student Pascual Jordan, began immediately to make the transcription and extension, and they submitted their results for publication; their manuscript was received for publication just 60 days after Heisenberg’s article.[4] A follow-on article by all three authors extending the theory to multiple dimensions was submitted for publication before the end of the year.[5]
Heisenberg determined to base his quantum mechanics "exclusively upon relationships between quantities that in principle are observable".[9] He observed that one could not then use any statements about such things as "the position and period of revolution of the electron".[10] Rather, to make true progress in understanding the radiation of the simplest case, the radiation of excited hydrogen atoms, one had measurements only of the frequencies and the intensities of the hydrogen bright-line spectrum to work with.
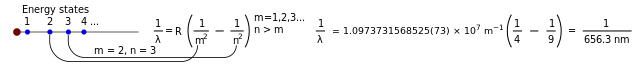
In classical physics, the intensity of each frequency of light produced in a radiating system is equal to the square of the amplitude of the radiation at that frequency, so attention next fell on amplitudes. The classical equations that Heisenberg hoped to use to form quantum theoretical equations would first yield the amplitudes, and in classical physics one could compute the intensities simply by squaring the amplitudes. But Heisenberg saw that "the simplest and most natural assumption would be"[11] to follow the lead provided by recent work in computing light dispersion done by Hans Kramers.[12] The work he had done assisting Kramers in the previous year[13] now gave him an important clue about how to model what happened to excited hydrogen gas when it radiated light and what happened when incoming radiation of one frequency excited atoms in a dispersive medium and then the energy delivered by the incoming light was re-radiated – sometimes at the original frequency but often at two lower frequencies the sum of which equalled the original frequency. According to their model, an electron that had been driven to a higher energy state by accepting the energy of an incoming photon might return in one step to its equilibrium position, re-radiating a photon of the same frequency, or it might return in more than one step, radiating one photon for each step in its return to its equilibrium state. Because of the way factors cancel out in deriving the new equation based on these considerations, the result turns out to be relatively simple.
Also included in the manuscript was the Heisenberg commutator, his law of multiplication needed to describe certain properties of atoms, whereby the product of two physical quantities did not commute. Therefore, PQ would differ from QP where, for example, P was an electron's momentum, and Q its position. Paul Dirac, who had received a proof copy in August 1925, realized that the commutative law had not been fully developed, and he produced an algebraic formulation to express the same results in more logical form.[14]
By means of an intense series of mathematical analogies that some physicists have termed "magical", Heisenberg wrote out an equation that is the quantum mechanical analog for the classical computation of intensities. The equation below appears in the paper.[15][16] Its general form is as follows:
This general format indicates that some term C is to be computed by summing up all of the products of some group of terms A by some related group of terms B. There will potentially be an infinite series of A terms and their matching B terms. Each of these multiplications has as its factors two measurements that pertain to sequential downward transitions between energy states of an electron. This type of rule differentiates matrix mechanics from the kind of physics familiar in everyday life because the important values are where (in what energy state or "orbital") the electron begins and in what energy state it ends, not what the electron is doing while in one or another state.
If A and B both refer to lists of frequencies, for instance, the calculation proceeds as follows:
Multiply the frequency for a change of energy from state n to state n − a by the frequency for a change of energy from state n − a to state n − b, and to that add the product found by multiplying the frequency for a change of energy from state n − a to state n − b by the frequency for a change of energy from state n − b to state n − c, and so forth. Symbolically, that is:
(According to the convention used, n − a represents a higher energy state than n, so a transition from n to n − a would indicate that an electron has accepted energy from an incoming photon and has risen to a higher orbital, while a transition from n − a to n would represent an electron falling to a lower orbital and emitting a photon.)
It would be easy to perform each individual step of this process for some measured quantity. For instance, the boxed formula at the head of this article gives each needed wavelength in sequence. The values calculated could very easily be filled into a grid as described below. However, since the series is infinite, nobody could do the entire set of calculations.
Heisenberg originally devised this equation to enable himself to multiply two measurements of the same kind (amplitudes), so it happened not to matter in which order they were multiplied. Heisenberg noticed, however that if he tried to use the same schema to multiply two variables, such as momentum, p, and displacement, q, then "a significant difficulty arises".[17] It turns out that multiplying a matrix of p by a matrix of q gives a different result from multiplying a matrix of q by a matrix of p. It only made a tiny bit of difference, but that difference could never be reduced below a certain limit, and that limit involved the Planck constant, h. More on that later. Below is a very short sample of what the calculations would be, placed into grids that are called matrices. Heisenberg's teacher saw almost immediately that his work should be expressed in a matrix format because mathematicians already were familiar with how to do computations involving matrices in an efficient way. (Since Heisenberg was interested in photon radiation, the illustrations will be given in terms of electrons going from a higher energy level to a lower level, e.g., n ← n − 1, instead of going from a lower level to a higher level, e.g., n → n − 1.) (equation for the conjugate variables momentum and position)
Matrix of p
| Electron states | n − a | n − b | n − c | ... |
|---|---|---|---|---|
| n | p(n︎ ← n − a) | p(n︎ ← n − b) | p(n︎ ← n − c) | ... |
| n − a | p(n − a︎ ← n − a) | p(n − a︎ ← n − b) | p(n − a︎ ← n − c) | ... |
| n − b | p(n − b︎ ← n − a) | p(n − b︎ ← n − b) | p(n − b︎ ← n − c) | ... |
| transition... | ... | ... | ... | ... |
Matrix of q
| Electron states | n − b | n − c | n − d | ... |
|---|---|---|---|---|
| n − a | q(n − a︎ ← n − b) | q(n − a︎ ← n − c) | q(n − a︎ ← n − d) | ... |
| n − b | q(n − b︎ ← n − b) | q(n − b︎ ← n − c) | q(n − b︎ ← n − d) | ... |
| n − c | q(n − c︎ ← n − b) | q(n − c︎ ← n − c) | q(n − c︎ ← n − d) | ... |
| transition ... | ... | ... | ... | ... |
The matrix for the product of the above two matrices as specified by the relevant equation in the Umdeutung paper is
| Electron states | n − b | n − c | n − d | ... |
| n | A | ... | ... | ... |
| n − a | ... | B | ... | ... |
| n − b | ... | ... | C | ... |
where
A = p(n︎ ← n − a)q(n − a︎ ← n − b) + p(n︎ ← n − b)q(n − b︎ ← n − b) + p(n︎ ← n − c)q(n − c︎ ← n − b) + ...
B = p(n − a︎ ← n − a)q(n − a︎ ← n − c) + p(n − a︎ ← n − b)q(n − b︎ ← n − c) + p(n − a︎ ← n − c)q(n − c︎ ← n − c) + ...
C = p(n − b︎ ← n − a)q(n − a︎ ← n − d)+p(n − b︎ ← n − b)q(n − b︎ ← n − d) + p(n − b︎ ← n − c)q(n − d︎ ← n − d) + ...
and so forth.
If the matrices were reversed, the following values would result
A = q(n︎ ← n − a)p(n − a︎ ← n − b) + q(n︎ ← n − b)p(n − b︎ ← n − b) + q(n︎ ← n − c)p(n − c︎ ← n − b) + ...
B = q(n − a︎ ← n − a)p(n − a︎ ← n − c) + q(n − a︎ ← n − b)p(n − b︎ ← n − c) + q(n − a︎ ← n − c)p(n − c︎ ← n − c) + ...
C = q(n − b︎ ← n − a)p(n − a︎ ← n − d) + q(n − b︎ ← n − b)p(n − b︎ ← n − d) + q(n − b︎ ← n − c)p(n − d︎ ← n − d) + ...
and so forth.
Werner Heisenberg used the idea that since classical physics is correct when it applies to phenomena in the world of things larger than atoms and molecules, it must stand as a special case of a more inclusive quantum theoretical model. So he hoped that he could modify quantum physics in such a way that when the parameters were on the scale of everyday objects it would look just like classical physics, but when the parameters were pulled down to the atomic scale the discontinuities seen in things like the widely spaced frequencies of the visible hydrogen bright line spectrum would come back into sight.
The one thing that people at that time most wanted to understand about hydrogen radiation was how to predict or account for the intensities of the lines in its spectrum. Although Heisenberg did not know it at the time, the general format he worked out to express his new way of working with quantum theoretical calculations can serve as a recipe for two matrices and how to multiply them.[18]
The Umdeutung paper does not mention matrices. Heisenberg's great advance was the "scheme which was capable in principle of determining uniquely the relevant physical qualities (transition frequencies and amplitudes)"[19] of hydrogen radiation.
After Heisenberg wrote the Umdeutung paper, he turned it over to one of his senior colleagues for any needed corrections and went on vacation. Max Born puzzled over the equations and the non-commuting equations that Heisenberg had found troublesome and disturbing. After several days he realized that these equations amounted to directions for writing out matrices.[20]
By consideration of ... examples. .. [Heisenberg] found this rule ... This was in the summer of 1925. Heisenberg ... took leave of absence ... and handed over his paper to me for publication ... Heisenberg's rule of multiplication left me no peace, and after a week of intensive thought and trial, I suddenly remembered an algebraic theory....Such quadratic arrays are quite familiar to mathematicians and are called matrices, in association with a definite rule of multiplication. I applied this rule to Heisenberg's quantum condition and found that it agreed for the diagonal elements. It was easy to guess what the remaining elements must be, namely, null; and immediately there stood before me the strange formula
The symbol Q is the matrix for displacement, P is the matrix for momentum, i stands for the square root of negative one, and h is the Planck constant.[21] Born and a few colleagues took up the task of working everything out in matrix form before Heisenberg returned from his time off, and within a few months the new quantum mechanics in matrix form formed the basis for another paper. This relation is now known as Heisenberg's uncertainty principle.
When quantities such as position and momentum are mentioned in the context of Heisenberg's matrix mechanics, a statement such as pq ≠ qp does not refer to a single value of p and a single value q but to a matrix (grid of values arranged in a defined way) of values of position and a matrix of values of momentum. So multiplying p times q or q times p is really talking about the matrix multiplication of the two matrices. When two matrices are multiplied, the answer is a third matrix.
Paul Dirac decided that the essence of Heisenberg's work lay in the very feature that Heisenberg had originally found problematical – the fact of non-commutativity such as that between multiplication of a momentum matrix by a displacement matrix and multiplication of a displacement matrix by a momentum matrix. That insight led Dirac in new and productive directions.[22]
Wikiwand in your browser!
Seamless Wikipedia browsing. On steroids.
Every time you click a link to Wikipedia, Wiktionary or Wikiquote in your browser's search results, it will show the modern Wikiwand interface.
Wikiwand extension is a five stars, simple, with minimum permission required to keep your browsing private, safe and transparent.
