Differentiation rules
Rules for computing derivatives of functions From Wikipedia, the free encyclopedia
This article is a summary of differentiation rules, that is, rules for computing the derivative of a function in calculus.
Elementary rules of differentiation
Summarize
Perspective
Unless otherwise stated, all functions are functions of real numbers () that return real values, although, more generally, the formulas below apply wherever they are well defined,[1][2] including the case of complex numbers ().[3]
Constant term rule
For any value of , where , if is the constant function given by , then .[4]
Proof
Let and . By the definition of the derivative:
This computation shows that the derivative of any constant function is 0.
Intuitive (geometric) explanation
The derivative of the function at a point is the slope of the line tangent to the curve at the point. The slope of the constant function is 0, because the tangent line to the constant function is horizontal and its angle is 0.
In other words, the value of the constant function, , will not change as the value of increases or decreases.
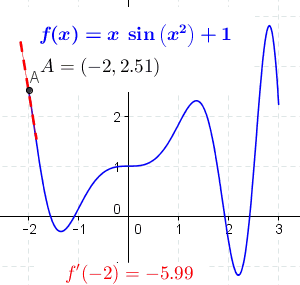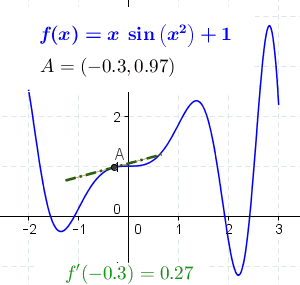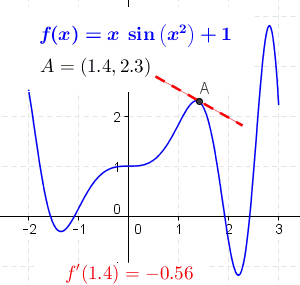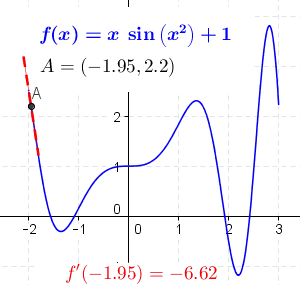
Differentiation is linear
For any functions and and any real numbers and , the derivative of the function with respect to is .
In Leibniz's notation, this formula is written as:
Special cases include:
- The constant factor rule:
- The sum rule:
- The difference rule:
Product rule
For the functions and , the derivative of the function with respect to is:
In Leibniz's notation, this formula is written:
Chain rule
The derivative of the function is:
In Leibniz's notation, this formula is written as: often abridged to:
Focusing on the notion of maps, and the differential being a map , this formula is written in a more concise way as:
Inverse function rule
If the function has an inverse function , meaning that and , then:
In Leibniz notation, this formula is written as:
Power laws, polynomials, quotients, and reciprocals
Summarize
Perspective
Polynomial or elementary power rule
If , for any real number , then:
When , this formula becomes the special case that, if , then .
Combining the power rule with the sum and constant multiple rules permits the computation of the derivative of any polynomial.
Reciprocal rule
The derivative of for any (nonvanishing) function is: wherever is nonzero.
In Leibniz's notation, this formula is written:
The reciprocal rule can be derived either from the quotient rule or from the combination of power rule and chain rule.
Quotient rule
If and are functions, then: wherever is nonzero.
This can be derived from the product rule and the reciprocal rule.
Generalized power rule
The elementary power rule generalizes considerably. The most general power rule is the functional power rule: for any functions and , wherever both sides are well defined.
Special cases:
- If , then when is any nonzero real number and is positive.
- The reciprocal rule may be derived as the special case where .
Derivatives of exponential and logarithmic functions
Summarize
Perspective
The equation above is true for all , but the derivative for yields a complex number.
The equation above is also true for all but yields a complex number if .
where is the Lambert W function.
Logarithmic derivatives
The logarithmic derivative is another way of stating the rule for differentiating the logarithm of a function (using the chain rule): wherever is positive.
Logarithmic differentiation is a technique which uses logarithms and its differentiation rules to simplify certain expressions before actually applying the derivative.[citation needed]
Logarithms can be used to remove exponents, convert products into sums, and convert division into subtraction—each of which may lead to a simplified expression for taking derivatives.
Derivatives of trigonometric functions
The derivatives in the table above are for when the range of the inverse secant is and when the range of the inverse cosecant is .
It is common to additionally define an inverse tangent function with two arguments, . Its value lies in the range and reflects the quadrant of the point . For the first and fourth quadrant (i.e., ), one has . Its partial derivatives are:
Derivatives of hyperbolic functions
Derivatives of special functions
Gamma function
with being the digamma function, expressed by the parenthesized expression to the right of in the line above.
Riemann zeta function
Derivatives of integrals
Summarize
Perspective
Suppose that it is required to differentiate with respect to the function:
where the functions and are both continuous in both and in some region of the plane, including , where , and the functions and are both continuous and both have continuous derivatives for . Then, for :
This formula is the general form of the Leibniz integral rule and can be derived using the fundamental theorem of calculus.
Derivatives to nth order
Some rules exist for computing the th derivative of functions, where is a positive integer, including:
Faà di Bruno's formula
If and are -times differentiable, then: where and the set consists of all non-negative integer solutions of the Diophantine equation .
General Leibniz rule
If and are -times differentiable, then:
See also
- Differentiable function – Mathematical function whose derivative exists
- Differential of a function – Notion in calculus
- Differentiation of integrals – Problem in mathematics
- Differentiation under the integral sign – Differentiation under the integral sign formula
- Hyperbolic functions – Collective name of 6 mathematical functions
- Inverse hyperbolic functions – Mathematical functions
- Inverse trigonometric functions – Inverse functions of sin, cos, tan, etc.
- Lists of integrals
- List of mathematical functions
- Matrix calculus – Specialized notation for multivariable calculus
- Trigonometric functions – Functions of an angle
- Vector calculus identities – Mathematical identities
References
Sources and further reading
External links
Wikiwand - on
Seamless Wikipedia browsing. On steroids.




























![{\displaystyle [{\text{D}}(f\circ g)]_{x}=[{\text{D}}f]_{g(x)}\cdot [{\text{D}}g]_{x}.}](http://wikimedia.org/api/rest_v1/media/math/render/svg/11ed19ba5c8323e932203fae323a6e684f7b4bf1)






























![{\displaystyle {\frac {d}{dx}}\left(f_{1}(x)^{f_{2}(x)^{\left(...\right)^{f_{n}(x)}}}\right)=\left[\sum \limits _{k=1}^{n}{\frac {\partial }{\partial x_{k}}}\left(f_{1}(x_{1})^{f_{2}(x_{2})^{\left(...\right)^{f_{n}(x_{n})}}}\right)\right]{\biggr \vert }_{x_{1}=x_{2}=...=x_{n}=x},\qquad {\text{ if }}f_{i<n}(x)>0{\text{ and }}{\frac {df_{i}}{dx}}{\text{ exists.}}}](http://wikimedia.org/api/rest_v1/media/math/render/svg/dd55c061b46a1308dd0527fa0f12f581cd9f959c)













![{\textstyle [0,\pi ]}](http://wikimedia.org/api/rest_v1/media/math/render/svg/856c41db5d37ffc97c5123272126eb03b2f742bf)
![{\textstyle \left[-{\frac {\pi }{2}},{\frac {\pi }{2}}\right]}](http://wikimedia.org/api/rest_v1/media/math/render/svg/b72e6de4a8a1535df6b671712877fe86434f7c8d)

![{\textstyle [-\pi ,\pi ]}](http://wikimedia.org/api/rest_v1/media/math/render/svg/967e7f43dd96f2665fa178602b395c8a990a1dac)


































![{\displaystyle {\frac {d^{n}}{dx^{n}}}[f(g(x))]=n!\sum _{\{k_{m}\}}f^{(r)}(g(x))\prod _{m=1}^{n}{\frac {1}{k_{m}!}}\left(g^{(m)}(x)\right)^{k_{m}},}](http://wikimedia.org/api/rest_v1/media/math/render/svg/3bc039afd9d17b704610c62dd85abd854181e29f)



![{\displaystyle {\frac {d^{n}}{dx^{n}}}[f(x)g(x)]=\sum _{k=0}^{n}{\binom {n}{k}}{\frac {d^{n-k}}{dx^{n-k}}}f(x){\frac {d^{k}}{dx^{k}}}g(x).}](http://wikimedia.org/api/rest_v1/media/math/render/svg/7266c7523dca0d61e13c156ee57b11c3b9db46f3)