Υπερβολικές συναρτήσεις

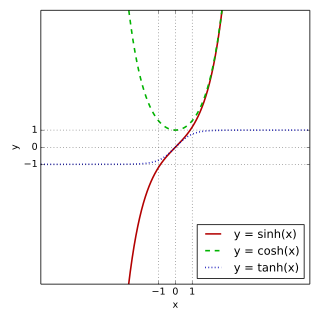
Στα μαθηματικά, οι υπερβολικές συναρτήσεις είναι ανάλογες των συμβατικών τριγωνομετρικών ή κυκλικών συναρτήσεων. Οι βασικές υπερβολικές συναρτήσεις είναι το υπερβολικό ημίτονο (συμβολίζεται sinh) και το υπερβολικό συνημίτονο (cosh), από τις οποίες προκύπτουν η υπερβολική εφαπτομένη (tanh) και οι υπόλοιπες υπερβολικές, κατ' αναλογία των παράγωγων τριγωνομετρικών συναρτήσεων. Οι συναρτήσεις αυτές ονομάστηκαν έτσι επειδή η γεωμετρική σχέση τους με μία υπερβολή είναι σχεδόν ίδια με την σχέση των τριγωνομετρικών συναρτήσεων με την περιφέρεια.^[1]

Γραφικές παραστάσεις των συναρτήσεων sinh, cosh και tanh

Γραφικές παραστάσεις των συναρτήσεων csch, sech και coth

Αλγεβρικές εκφράσεις

• Υπερβολικό ημίτονο

${\displaystyle \sinh x={\frac {e^{x}-e^{-x}}{2}}=-i\sin ix\!}$

• Υπερβολικό συνημίτονο

${\displaystyle \cosh x={\frac {e^{x}+e^{-x}}{2}}=\cos ix\!}$

• Υπερβολική εφαπτομένη

${\displaystyle \tanh x={\frac {\sinh x}{\cosh x}}={\frac {\frac {e^{x}-e^{-x}}{2}}{\frac {e^{x}+e^{-x}}{2}}}={\frac {e^{x}-e^{-x}}{e^{x}+e^{-x}}}={\frac {e^{2x}-1}{e^{2x}+1}}=-i\tan ix\!}$

• Υπερβολική συνεφαπτομένη

${\displaystyle \coth x={\frac {\cosh x}{\sinh x}}={\frac {\frac {e^{x}+e^{-x}}{2}}{\frac {e^{x}-e^{-x}}{2}}}={\frac {e^{x}+e^{-x}}{e^{x}-e^{-x}}}={\frac {e^{2x}+1}{e^{2x}-1}}=i\cot ix\!}$

• Υπερβολική τέμνουσα

${\displaystyle \operatorname {sech} x={\frac {1}{\cosh x}}={\frac {2}{e^{x}+e^{-x}}}=\sec {ix}\!}$

• υπερβολική συντέμνουσα

${\displaystyle \operatorname {cosech} x={\frac {1}{\sinh x}}={\frac {2}{e^{x}-e^{-x}}}=i\,\csc \,ix\!}$

Όπου ${\displaystyle i}$ είναι η φανταστική μονάδα που ορίζεται ως ${\displaystyle i^{2}=-1}$.

Χρήσιμες σχέσεις

${\displaystyle \sinh(-x)=-\sinh x\,\!}$

${\displaystyle \cosh(-x)=\cosh x\,\!}$

Οπότε:

${\displaystyle \tanh(-x)=-\tanh x\,\!}$

${\displaystyle \coth(-x)=-\coth x\,\!}$

${\displaystyle \operatorname {sech} (-x)=\operatorname {sech} \,x\,\!}$

${\displaystyle \operatorname {cosech} (-x)=-\operatorname {cosech} \,x\,\!}$

Προκύπτει δηλαδή ότι οι cosh x και sech x είναι άρτιες συναρτήσεις, ενώ οι υπόλοιπες είναι περιττές συναρτήσεις.

${\displaystyle \operatorname {sech} ^{-1}x=\cosh ^{-1}\left({\frac {1}{x}}\right)}$

${\displaystyle \operatorname {cosech} ^{-1}x=\sinh ^{-1}\left({\frac {1}{x}}\right)}$

${\displaystyle \coth ^{-1}x=\tanh ^{-1}\left({\frac {1}{x}}\right)}$

Τα υπερβολικά ημίτονα και συνημίτονα ικανοποιούν τη σχέση:

${\displaystyle \cosh ^{2}x-\sinh ^{2}x=1\,}$

η οποία είναι αντίστοιχη της συμβατικής τριγωνομετρικής σχέσης:

${\displaystyle \sin ^{2}x+\cos ^{2}x=1\,}$

Η υπερβολική εφαπτομένη είναι λύση του μη γραμμικού προβλήματος οριακών τιμών.^[2]:

${\displaystyle {\frac {1}{2}}f''=f^{3}-f\qquad$ ;\qquad f(0)=f'(\infty )=0}

Αντίστροφες υπερβολικές εκφρασμένες με λογάριθμους

${\displaystyle \sinh ^{-1}x=\ln \left(x+{\sqrt {x^{2}+1}}\right)}$

${\displaystyle \cosh ^{-1}x=\ln \left(x+{\sqrt {x^{2}-1}}\right);x\geq 1}$

${\displaystyle \tanh ^{-1}x={\frac {1}{2}}\ln \left({\frac {1+x}{1-x}}\right);\left|x\right|<1}$

${\displaystyle \operatorname {sech} ^{-1}x=\ln \left({\frac {1+{\sqrt {1-x^{2}}}}{x}}\right);0<x\leq 1}$

${\displaystyle \operatorname {cosech} ^{-1}x=\ln \left({\frac {1}{x}}+{\frac {\sqrt {1+x^{2}}}{\left|x\right|}}\right)}$

${\displaystyle \coth ^{-1}x={\frac {1}{2}}\ln \left({\frac {x+1}{x-1}}\right);\left|x\right|>1}$

Παράγωγοι

${\displaystyle {\frac {d}{dx}}\sinh(x)=\cosh(x)\,}$

${\displaystyle {\frac {d}{dx}}\cosh(x)=\sinh(x)\,}$

${\displaystyle {\frac {d}{dx}}\tanh(x)=1-\tanh ^{2}(x)={\hbox{sech}}^{2}(x)=1/\cosh ^{2}(x)\,}$

${\displaystyle {\frac {d}{dx}}\coth(x)=1-\coth ^{2}(x)=-{\hbox{csch}}^{2}(x)=-1/\sinh ^{2}(x)\,}$

${\displaystyle {\frac {d}{dx}}\ {\hbox{csch(x)}}=-\coth(x)\ {\hbox{csch(x)}}\,}$

${\displaystyle {\frac {d}{dx}}\ {\hbox{sech(x)}}=-\tanh(x)\ {\hbox{sech(x)}}\,}$

${\displaystyle {\frac {d}{dx}}\left(\sinh ^{-1}x\right)={\frac {1}{\sqrt {1+x^{2}}}}}$

${\displaystyle {\frac {d}{dx}}\left(\cosh ^{-1}x\right)={\frac {1}{\sqrt {x^{2}-1}}}}$

${\displaystyle {\frac {d}{dx}}\left(\tanh ^{-1}x\right)={\frac {1}{1-x^{2}}}}$

${\displaystyle {\frac {d}{dx}}\left(\operatorname {csch} ^{-1}x\right)=-{\frac {1}{\left|x\right|{\sqrt {1+x^{2}}}}}}$

${\displaystyle {\frac {d}{dx}}\left(\operatorname {sech} ^{-1}x\right)=-{\frac {1}{x{\sqrt {1-x^{2}}}}}}$

${\displaystyle {\frac {d}{dx}}\left(\coth ^{-1}x\right)={\frac {1}{1-x^{2}}}}$

Συνήθη ολοκληρώματα

${\displaystyle \int \sinh ax\,dx={\frac {1}{a}}\cosh ax+C}$

${\displaystyle \int \cosh ax\,dx={\frac {1}{a}}\sinh ax+C}$

${\displaystyle \int \tanh ax\,dx={\frac {1}{a}}\ln(\cosh ax)+C}$

${\displaystyle \int \coth ax\,dx={\frac {1}{a}}\ln(\sinh ax)+C}$

${\displaystyle \int {\frac {du}{\sqrt {a^{2}+u^{2}}}}=\sinh ^{-1}\left({\frac {u}{a}}\right)+C}$

${\displaystyle \int {\frac {du}{\sqrt {u^{2}-a^{2}}}}=\cosh ^{-1}\left({\frac {u}{a}}\right)+C}$

${\displaystyle \int {\frac {du}{a^{2}-u^{2}}}={\frac {1}{a}}\tanh ^{-1}\left({\frac {u}{a}}\right)+C;u^{2}<a^{2}}$

${\displaystyle \int {\frac {du}{a^{2}-u^{2}}}={\frac {1}{a}}\coth ^{-1}\left({\frac {u}{a}}\right)+C;u^{2}>a^{2}}$

${\displaystyle \int {\frac {du}{u{\sqrt {a^{2}-u^{2}}}}}=-{\frac {1}{a}}\operatorname {sech} ^{-1}\left({\frac {u}{a}}\right)+C}$

${\displaystyle \int {\frac {du}{u{\sqrt {a^{2}+u^{2}}}}}=-{\frac {1}{a}}\operatorname {csch} ^{-1}\left|{\frac {u}{a}}\right|+C}$

Στις πιο πάνω σχέσεις, C καλούμε την σταθερά ολοκλήρωσης.

Σχέσεις με σειρά Τέιλορ

Είναι δυνατόν να εκφράσουμε τις υπερβολικές συναρτήσεις με χρήση σειράς Taylor:

${\displaystyle \sinh x=x+{\frac {x^{3}}{3!}}+{\frac {x^{5}}{5!}}+{\frac {x^{7}}{7!}}+\cdots =\sum _{n=0}^{\infty }{\frac {x^{2n+1}}{(2n+1)!}}}$

${\displaystyle \cosh x=1+{\frac {x^{2}}{2!}}+{\frac {x^{4}}{4!}}+{\frac {x^{6}}{6!}}+\cdots =\sum _{n=0}^{\infty }{\frac {x^{2n}}{(2n)!}}}$

${\displaystyle \tanh x=x-{\frac {x^{3}}{3}}+{\frac {2x^{5}}{15}}-{\frac {17x^{7}}{315}}+\cdots =\sum _{n=1}^{\infty }{\frac {2^{2n}(2^{2n}-1)B_{2n}x^{2n-1}}{(2n)!}},\left|x\right|<{\frac {\pi }{2}}}$

${\displaystyle \coth x={\frac {1}{x}}+{\frac {x}{3}}-{\frac {x^{3}}{45}}+{\frac {2x^{5}}{945}}+\cdots ={\frac {1}{x}}+\sum _{n=1}^{\infty }{\frac {2^{2n}B_{2n}x^{2n-1}}{(2n)!}},0<\left|x\right|<\pi }$ (Σειρά Laurent)

${\displaystyle \operatorname {sech} \,x=1-{\frac {x^{2}}{2}}+{\frac {5x^{4}}{24}}-{\frac {61x^{6}}{720}}+\cdots =\sum _{n=0}^{\infty }{\frac {E_{2n}x^{2n}}{(2n)!}},\left|x\right|<{\frac {\pi }{2}}}$

${\displaystyle \operatorname {csch} \,x={\frac {1}{x}}-{\frac {x}{6}}+{\frac {7x^{3}}{360}}-{\frac {31x^{5}}{15120}}+\cdots ={\frac {1}{x}}+\sum _{n=1}^{\infty }{\frac {2(1-2^{2n-1})B_{2n}x^{2n-1}}{(2n)!}},0<\left|x\right|<\pi }$ (Σειρά Laurent)

όπου

${\displaystyle B_{n}\,}$ είναι ο νιοστός αριθμός Μπερνούλι

${\displaystyle E_{n}\,}$ είναι ο νιοστός αριθμός Όιλερ